From Wikipedia, the free encyclopedia
In field theory, a branch of mathematics, the minimal polynomial of an element α of a field extension is, roughly speaking, the polynomial of lowest degree having coefficients in the field, such that α is a root of the polynomial. If the minimal polynomial of α exists, it is unique. The coefficient of the highest-degree term in the polynomial is required to be 1.
More formally, a minimal polynomial is defined relative to a field extension E/F and an element of the extension field E/F. The minimal polynomial of an element, if it exists, is a member of F[x], the ring of polynomials in the variable x with coefficients in F. Given an element α of E, let Jα be the set of all polynomials f(x) in F[x] such that f(α) = 0. The element α is called a root or zero of each polynomial in Jα
More specifically, Jα is the kernel of the ring homomorphism from F[x] to E which sends polynomials g to their value g(α) at the element α. Because it is the kernel of a ring homomorphism, Jα is an ideal of the polynomial ring F[x]: it is closed under polynomial addition and subtraction (hence containing the zero polynomial), as well as under multiplication by elements of F (which is scalar multiplication if F[x] is regarded as a vector space over F).
The zero polynomial, all of whose coefficients are 0, is in every Jα since 0αi = 0 for all α and i. This makes the zero polynomial useless for classifying different values of α into types, so it is excepted. If there are any non-zero polynomials in Jα, i.e. if the latter is not the zero ideal, then α is called an algebraic element over F, and there exists a monic polynomial of least degree in Jα. This is the minimal polynomial of α with respect to E/F. It is unique and irreducible over F. If the zero polynomial is the only member of Jα, then α is called a transcendental element over F and has no minimal polynomial with respect to E/F.
Minimal polynomials are useful for constructing and analyzing field extensions. When α is algebraic with minimal polynomial f(x), the smallest field that contains both F and α is isomorphic to the quotient ring F[x]/⟨f(x)⟩, where ⟨f(x)⟩ is the ideal of F[x] generated by f(x). Minimal polynomials are also used to define conjugate elements.
Definition[edit]
Let E/F be a field extension, α an element of E, and F[x] the ring of polynomials in x over F. The element α has a minimal polynomial when α is algebraic over F, that is, when f(α) = 0 for some non-zero polynomial f(x) in F[x]. Then the minimal polynomial of α is defined as the monic polynomial of least degree among all polynomials in F[x] having α as a root.
Properties[edit]
Throughout this section, let E/F be a field extension over F as above, let α ∈ E be an algebraic element over F and let Jα be the ideal of polynomials vanishing on α.
Uniqueness[edit]
The minimal polynomial f of α is unique.
To prove this, suppose that f and g are monic polynomials in Jα of minimal degree n > 0. We have that r := f−g ∈ Jα (because the latter is closed under addition/subtraction) and that m := deg(r) < n (because the polynomials are monic of the same degree). If r is not zero, then r / cm (writing cm ∈ F for the non-zero coefficient of highest degree in r) is a monic polynomial of degree m < n such that r / cm ∈ Jα (because the latter is closed under multiplication/division by non-zero elements of F), which contradicts our original assumption of minimality for n. We conclude that 0 = r = f − g, i.e. that f = g.
Irreducibility[edit]
The minimal polynomial f of α is irreducible, i.e. it cannot be factorized as f = gh for two polynomials g and h of strictly lower degree.
To prove this, first observe that any factorization f = gh implies that either g(α) = 0 or h(α) = 0, because f(α) = 0 and F is a field (hence also an integral domain). Choosing both g and h to be of degree strictly lower than f would then contradict the minimality requirement on f, so f must be irreducible.
Minimal polynomial generates Jα[edit]
The minimal polynomial f of α generates the ideal Jα, i.e. every g in Jα can be factorized as g=fh for some h’ in F[x].
To prove this, it suffices to observe that F[x] is a principal ideal domain, because F is a field: this means that every ideal I in F[x], Jα amongst them, is generated by a single element f. With the exception of the zero ideal I = {0}, the generator f must be non-zero and it must be the unique polynomial of minimal degree, up to a factor in F (because the degree of fg is strictly larger than that of f whenever g is of degree greater than zero). In particular, there is a unique monic generator f, and all generators must be irreducible. When I is chosen to be Jα, for α algebraic over F, then the monic generator f is the minimal polynomial of α.
Examples[edit]
Minimal polynomial of a Galois field extension[edit]
Given a Galois field extension 


if 








Quadratic field extensions[edit]
Q(√2)[edit]
If F = Q, E = R, α = √2, then the minimal polynomial for α is a(x) = x2 − 2. The base field F is important as it determines the possibilities for the coefficients of a(x). For instance, if we take F = R, then the minimal polynomial for α = √2 is a(x) = x − √2.
Q(√d)[edit]
In general, for the quadratic extension given by a square-free 

in particular, this implies 


Biquadratic field extensions[edit]
If α = √2 + √3, then the minimal polynomial in Q[x] is a(x) = x4 − 10x2 + 1 = (x − √2 − √3)(x + √2 − √3)(x − √2 + √3)(x + √2 + √3).
Notice if 



Roots of unity[edit]
The minimal polynomials in Q[x] of roots of unity are the cyclotomic polynomials.
Swinnerton-Dyer polynomials[edit]
The minimal polynomial in Q[x] of the sum of the square roots of the first n prime numbers is constructed analogously, and is called a Swinnerton-Dyer polynomial.
See also[edit]
- Ring of integers
- Algebraic number field
- Minimal polynomials of
References[edit]
- Weisstein, Eric W. «Algebraic Number Minimal Polynomial». MathWorld.
- Minimal polynomial at PlanetMath.
- Pinter, Charles C. A Book of Abstract Algebra. Dover Books on Mathematics Series. Dover Publications, 2010, p. 270–273. ISBN 978-0-486-47417-5
Возвращаясь
к многочленам f(x)
= x4+x+1
и f*(x)
= x4+x3+1,
отмечаем , что всё сказанное относительно
двойственных многочленов справедливо
для этих многочленов.
1.
Корни f(x)
– α1,
α2,
α4,
α8
и корни f*(x)
– α7,
α11,
α13,
α14
являются элементами поля GF(24).
Справедливо:
α1
α14
= α15
= 1; α2
α13
= α15
= 1; α4
α11
= α15
= 1;α8
α7
= α15 =
1.
2.
f(x)
и f*(x)
– неприводимые многочлены, при этом
f7(x)
= x4f1(1/x)
= x4(1+
.
3.f1(x)
и f7(x)
принадлежат одному показателю 15, т.к.
не являются делителями никакого двучлена
меньшей степени.
Проверить
этот факт можно непосредственным
делением х5+1,
х6+1
и т.д. на многочлены f1(x)
и f7(x).
Найдем двучлен минимальной степени,
делителем которого является f1(x)f7(x)
= f17(x)
=
=
(x4+x+1)(x4+x3+1)
= x8+x7+x5+x4+x3+x+1.
Воспользуемся
приемом [4], который эффективнее, чем
последовательное деление х9+1,
х10+1
и т.д. на f17(x).
Будем
искать одночлен хn
остаток от деления которого на f17(x)
равен 1.
Остаток
от деления х8
на f17(x):
X8
= x7+x5+x4+x3+x+1
(mod f17(x));
X9
= x8+x6+x5+x4+x2+x
=
=
x7+x5+x4+x3+x+1+x6+x5+x4+x2+x
=
=
x7+x6+x3+x2+1
(mod f17(x));
X10
= x8+x7+x4+x3+x
=
=
x7+x5+x4+x3+x+1+x7+x4+x3+x
=
=
x5+1
(mod f17(x));
X11
= x6+x
(mod f17(x));
X12
= x7+x2
(mod f17(x));
X13
= x8+x3
= x7+x5+x4+x3+x+1+x3
=
=
x7+x5+x4+x+1
(mod f17(x));
X14
= x8+x6+x5+x2+x
=
=
x7+x5+x4+x3+x+1+x6+x5+x2+x
=
=
x7+x6+x4+x3+x2+1
(mod f17(x));
X15
= x8+x7+x5+x4+x3+x
=
=x7+x5+x4+x3+x+1+x7+x5+x4+x3+x
= 1(mod f17(x)).
Итак
х15+1
– двучлен минимальной степени сомножителем
которого является (х4+х+1)(х4+х3+1).
2.2.Минимальные
многочлены и их свойства
Выше
было показано, что между корнями хq-x
и элементами GF(q),
где q
= pm
существует однозначное соответствие,
заключающееся в том, что каждый элемент
β из GF(q)
является корнем хq-х.
При
этом коэффициенты многочлена хq-x
и его неприводимых сомножителей являются
элементами поля GF(q).Элемент
β, являясь корнем
хq-х,
является корнем одного из его сомножителей.
|
Минимальным |
Пример2.2.1.
Для элементов поля GF(24)
минимальными многочленами являются:
Элемент Минимальный
многочлен
-
х,
-
х+1,
α х4+х+1,
α-1
= α14 х4+х3+1,
α3 х4+х3+х2+х+1,
α5 х2+х+1.
Процесс
нахождения минимальных многочленов
будет обобщен в §2.4.
2.3. Свойства минимальных многочленов над полем gf(p)
1.
|
Минимальный |
Действительно,
если m(x)
= m1(x)m2(x),
то m(β)
= m1(β)m2(β)
= 0, так что либо m1(β)
= 0, либо m2(β)
= 0, что противоречит определению.
2.
|
Если |
Из
этого
вытекает:
3.
|
Минимальный |
Из
этого следует, что
4.
|
Степени |
С
учетом сказанного:
5.
|
Если |
6.
Как
найти m(x)
минимальный многочлен для β = αi
из GF(pm)
?
Если
i
лежит в циклотомическом классе CS(Pm-1)
, то
|
|
Из
свойства 3 непосредственно следует
7.
|
|
где
s
пробегает всё множество представителей
циклотомических классов по модулю pm-1.
Полученный
результат конкретизирует свойство 5
§2.1.
Пример
2.3.1. В
соответствии с данными Примера 2.2.1
произведение всех минимальных многочленов
для элементов поля GF(24)
равно:
х(х+1)(х4+х+1)(х4+х3+1)(х4+х3+х2+х+1)(х2+х+1)
= х16+х,
откуда (х+1)(х4+х+1)(х4+х3+1)(х4+х3+х2+х+1)(х2+х+1)
= х15+1.
Соседние файлы в предмете [НЕСОРТИРОВАННОЕ]
- #
- #
- #
- #
- #
- #
- #
- #
- #
- #
- #
Сообщения без ответов | Активные темы | Избранное
Правила форума
В этом разделе нельзя создавать новые темы.
Если Вы хотите задать новый вопрос, то не дописывайте
его в существующую тему, а создайте новую в корневом разделе «Помогите решить/разобраться (М)».
Если Вы зададите новый вопрос в существующей теме, то в случае нарушения оформления или других правил форума Ваше сообщение и все ответы на него могут быть удалены без предупреждения.
Не ищите на этом форуме халяву
, правила запрещают участникам публиковать готовые решения стандартных учебных задач. Автор вопроса обязан привести свои попытки решения
и указать конкретные затруднения.
Обязательно просмотрите тему
Правила данного раздела, иначе Ваша тема может быть удалена
или перемещена в Карантин, а Вы так и не узнаете, почему.
|
|
минимальный многочлен элемента поля GF(q)
|
|
04/06/10 |
Имеем конечное поле GF(16), q=2, m=4. Поле построено по примитивному многочлену
|
|
|
|
|
Sonic86 |
Re: минимальный многочлен элемента поля GF(q)
|
||
08/04/08 |
Так, Вам нужна книжка Лидл Нидеррайтер Конечные поля. Большая, но несложная.
|
||
|
|
|||
|
Leox |
Re: минимальный многочлен элемента поля GF(q)
|
|
25/08/05 |
Имеем конечное поле GF(16), q=2, m=4. Поле построено по примитивному многочлену минимальный многочлен елемента
|
|
|
|
|
endo |
Re: минимальный многочлен элемента поля GF(q)
|
|
04/06/10 |
откуда это такая формула сразу нарисовалась? пойду читать Лидла.
|
|
|
|
|
Leox |
Re: минимальный многочлен элемента поля GF(q)
|
|
25/08/05 |
откуда это такая формула сразу нарисовалась? пойду читать Лидла. в Лидла, том.2, стр. 605
|
|
|
|
|
Sonic86 |
Re: минимальный многочлен элемента поля GF(q)
|
||
08/04/08 |
|||
|
|
|||
|
Leox |
Re: минимальный многочлен элемента поля GF(q)
|
|
25/08/05 |
Выглядит очень правдоподобно
|
|
|
|
|
endo |
Re: минимальный многочлен элемента поля GF(q)
|
|
04/06/10 |
|
|
|
|
|
Leox |
Re: минимальный многочлен элемента поля GF(q)
|
|
25/08/05 |
вопрос то в чем? формула для минимального многочлена у вас есть, теперь расскрывайте скобки, учитывая реализацию поля
|
|
|
|
|
endo |
Re: минимальный многочлен элемента поля GF(q)
|
|
04/06/10 |
|
|
|
|
Модераторы: Модераторы Математики, Супермодераторы
Кто сейчас на конференции |
|
Сейчас этот форум просматривают: нет зарегистрированных пользователей |
| Вы не можете начинать темы Вы не можете отвечать на сообщения Вы не можете редактировать свои сообщения Вы не можете удалять свои сообщения Вы не можете добавлять вложения |
Пусть $%x=sqrt{q}+sqrt[4]p$%. Тогда $%(x-sqrt{q})^2=sqrt{p}$%, то есть $%x$% будет корнем уравнения $%x^2+q-sqrt{p}=2xsqrt{q}$%. После возведения в квадрат ещё раз, получится $%x^4+2(q-sqrt{p})x^2+q^2+p-2qsqrt{p}=4x^2q$%, и возникает уравнение 4-й степени $%x^4-2(q+sqrt{p})x^2+q^2+p-2qsqrt{p}=0$%.
Этим описывается общая ситуация. Данный многочлен в отдельных случаях может не быть минимальным. Однако в условии про значения $%p$% и $%q$% не сказано даже то, что они рациональны, поэтому анализировать все случаи представляется нецелесообразным. Ясно, например, что при $%p=q=1$% минимальный многочлен будет иметь степень 1. Если $%q$% является квадратом рационального числа, то $%x$% выражается как корень квадратного уравнения, и так далее. Но раз само условие дано без уточнений, то и в решении детально ничего уточнять не будем.




 . Как найти минимальный многочлен для каждого элемента поля GF(16), построенного как расширение поля GF(2)?
. Как найти минимальный многочлен для каждого элемента поля GF(16), построенного как расширение поля GF(2)? (это потому что поле, и у него мультипликативная группа конечная и циклична). Можно разложить на множители над полем и отсюда попытаться, что-то найти, но честно говоря не уверен — думайте пока.
(это потому что поле, и у него мультипликативная группа конечная и циклична). Можно разложить на множители над полем и отсюда попытаться, что-то найти, но честно говоря не уверен — думайте пока.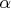 равен
равен 