Показатель преломления — это безразмерная физическая величина, характеризующая отличие фазовых скоростей света в двух средах.
Более подробно о показателе преломления и о том, как его рассчитать, вы узнаете из данной статьи.
Простое объяснение.
Наблюдайте за ходом светового луча из одной среды, например воздуха, в другую среду, например воду. Это можно сделать, например, глядя снизу на поверхность воды над собой при нырянии в бассейне. Если вы это сделаете, то увидите изменение направления луча при переходе из одной среды в другую. Это изменение направления также называется преломлением света. Вы всегда можете наблюдать это в средах с различными показателями преломления.
Показатель преломления — это свойство оптического материала. Это отношение длины волны света в вакууме c0 к длине волны света в среде cM, то есть n = c0 / cM .
Показатель преломления является безразмерным числом и зависит от частоты света. Поскольку показатель преломления зависит от частоты волны (света), мы также говорим о дисперсии. Если две среды имеют разные показатели преломления, вы наблюдаете преломление и отражение света на их границах. Среда с более высоким показателем преломления имеет более высокую оптическую плотность.
Другими терминами для обозначения показателя преломления являются также индекс преломления или оптическая плотность.
Закон преломления Снеллиуса
Закон преломления Снеллиуса гласит, что луч света преломляется, когда попадает в среду с другой оптической плотностью. Причиной преломления является изменение зависящей от материала фазовой скорости, которая входит в закон преломления как показатель преломления. Закон преломления — это зависимость между углом падения θ1 и углом отражения θ2 преломленного света.
n1 * sin θ1 = n2 * sin θ2
В этой формуле n1 и n2 означают показатели преломления двух сред.
Вещества с показателем преломления
Оптическая плотность вакуума определяется как 1. В видимом спектре показатели преломления прозрачных или слабо поглощающих материалов больше 1. Для электропроводящих и сильно поглощающих сред преобладают другие физические свойства. Хотя их показатели преломления находятся между 0 и 1, эти значения следует интерпретировать по-разному. В этих средах в комплексном показателе преломления преобладает мнимая часть.
Кроме того, каждое вещество имеет диапазон длин волн, в котором действительная часть показателя преломления меньше 1, но все еще положительна. Здесь оптическая плотность для малых длин волн всегда меньше 1 и приближается к 1 снизу по мере уменьшения длины волны.
Показатель преломления воздуха
Значение показателя преломления воздуха можно найти в таблице 1 ниже. Он зависит от плотности и температуры, а также от состава воздуха. В частности, влажность воздуха оказывает большое влияние на его коэффициент преломления. Согласно формуле барометрической высоты, давление воздуха экспоненциально уменьшается на больших высотах. На высоте 8 километров коэффициент преломления воздуха составляет всего 1,00011.
Показатель преломления воды
Для показателя преломления воды действуют те же принципы, что и для воздуха. На больших глубинах давление и температура выше, что влияет на преломление света. Но вы также можете легко убедиться в этом, наполнив стакан холодной воды горячей. Вы увидите, что горячая вода менее прозрачна, чем холодная. Поэтому оптическая плотность выше при использовании более горячей воды.
Таблица показателей преломления
В следующей таблице представлен обзор некоторых наиболее важных показателей преломления.
| Среда | Показатель преломления |
| Воздух | 1,000292 |
| Вода (жидкость, 20°C) | 1,3330 |
| Стекло | 1.45 — 2.14 |
| Этанол | 1,3614 |
Комплексный показатель преломления
Если вы посмотрите на электромагнитную волну и рассмотрите ее поглощение в среде, то обнаружите, что можно также объединить классический показатель преломления и затухание волны в комплексный показатель преломления. Для этого существуют различные, эквивалентные представления:
- Сумма действительной части с мнимой частью комплексного числа: n = nr + i * ni , где i — мнимая единица
- Разница между действительной и мнимой частями комплексного числа: n = nr — i*k
- Произведение действительного показателя преломления на комплексное число: n = n * ( 1 — i * k).
Знак минус, используемый в некоторых представлениях, гарантирует, что мнимая часть получит положительный знак в случае поглощающих сред. Эта мнимая часть называется коэффициентом молярной экстинкции. Переменная κ называется показателем поглощения. Это мнимая часть, деленная на показатель преломления n.
Как действительная, так и мнимая части оптической плотности зависят от частоты.
Диэлектрическая проницаемость и проницаемость
Комплексный показатель преломления связан с проницаемостью εr (способность к поляризации) и проницаемостью μr (способность к намагничиванию): n = εr * μr .
Все величины являются комплекснозначными и зависят от частоты. В случае немагнитных сред, μr ≈ 1. Таким образом, вы формируете комплексный показатель преломления непосредственно из действительной и мнимой частей ( ε1, ε2 ) проницаемости.
n ≈ εr = ε1 + i * ε2
Сравнение с комплексным показателем преломления представления суммы и разности позволяет вычислить n и k, соответственно.
Атомы с показателем преломления
Показатель преломления кристаллических веществ напрямую зависит от их атомной структуры. Кристаллическая решетка твердого тела влияет на его полосовую структуру и, следовательно, на его преломляющее поведение.
Частично кристаллические материалы также демонстрируют корреляцию между плотностью и оптической плотностью. Однако эта зависимость, как правило, не является линейной.
Применение показателя преломления
Показатель преломления является наиболее важным параметром для оптических линз. Оптический расчет, используемый для проектирования оптических приборов, основан на сочетании различных преломляющих линз с подходящими стеклами.
В химии и фармации различные вещества характеризуются оптической плотностью при определенных температурах. Кроме того, определяя коэффициент преломления, вы узнаете содержание определенного вещества в растворе.
Список использованной литературы
- Тихомирова С. А., Яворский Б. М. Физика (базовый уровень) – М.: Мнемозина, 2012.
- Генденштейн Л. Э., Дик Ю. И. Физика 10 класс. – М.: Мнемозина, 2014.
- Савельев, И. В. Электричество и магнетизм. Волны. Оптика. // Курс общей физики: Учеб. пособие.. — М.: «Наука», 1988. — Т. 2. — 496 с.
Преломление света.
-
Закон преломления (частный случай).
-
Обратимость световых лучей.
-
Закон преломления (общий случай).
-
Полное внутреннее отражение.
-
Разберем задачи ЕГЭ по теме: Преломление света.
Автор — профессиональный репетитор, автор учебных пособий для подготовки к ЕГЭ Игорь Вячеславович Яковлев
Темы кодификатора ЕГЭ: закон преломления света, полное внутреннее отражение.
На границе раздела двух прозрачных сред наряду с отражением света наблюдается его преломление — свет, переходя в другую среду, меняет направление своего распространения.
Преломление светового луча происходит при его наклонном падении на поверхность раздела (правда, не всегда — читайте дальше про полное внутреннее отражение). Если же луч падает перпендикулярно поверхности, то преломления не будет — во второй среде луч сохранит своё направление и также пойдёт перпендикулярно поверхности.
к оглавлению ▴
Закон преломления (частный случай).
Мы начнём с частного случая, когда одна из сред является воздухом. Именно такая ситуация присутствует в подавляющем большинстве задач. Мы обсудим соответствующий частный случай закона преломления, а уж затем дадим самую общую его формулировку.
Предположим, что луч света, идущий в воздухе, наклонно падает на поверхность стекла, воды или какой-либо другой прозрачной среды. При переходе в среду луч преломляется, и его дальнейший ход показан на рис. 1.
 |
| Рис. 1. Преломление луча на границе «воздух–среда» |
В точке падения проведён перпендикуляр (или, как ещё говорят, нормаль)
к поверхности среды. Луч
, как и раньше, называется падающим лучом, а угол
между падающим лучом и нормалью — углом падения. Луч
— это преломлённый луч; угол
между преломлённым лучом и нормалью к поверхности называется углом преломления.
Всякая прозрачная среда характеризуется величиной , которая называется показателем преломления этой среды. Показатели преломления различных сред можно найти в таблицах. Например, для стекла
, а для воды
. Вообще, у любой среды
; показатель преломления равен единице только в вакууме. У воздуха
, поэтому для воздуха с достаточной точностью можно полагать в задачах
(в оптике воздух не сильно отличается от вакуума).
Закон преломления (переход «воздух–среда»).
1) Падающий луч, преломлённый луч и нормаль к поверхности, проведённая в точке падения, лежат в одной плоскости.
2) Отношение синуса угла падения к синусу угла преломления равно показателю преломления среды:
. (1)
Поскольку из соотношения (1) следует, что
, то есть
— угол преломления меньше угла падения. Запоминаем: переходя из воздуха в среду, луч после преломления идёт ближе к нормали.
Показатель преломления непосредственно связан со скоростью распространения света в данной среде. Эта скорость всегда меньше скорости света в вакууме:
. И вот оказывается,что
. (2)
Почему так получается, мы с вами поймём при изучении волновой оптики. А пока скомбинируем формулы . (1) и (2):
. (3)
Так как показатель преломления воздуха очень близок единице, мы можем считать, что скорость света в воздухе примерно равна скорости света в вакууме . Приняв это во внимание и глядя на формулу . (3), делаем вывод: отношение синуса угла падения к синусу угла преломления равно отношению скорости света в воздухе к скорости света в среде.
к оглавлению ▴
Обратимость световых лучей.
Теперь рассмотрим обратный ход луча: его преломление при переходе из среды в воздух. Здесь нам окажет помощь следующий полезный принцип.
Принцип обратимости световых лучей. Траектория луча не зависит от того, в прямом или обратном направлении распространяется луч. Двигаясь в обратном направлении, луч пойдёт в точности по тому же пути, что и в прямом направлении.
Согласно принципу обратимости, при переходе из среды в воздух луч пойдёт по той же самой траектории, что и при соответствующем переходе из воздуха в среду (рис. 2) Единственное отличие рис. 2 от рис. 1 состоит в том, что направление луча поменялось на противоположное.
 |
| Рис. 2. Преломление луча на границе «среда–воздух» |
Раз геометрическая картинка не изменилась, той же самой останется и формула (1): отношение синуса угла к синусу угла
по-прежнему равно показателю преломления среды. Правда, теперь углы поменялись ролями: угол
стал углом падения, а угол
— углом преломления.
В любом случае, как бы ни шёл луч — из воздуха в среду или из среды в воздух — работает следующее простое правило. Берём два угла — угол падения и угол преломления; отношение синуса большего угла к синусу меньшего угла равно показателю преломления среды.
Теперь мы целиком подготовлены для того, чтобы обсудить закон преломления в самом общем случае.
к оглавлению ▴
Закон преломления (общий случай).
Пусть свет переходит из среды 1 с показателем преломления в среду 2 с показателем преломления
. Среда с большим показателем преломления называется оптически более плотной; соответственно, среда с меньшим показателем преломления называется оптически менее плотной.
Переходя из оптически менее плотной среды в оптически более плотную, световой луч после преломления идёт ближе к нормали (рис. 3). В этом случае угол падения больше угла преломления: .
Наоборот, переходя из оптически более плотной среды в оптически менее плотную, луч отклоняется дальше от нормали (рис. 4). Здесь угол падения меньше угла преломления:
Оказывается, оба этих случая охватываются одной формулой — общим законом преломления, справедливым для любых двух прозрачных сред.
Закон преломления.
1) Падающий луч, преломлённый луч и нормаль к поверхности раздела сред, проведённая в точке падения, лежат в одной плоскости.
2) Отношение синуса угла падения к синусу угла преломления равно отношению показателя преломления второй среды к показателю преломления первой среды:
. (4)
Нетрудно видеть, что сформулированный ранее закон преломления для перехода «воздух–среда» является частным случаем данного закона. В самом деле, полагая в формуле (4) , мы придём к формуле (1).
Вспомним теперь, что показатель преломления — это отношение скорости света в вакууме к скорости света в данной среде: . Подставляя это в (4), получим:
. (5)
Формула (5) естественным образом обобщает формулу (3). Отношение синуса угла падения к синусу угла преломления равно отношению скорости света в первой среде к скорости света во второй среде.
к оглавлению ▴
Полное внутреннее отражение.
При переходе световых лучей из оптически более плотной среды в оптически менее плотную наблюдается интересное явление — полное внутреннее отражение. Давайте разберёмся, что это такое.
Будем считать для определённости, что свет идёт из воды в воздух. Предположим, что в глубине водоёма находится точечный источник света , испускающий лучи во все стороны. Мы рассмотрим некоторые из этих лучей (рис. 5).
 |
| Рис. 5. Полное внутреннее отражение |
Луч падает на поверхность воды под наименьшим углом. Этот луч частично преломляется (луч
) и частично отражается назад в воду (луч
). Таким образом, часть энергии падающего луча передаётся преломлённому лучу, а оставшаяся часть энергии -отражённому лучу.
Угол падения луча больше. Этот луч также разделяется на два луча — преломлённый и отражённый. Но энергия исходного луча распределяется между ними по-другому: преломлённый луч
будет тусклее, чем луч
(то есть получит меньшую долю энергии), а отражённый луч
— соответственно ярче, чем луч
(он получит большую долю энергии).
По мере увеличения угла падения прослеживается та же закономерность: всё большая доля энергии падающего луча достаётся отражённому лучу, и всё меньшая — преломлённому лучу. Преломлённый луч становится всё тусклее и тусклее, и в какой-то момент исчезает совсем!
Это исчезновение происходит при достижении угла падения , которому отвечает угол преломления
. В данной ситуации преломлённый луч
должен был бы пойти параллельно поверхности воды, да идти уже нечему — вся энергия падающего луча
целиком досталась отражённому лучу
.
При дальнейшем увеличении угла падения преломлённый луч и подавно будет отсутствовать.
Описанное явление и есть полное внутреннее отражение. Вода не выпускает наружу лучи с углами падения, равными или превышающими некоторое значение — все такие лучи целиком отражаются назад в воду. Угол
называется предельным углом полного отражения.
Величину легко найти из закона преломления. Имеем:
.
Но , поэтому
,
откуда
.
Так, для воды предельный угол полного отражения равен:
.
Явление полного внутреннего отражения вы легко можете наблюдать дома. Налейте воду в стакан, поднимите его и смотрите на поверхность воды чуть снизу сквозь стенку стакана. Вы увидите серебристый блеск поверхности — вследствие полного внутреннего отражения она ведёт себя подобно зеркалу.
Важнейшим техническим применением полного внутреннего отражения является волоконная оптика. Световые лучи, запущенные внутрь оптоволоконного кабеля (световода) почти параллельно его оси, падают на поверхность под большими углами и целиком, без потери энергии отражаются назад внутрь кабеля. Многократно отражаясь, лучи идут всё дальше и дальше, перенося энергию на значительное расстояние. Волоконно-оптическая связь применяется, например, в сетях кабельного телевидения и высокоскоростного доступа в Интернет.
к оглавлению ▴
Разберем задачи ЕГЭ по теме: Преломление света.
Задача 1. Нижняя грань AC прозрачного клина посеребрена и представляет собой плоское зеркало. Угол при вершине клина . Луч света падает из воздуха на клин перпендикулярно грани AB, преломляется и выходит в воздух через ту же грань AB, но уэе под углом преломления
Определите показатель преломления материала клина. Сделайте рисунок, поясняющий ход луча в клине.
Дано:
n-?
Решение. Решение задач по геометрической оптике необходимо начинать с построения чертежа (рисунка), моделирующего условия, описанные в тексте задачи.
Световой луч падает на прозрачный клин перпендикулярно стороне АВ (см.рис.1). В этом случае, световой луч не преломляется на границе раздела воздух-клин, так как угол падения равен 0, соответственно, угол преломления также равен 0. Следовательно, внутри клина световой луч попадает на нижнюю грань АС, которая представляет собой плоское зеркало. Согласно рис.1 величина угла
Тогда угол падения луча на плоское зеркало будет равен
То есть угол падения равен .
Согласно закону отражения света, угол падения светового луча равен углу отражения. В треугольнике МКО угол КОМ образован суммой двух углов α, поэтому он равен 60°. Тогда угол падения светового луча на грань АВ также будет равен (равенство накрест лежащих углов).
На следующем этапе задачи надо применить закон преломления света, так как луч переходит из одной среды в другую.
При записи этой формулы учтено, что второй средой является воздух с показателем преломления равным 1, а первой средой является материал клина с показателем преломления n, который необходимо определить. Из последней формулы можно выразить и рассчитать n.
Ответ: 1,15
Задача 2. На тонкую собирающую линзу от удалённого источника падает пучок параллельных лучей (см. рисунок). Как изменится положение изображения источника, создаваемого линзой, если между линзой и её фокусом поставить
плоскопараллельную стеклянную пластинку с показателем преломления n (на рисунке положение пластинки отмечено пунктиром)? Ответ поясните, указав, какие физические закономерности Вы использовали. Сделайте рисунок, поясняющий ход лучей до и после установки плоскопараллельной стеклянной пластинки.
Решение. Рассмотрим ход световых лучей от удаленного источника через линзу при отсутствии плоскопараллельной стеклянной пластинки (см.рис.1).
Луч 1-1ʹ проходит через оптический центр линзы и не преломляется. Луч 2-2ʹ идет через фокус и после прохождения через линзу, идет параллельно главной оптической оси. Пересечение этих двух лучей дает действительное изображение удаленного источника, которое расположено в фокальной плоскости линзы. Этот факт также можно доказать, используя формулу тонкой линзы.
Так как источник света расположен на расстоянии то
Тогда формула тонкой линзы (1) примет вид следовательно, f=F, т.е. изображение формируется в фокальной плоскости линзы.
Рассмотрим ход световых лучей через плоскопараллельную стеклянную пластинку. Для этого необходимо использовать закон преломления света.
Рис.2
Согласно рис.2 угол падения луча на пластину равен α. Закон преломления света на границе раздела воздух-пластинка имеет вид:
Здесь учтено, что показатель преломления воздуха равен 1, а пластинки n.
При переходе светового луча из пластинки в воздух, закон преломления света будет иметь вид:
В этом случае первой средой является пластинка с показателем преломления n, а второй средой будет воздух с показателем преломления равным 1.
Из (1) и (2) выразим и
.
и
Так как правые части этих уравнений равны, то
Отсюда вытекает равенство углов . Следовательно, луч, падающий на стеклянную пластину, выходит из нее, оставаясь параллельным входящему лучу. Но при этом выходящий луч немного смещается вверх.
Исходя из этого можно сделать вывод, что изображение удаленного источника после прохождения через плоскопараллельную стеклянную пластину, не изменится. Из удаленного источника выходит бесконечное количество параллельных лучей, которые собираются в фокальной плоскости линзы.
Ответ: не изменится.
Задача 3. Ученик провел опыт по преломлению света, представленный на фотографии. Как изменится при уменьшении угла падения угол преломления светового пучка и скорость света, распространяющегося в стекле? Для каждой величины определите соответствующий характер изменения:
1) увеличится
2) уменьшиться
3) не изменится
Запишите в таблицу выбранные цифры для каждой величины. Цифры в ответе могут повторяться.
| Угол преломления | Скорость света в стекле |
Решение. Для ответа на первый вопрос задачи необходимо применить закон преломления света для границы раздела воздух-стекло.
Показатель преломления стекла равен n, а воздуха 1.
При уменьшении угла падения α, будет уменьшаться и значение Так как показатель преломления стекла не изменяется, то значение
так же будет уменьшаться. Поэтому угол преломления уменьшится.
Для ответа на второй вопрос надо учесть, что скорость света в данной среде определяется значением показателя преломления где с – скорость света в вакууме, а n – показатель преломления среды (стекла). Так как эти обе величины не изменяются, то скорость света в стекле так же не изменяется.
Ответ: 23.
Задача 4. Чему равен синус предельного угла полного внутреннего отражения при переходе света из вещества с в вещество с
?
Решение.
Явление полного внутреннего отражения наблюдается при переходе светового луча из оптически более плотной среды в оптически менее плотную (см.рис.1). Источник света S должен находиться в среде с большим показателем преломления.
Для нахождения синуса угла полного внутреннего отражения необходимо воспользоваться законом преломления света.
При полном внутреннем отражении преломленный луч скользит по границе раздела двух сред и угол преломления . С учетом того, что
уравнение (1) примет вид:
Ответ: 0,8.
Если вам нравятся наши материалы — записывайтесь на курсы подготовки к ЕГЭ по физике онлайн
Благодарим за то, что пользуйтесь нашими публикациями.
Информация на странице «Преломление света.» подготовлена нашими редакторами специально, чтобы помочь вам в освоении предмета и подготовке к ЕГЭ и ОГЭ.
Чтобы успешно сдать необходимые и поступить в ВУЗ или техникум нужно использовать все инструменты: учеба, контрольные, олимпиады, онлайн-лекции, видеоуроки, сборники заданий.
Также вы можете воспользоваться другими статьями из данного раздела.
Публикация обновлена:
08.05.2023
Определение показателя преломления воздуха
Цель
работы:
определить
показатель преломления воздуха с помощью
интерферометра.
Оборудование:
интерферометр,
помпа, манометр.
ТЕОРЕТИЧЕСКОЕ
ВВЕДЕНИЕ
Интерферометр
– это измерительный прибор, в котором
используется явление интерференции
волн. Интерферометры применяются для
измерения длин волн, расстояний,
показателей преломления прозрачных
сред, для контроля качества обработки
поверхности.
Интерференция
– это явление сложения когерентных
волн, в результате которого в пространстве
возникают области усиления и ослабления
колебаний, происходит перераспределение
энергии из области минимумов в область
максимумов. Волны являются когерентными,
если частоты колебаний одинаковы,
разность фаз в точке наблюдения постоянна;
для световых волн, которые являются
поперечными, плоскости колебаний
световых векторов должны быть параллельны.
Волны
максимально усиливают друг друга, если
направления световых векторов совпадают,
разность фаз кратна 2π радиан: ∆φ=2π
κ, где κ
= 1, 2, 3…– целое число. Волны ослабляют
друг друга, если направление световых
векторов противоположно, разность фаз
кратна нечетному числу π радиан: ∆φ
= (2
κ+1)
π. Пусть
интерферируют две гармонические волны:
Е1=
Е1,0
cos
(ωt—2π
l1
/ λı),
(1)
Е2
= Е2,0
cos
(ωt—2π
l2
/
λ2). (2)
Здесь
Е
– световой вектор (вектор напряженности
электрического поля волны), ω
– циклическая
частота колебаний, λ
– длина
волны, l
– расстояние
от источников до точки наблюдения.
Разность фаз в точке наблюдения будет
равна
∆φ
=2π
(lı
/ λı—
l2
/
λ2
).
(3)
Для
удобства решения задач интерференции
считают, что обе волны распространяются
не в разных средах, а в вакууме с одинаковой
скоростью света, с одинаковой длиной
волны λ. Но зато увеличивают длину пути
так, чтобы время распространения не
изменилось. Это воображаемое расстояние
называют оптическим путем. Длины волн
в средах связаны с длиной волны в вакууме:
λı=
λ /n1
,
λ2=
λ /n2
,
где n1
и n2
– абсолютные показатели преломления
сред. Тогда разность фаз в формуле (3)
будет равна
.
Произведение геометрического пути на
показатель преломления l∙n
и есть оптический путь. Условия усиления
и ослабления колебаний через разность
оптических путей примут вид
max:
ΔL= l1n1
—
l2
n2
= k λ , (4)
min:
ΔL= l1n1–l2n2
=
(2
k+1)
λ
/2. (5)
Р
ассмотрим
в качестве примера интерференцию
от двух
когерентных источников
света в виде
параллельных, близко расположенных
узких щелей. Расстояние между щелями d
много меньше
расстояния от щелей экрана l
(рис. 1).
На
экране наблюдается интерференционная
картина в виде чередующихся темных и
светлых полос. В центре экрана центральный
максимум, к
= 0. Он образован волнами (пунктир) с
одинаковым оптическим путем. Следующий
максимум к
= 1-го порядка
будет при оптической разности путей в
одну длину волны, второй – в две длины
волны и так далее.
Определим
расстояние Yк
от центра до максимума к-порядка.
Запишем приближенно подобие треугольников
(рис. 1): Yк
/ l
=∆L
/ d.
Откуда расстояние до максимума к-порядка,
с учетом условия (4) ΔL=kλ,
будет
. (6)
Из
этой формулы следует, что наблюдать
интерференционную картину на экране,
удаленном от щелей на несколько метров,
можно, если расстояние между щелями
составляет доли миллиметра. Это
осуществляется в специальных приборах
– интерферометрах.
Н
апример,
интерферометр Жамена
(рис. 2). Свет от лампы, пройдя через
конденсор, падает на толстую стеклянную
пластину. Лучи 1 и 2, отраженные от нижней
пластины, распространяются как бы из
бесконечности слабо расходящимися
пучками. Они проходят через кюветы и
при отражении от второй пластины
собираются вместе. Интерференционная
картина наблюдается через окуляр.
П
ри
идентичных пластинах разность оптических
путей будет зависеть от показателей
преломления среды в кюветах n1
и n2
и длины кювет l:
∆L=
l
(n1–n2).
Показатель
преломления воздуха зависит от
концентрации примесей, например метана.
Поэтому интерферометр используют как
шахтный, для определения концентрации
метана. Еще показатель преломления
газов зависит от давления.
Лабораторная
установка
для измерения
показателя преломления воздуха состоит
из шахтного интерферометра, помпы для
изменения давления и манометра для
измерения давления (рис. 3).
При
повышении давления в одной из кювет,
появляется оптическая разность хода
между лучами. Смещение интерференционной
картины на к
полос соответствует изменению разности
оптических путей лучей на ∆L
= к λ.
Разность оптических путей зависит от
длины кювет l
и разности показателей преломления
воздуха в кюветах: ∆L
= 2
∆n
l.
Число 2 обусловлено 2-кратным прохождением
лучей через кюветы, так как для уменьшения
габаритов в шахтном интерферометре
установлена оборотная призма. Итак,
изменение показателя преломления
воздуха можно определить по числу
смещенных интерференционных полос:
. (7)
ВЫПОЛНЕНИЕ РАБОТЫ
1. Установить
винтом помпы относительное давление,
равное нулю. Включить в сеть 220 В
трансформатор осветителя интерферометра.
Наблюдать интерференционную картину
через окуляр интерферометра. Компенсатором
установить какую-нибудь темную
интерференционную полосу против нуля
шкалы (или у края окна).
2. Увеличивая
давление в одной из кювет поворотом
винта помпы, наблюдать смещение
интерференционной картины сначала на
одну полосу. Измерить избыточное давление
по шкале манометра.
Увеличивая
давление, опыт повторить не менее 5 раз
при смещении на 2, 3 и т. д. полос
интерференции. Записать в таблицу
результаты измерений. Выключить
осветитель, вывернуть винт помпы.
|
Длина |
0,67 |
||||||
|
Длина |
50 |
||||||
|
Число |
0 |
1 |
2 |
3 |
4 |
5 |
6 |
|
Давление |
0 |
3. Построить
график зависимости повышения давления
от числа смещенных полос интерференции
(рис. 4).
4. Определить
изменение показателя преломления
воздуха Δn
в последнем опыте при наибольшем числе
смещенных полос (к
= 6) по формуле (7). Определить по графику
соответствующее повышение давления ΔР
(точка А).
5. Определить
среднее значение показателя преломления
воздуха при атмосферном давлении, равном
Pатм
= 740 мм
рт. ст. Будем считать, что при повышении
давления от нуля до атмосферного
показатель преломления воздуха
увеличился от единицы до искомого
значения <n>
в такой же пропорции, как в проведенных
опытах. Составим пропорцию
∆n/∆P=(<n>–1)/Pатм.
Откуда
. (8)
6
.
Оценить случайную погрешность измерения
показателя преломления воздуха по
формуле
, (9)
где
m
– число измерений, σР
– диапазон
отклонения экспериментальных точек от
средней линии на рис. 4.
7.
Записать результат работы n
= <n>
± δn,
Р = 90
%. Сделать выводы.
КОНТРОЛЬНЫЕ
ВОПРОСЫ
1. Дайте
определение явления интерференции,
понятие когерентности волн.
2. Запишите
условие разности фаз волн при усилении
или ослабления колебаний.
3. Объясните
введение оптического пути. Запишите
условие образования интерференционных
максимума и минимума через разность
оптических путей волн.
4. Выведите
формулу для координаты интерференционной
полосы при двулучевой интерференции.
5. Объясните
принцип действия интерферометра Жамена.
Для чего нужна вторая стеклянная
пластинка? От чего зависит разность
оптических путей двух интерферирующих
волн?
6. Выведите
формулу для расчета показателя преломления
воздуха при атмосферном давлении.
Работа 40
Соседние файлы в предмете [НЕСОРТИРОВАННОЕ]
- #
- #
- #
- #
- #
- #
- #
- #
- #
- #
- #
In optics, the refractive index (or refraction index) of an optical medium is a dimensionless number that gives the indication of the light bending ability of that medium.
Refraction of a light ray
The refractive index determines how much the path of light is bent, or refracted, when entering a material. This is described by Snell’s law of refraction, n1 sin θ1 = n2 sin θ2, where θ1 and θ2 are the angle of incidence and angle of refraction, respectively, of a ray crossing the interface between two media with refractive indices n1 and n2. The refractive indices also determine the amount of light that is reflected when reaching the interface, as well as the critical angle for total internal reflection, their intensity (Fresnel’s equations) and Brewster’s angle.[1]
The refractive index can be seen as the factor by which the speed and the wavelength of the radiation are reduced with respect to their vacuum values: the speed of light in a medium is v = c/n, and similarly the wavelength in that medium is λ = λ0/n, where λ0 is the wavelength of that light in vacuum. This implies that vacuum has a refractive index of 1, and assumes that the frequency (f = v/λ) of the wave is not affected by the refractive index.
The refractive index may vary with wavelength. This causes white light to split into constituent colors when refracted. This is called dispersion. This effect can be observed in prisms and rainbows, and as chromatic aberration in lenses. Light propagation in absorbing materials can be described using a complex-valued refractive index.[2] The imaginary part then handles the attenuation, while the real part accounts for refraction. For most materials the refractive index changes with wavelength by several percent across the visible spectrum. Nevertheless, refractive indices for materials are commonly reported using a single value for n, typically measured at 633 nm.
The concept of refractive index applies across the full electromagnetic spectrum, from X-rays to radio waves. It can also be applied to wave phenomena such as sound. In this case, the speed of sound is used instead of that of light, and a reference medium other than vacuum must be chosen.[3]
For lenses (such as eye glasses), a lens made from a high refractive index material will be thinner, and hence lighter, than a conventional lens with a lower refractive index. Such lenses are generally more expensive to manufacture than conventional ones.
Definition[edit]
The relative refractive index of an optical medium 2 with respect to another reference medium 1 (n21) is given by the ratio of speed of light in medium 1 to that in medium 2. This can be expressed as follows:
If the reference medium 1 is vacuum, then the refractive index of medium 2 is considered with respect to vacuum. It is simply represented as n2 and is called the absolute refractive index of medium 2.
The absolute refractive index n of an optical medium is defined as the ratio of the speed of light in vacuum, c = 299792458 m/s, and the phase velocity v of light in the medium,
Since c is constant, n is inversely proportional to v :
The phase velocity is the speed at which the crests or the phase of the wave moves, which may be different from the group velocity, the speed at which the pulse of light or the envelope of the wave moves.[1] Historically air at a standardized pressure and temperature has been common as a reference medium.
History[edit]
Thomas Young was presumably the person who first used, and invented, the name «index of refraction», in 1807.[4]
At the same time he changed this value of refractive power into a single number, instead of the traditional ratio of two numbers. The ratio had the disadvantage of different appearances. Newton, who called it the «proportion of the sines of incidence and refraction», wrote it as a ratio of two numbers, like «529 to 396» (or «nearly 4 to 3»; for water).[5] Hauksbee, who called it the «ratio of refraction», wrote it as a ratio with a fixed numerator, like «10000 to 7451.9» (for urine).[6] Hutton wrote it as a ratio with a fixed denominator, like 1.3358 to 1 (water).[7]
Young did not use a symbol for the index of refraction, in 1807. In the later years, others started using different symbols:
n, m, and µ.[8][9][10] The symbol n gradually prevailed.
Typical values[edit]
Diamonds have a very high refractive index of 2.417.
Refractive index also varies with wavelength of the light as given by Cauchy’s equation:
The most general form of Cauchy’s equation is
where n is the refractive index, λ is the wavelength, A, B, C, etc., are coefficients that can be determined for a material by fitting the equation to measured refractive indices at known wavelengths. The coefficients are usually quoted for λ as the vacuum wavelength in micrometres.
Usually, it is sufficient to use a two-term form of the equation:
where the coefficients A and B are determined specifically for this form of the equation.
| Material | n |
|---|---|
| Vacuum | 1 |
| Gases at 0 °C and 1 atm | |
| Air | 1.000293 |
| Helium | 1.000036 |
| Hydrogen | 1.000132 |
| Carbon dioxide | 1.00045 |
| Liquids at 20 °C | |
| Water | 1.333 |
| Ethanol | 1.36 |
| Olive oil | 1.47 |
| Solids | |
| Ice | 1.31 |
| Fused silica (quartz) | 1.46[11] |
| PMMA (acrylic, plexiglas, lucite, perspex) | 1.49 |
| Window glass | 1.52[12] |
| Polycarbonate (Lexan™) | 1.58[13] |
| Flint glass (typical) | 1.69 |
| Sapphire | 1.77[14] |
| Cubic zirconia | 2.15 |
| Diamond | 2.417 |
| Moissanite | 2.65 |
For visible light most transparent media have refractive indices between 1 and 2. A few examples are given in the adjacent table. These values are measured at the yellow doublet D-line of sodium, with a wavelength of 589 nanometers, as is conventionally done.[15] Gases at atmospheric pressure have refractive indices close to 1 because of their low density. Almost all solids and liquids have refractive indices above 1.3, with aerogel as the clear exception. Aerogel is a very low density solid that can be produced with refractive index in the range from 1.002 to 1.265.[16] Moissanite lies at the other end of the range with a refractive index as high as 2.65. Most plastics have refractive indices in the range from 1.3 to 1.7, but some high-refractive-index polymers can have values as high as 1.76.[17]
For infrared light refractive indices can be considerably higher. Germanium is transparent in the wavelength region from 2 to 14 µm and has a refractive index of about 4.[18] A type of new materials termed «topological insulators», was recently found which have high refractive index of up to 6 in the near to mid infrared frequency range. Moreover, topological insulators are transparent when they have nanoscale thickness. These properties are potentially important for applications in infrared optics.[19]
Refractive index below unity[edit]
According to the theory of relativity, no information can travel faster than the speed of light in vacuum, but this does not mean that the refractive index cannot be less than 1. The refractive index measures the phase velocity of light, which does not carry information.[20] The phase velocity is the speed at which the crests of the wave move and can be faster than the speed of light in vacuum, and thereby give a refractive index below 1. This can occur close to resonance frequencies, for absorbing media, in plasmas, and for X-rays. In the X-ray regime the refractive indices are lower than but very close to 1 (exceptions close to some resonance frequencies).[21]
As an example, water has a refractive index of 0.99999974 = 1 − 2.6×10−7 for X-ray radiation at a photon energy of 30 keV (0.04 nm wavelength).[21]
An example of a plasma with an index of refraction less than unity is Earth’s ionosphere. Since the refractive index of the ionosphere (a plasma), is less than unity, electromagnetic waves propagating through the plasma are bent «away from the normal» (see Geometric optics) allowing the radio wave to be refracted back toward earth, thus enabling long-distance radio communications. See also Radio Propagation and Skywave.[22]
Negative refractive index[edit]
Recent research has also demonstrated the existence of materials with a negative refractive index, which can occur if permittivity and permeability have simultaneous negative values.[23] This can be achieved with periodically constructed metamaterials. The resulting negative refraction (i.e., a reversal of Snell’s law) offers the possibility of the superlens and other new phenomena to be actively developed by means of metamaterials.[24][25]
Microscopic explanation[edit]
At the atomic scale, an electromagnetic wave’s phase velocity is slowed in a material because the electric field creates a disturbance in the charges of each atom (primarily the electrons) proportional to the electric susceptibility of the medium. (Similarly, the magnetic field creates a disturbance proportional to the magnetic susceptibility.) As the electromagnetic fields oscillate in the wave, the charges in the material will be «shaken» back and forth at the same frequency.[1]: 67 The charges thus radiate their own electromagnetic wave that is at the same frequency, but usually with a phase delay, as the charges may move out of phase with the force driving them (see sinusoidally driven harmonic oscillator). The light wave traveling in the medium is the macroscopic superposition (sum) of all such contributions in the material: the original wave plus the waves radiated by all the moving charges. This wave is typically a wave with the same frequency but shorter wavelength than the original, leading to a slowing of the wave’s phase velocity. Most of the radiation from oscillating material charges will modify the incoming wave, changing its velocity. However, some net energy will be radiated in other directions or even at other frequencies (see scattering).
Depending on the relative phase of the original driving wave and the waves radiated by the charge motion, there are several possibilities:
- If the electrons emit a light wave which is 90° out of phase with the light wave shaking them, it will cause the total light wave to travel slower. This is the normal refraction of transparent materials like glass or water, and corresponds to a refractive index which is real and greater than 1.[26]
- If the electrons emit a light wave which is 270° out of phase with the light wave shaking them, it will cause the wave to travel faster. This is called «anomalous refraction», and is observed close to absorption lines (typically in infrared spectra), with X-rays in ordinary materials, and with radio waves in Earth’s ionosphere. It corresponds to a permittivity less than 1, which causes the refractive index to be also less than unity and the phase velocity of light greater than the speed of light in vacuum c (note that the signal velocity is still less than c, as discussed above). If the response is sufficiently strong and out-of-phase, the result is a negative value of permittivity and imaginary index of refraction, as observed in metals or plasma.[26]
- If the electrons emit a light wave which is 180° out of phase with the light wave shaking them, it will destructively interfere with the original light to reduce the total light intensity. This is light absorption in opaque materials and corresponds to an imaginary refractive index.
- If the electrons emit a light wave which is in phase with the light wave shaking them, it will amplify the light wave. This is rare, but occurs in lasers due to stimulated emission. It corresponds to an imaginary index of refraction, with the opposite sign to that of absorption.
For most materials at visible-light frequencies, the phase is somewhere between 90° and 180°, corresponding to a combination of both refraction and absorption.
Dispersion[edit]
Light of different colors has slightly different refractive indices in water and therefore shows up at different positions in the rainbow.
In a prism, dispersion causes different colors to refract at different angles, splitting white light into a rainbow of colors.
The variation of refractive index with wavelength for various glasses. The shaded zone indicates the range of visible light.
The refractive index of materials varies with the wavelength (and frequency) of light.[27] This is called dispersion and causes prisms and rainbows to divide white light into its constituent spectral colors.[28] As the refractive index varies with wavelength, so will the refraction angle as light goes from one material to another. Dispersion also causes the focal length of lenses to be wavelength dependent. This is a type of chromatic aberration, which often needs to be corrected for in imaging systems. In regions of the spectrum where the material does not absorb light, the refractive index tends to decrease with increasing wavelength, and thus increase with frequency. This is called «normal dispersion», in contrast to «anomalous dispersion», where the refractive index increases with wavelength.[27] For visible light normal dispersion means that the refractive index is higher for blue light than for red.
For optics in the visual range, the amount of dispersion of a lens material is often quantified by the Abbe number:[28]
For a more accurate description of the wavelength dependence of the refractive index, the Sellmeier equation can be used.[29] It is an empirical formula that works well in describing dispersion. Sellmeier coefficients are often quoted instead of the refractive index in tables.
Principal refractive index wavelength ambiguity[edit]
Because of dispersion, it is usually important to specify the vacuum wavelength of light for which a refractive index is measured. Typically, measurements are done at various well-defined spectral emission lines.
Manufacturers of optical glass in general define principal index of refraction at yellow spectral line of helium (587.56 nm) and alternatively at a green spectral line of mercury (546.07 nm), called d and e lines respectively. Abbe number is defined for both and denoted Vd and Ve. The spectral data provided by glass manufacturers is also often more precise for these 2 wavelengths.[30][31][32][33]
Both, d and e spectral lines are singlets and thus are suitable to perform a very precise measurements, such as spectral goniometric method.[34][35]
In practical applications, measurements of refractive index are performed on various refractometers, such as Abbe refractometer. Measurement accuracy of such typical commercial devices is in the order of 0.0002.[36][37] Refractometers usually measure refractive index nD, defined for sodium doublet D (589.29 nm), which is actually a midpoint between 2 adjacent yellow spectral lines of sodium. Yellow spectral lines of helium (d) and sodium (D) are 1.73 nm apart, which can be considered negligible for typical refractometers, but can cause confusion and lead to errors if accuracy is critical.
All 3 typical principle refractive indices definitions can be found depending on application and region,[38] so a proper subscript should be used to avoid ambiguity.
Complex refractive index[edit]
When light passes through a medium, some part of it will always be absorbed. This can be conveniently taken into account by defining a complex refractive index,
Here, the real part n is the refractive index and indicates the phase velocity, while the imaginary part κ is called the optical extinction coefficient or absorption coefficient—although κ can also refer to the mass attenuation coefficient[39]: 3 —and indicates the amount of attenuation when the electromagnetic wave propagates through the material.[1]: 128
That κ corresponds to absorption can be seen by inserting this refractive index into the expression for electric field of a plane electromagnetic wave traveling in the x-direction. This can be done by relating the complex wave number k to the complex refractive index n through k = 2πn/λ0, with λ0 being the vacuum wavelength; this can be inserted into the plane wave expression for a wave travelling in the x direction as:
Here we see that κ gives an exponential decay, as expected from the Beer–Lambert law. Since intensity is proportional to the square of the electric field, intensity will depend on the depth into the material as
and thus the absorption coefficient is α = 4πκ/λ0,[1]: 128 and the penetration depth (the distance after which the intensity is reduced by a factor of 1/e) is δp = 1/α = λ0/4πκ.
Both n and κ are dependent on the frequency. In most circumstances κ > 0 (light is absorbed) or κ = 0 (light travels forever without loss). In special situations, especially in the gain medium of lasers, it is also possible that κ < 0, corresponding to an amplification of the light.
An alternative convention uses n = n + iκ instead of n = n − iκ, but where κ > 0 still corresponds to loss. Therefore, these two conventions are inconsistent and should not be confused. The difference is related to defining sinusoidal time dependence as Re[exp(−iωt)] versus Re[exp(+iωt)]. See Mathematical descriptions of opacity.
Dielectric loss and non-zero DC conductivity in materials cause absorption. Good dielectric materials such as glass have extremely low DC conductivity, and at low frequencies the dielectric loss is also negligible, resulting in almost no absorption. However, at higher frequencies (such as visible light), dielectric loss may increase absorption significantly, reducing the material’s transparency to these frequencies.
The real, n, and imaginary, κ, parts of the complex refractive index are related through the Kramers–Kronig relations. In 1986 A.R. Forouhi and I. Bloomer deduced an equation describing κ as a function of photon energy, E, applicable to amorphous materials. Forouhi and Bloomer then applied the Kramers–Kronig relation to derive the corresponding equation for n as a function of E. The same formalism was applied to crystalline materials by Forouhi and Bloomer in 1988.
The refractive index and extinction coefficient, n and κ, are typically measured from quantities that depend on them, such as reflectance, R, or transmittance, T, or ellipsometric parameters, ψ and δ. The determination of n and κ from such measured quantities will involve developing a theoretical expression for R or T, or ψ and δ in terms of a valid physical model for n and κ. By fitting the theoretical model to the measured R or T, or ψ and δ using regression analysis, n and κ can be deduced.
X-ray and extreme UV[edit]
For X-ray and extreme ultraviolet radiation the complex refractive index deviates only slightly from unity and usually has a real part smaller than 1. It is therefore normally written as n = 1 − δ + iβ (or n = 1 − δ − iβ with the alternative convention mentioned above).[2] Far above the atomic resonance frequency delta can be given by
where 



with 

Relations to other quantities[edit]
Optical path length[edit]
Optical path length (OPL) is the product of the geometric length d of the path light follows through a system, and the index of refraction of the medium through which it propagates,[40]
This is an important concept in optics because it determines the phase of the light and governs interference and diffraction of light as it propagates. According to Fermat’s principle, light rays can be characterized as those curves that optimize the optical path length.[1]: 68–69
Refraction[edit]
Refraction of light at the interface between two media of different refractive indices, with n2 > n1. Since the phase velocity is lower in the second medium (v2 < v1), the angle of refraction θ2 is less than the angle of incidence θ1; that is, the ray in the higher-index medium is closer to the normal.
When light moves from one medium to another, it changes direction, i.e. it is refracted. If it moves from a medium with refractive index n1 to one with refractive index n2, with an incidence angle to the surface normal of θ1, the refraction angle θ2 can be calculated from Snell’s law:[41]
When light enters a material with higher refractive index, the angle of refraction will be smaller than the angle of incidence and the light will be refracted towards the normal of the surface. The higher the refractive index, the closer to the normal direction the light will travel. When passing into a medium with lower refractive index, the light will instead be refracted away from the normal, towards the surface.
Total internal reflection[edit]
If there is no angle θ2 fulfilling Snell’s law, i.e.,
the light cannot be transmitted and will instead undergo total internal reflection.[42]: 49–50 This occurs only when going to a less optically dense material, i.e., one with lower refractive index. To get total internal reflection the angles of incidence θ1 must be larger than the critical angle[43]
Reflectivity[edit]
Apart from the transmitted light there is also a reflected part. The reflection angle is equal to the incidence angle, and the amount of light that is reflected is determined by the reflectivity of the surface. The reflectivity can be calculated from the refractive index and the incidence angle with the Fresnel equations, which for normal incidence reduces to[42]: 44
For common glass in air, n1 = 1 and n2 = 1.5, and thus about 4% of the incident power is reflected.[44] At other incidence angles the reflectivity will also depend on the polarization of the incoming light. At a certain angle called Brewster’s angle, p-polarized light (light with the electric field in the plane of incidence) will be totally transmitted. Brewster’s angle can be calculated from the two refractive indices of the interface as [1]: 245
Lenses[edit]
The focal length of a lens is determined by its refractive index n and the radii of curvature R1 and R2 of its surfaces. The power of a thin lens in air is given by the Lensmaker’s formula:[45]
where f is the focal length of the lens.
Microscope resolution[edit]
The resolution of a good optical microscope is mainly determined by the numerical aperture (NA) of its objective lens. The numerical aperture in turn is determined by the refractive index n of the medium filling the space between the sample and the lens and the half collection angle of light θ according to[46]: 6
For this reason oil immersion is commonly used to obtain high resolution in microscopy. In this technique the objective is dipped into a drop of high refractive index immersion oil on the sample under study.[46]: 14
Relative permittivity and permeability[edit]
The refractive index of electromagnetic radiation equals
where εr is the material’s relative permittivity, and μr is its relative permeability.[47]: 229 The refractive index is used for optics in Fresnel equations and Snell’s law; while the relative permittivity and permeability are used in Maxwell’s equations and electronics. Most naturally occurring materials are non-magnetic at optical frequencies, that is μr is very close to 1,[citation needed] therefore n is approximately √εr. In this particular case, the complex relative permittivity εr, with real and imaginary parts εr and ɛ̃r, and the complex refractive index n, with real and imaginary parts n and κ (the latter called the «extinction coefficient»), follow the relation
and their components are related by:[48]
and:
where 
Wave impedance[edit]
The wave impedance of a plane electromagnetic wave in a non-conductive medium is given by
where 
In non-magnetic media with 
Thus refractive index in a non-magnetic media is the ratio of the vacuum wave impedance to the wave impedance of the medium.
The reflectivity 
Density[edit]
In general, the refractive index of a glass increases with its density. However, there does not exist an overall linear relationship between the refractive index and the density for all silicate and borosilicate glasses. A relatively high refractive index and low density can be obtained with glasses containing light metal oxides such as Li2O and MgO, while the opposite trend is observed with glasses containing PbO and BaO as seen in the diagram at the right.
Many oils (such as olive oil) and ethanol are examples of liquids that are more refractive, but less dense, than water, contrary to the general correlation between density and refractive index.
For air, n − 1 is proportional to the density of the gas as long as the chemical composition does not change.[50] This means that it is also proportional to the pressure and inversely proportional to the temperature for ideal gases.
Group index[edit]
Sometimes, a «group velocity refractive index», usually called the group index is defined:[citation needed]
where vg is the group velocity. This value should not be confused with n, which is always defined with respect to the phase velocity. When the dispersion is small, the group velocity can be linked to the phase velocity by the relation[42]: 22
where λ is the wavelength in the medium. In this case the group index can thus be written in terms of the wavelength dependence of the refractive index as
When the refractive index of a medium is known as a function of the vacuum wavelength (instead of the wavelength in the medium), the corresponding expressions for the group velocity and index are (for all values of dispersion)[51]
where λ0 is the wavelength in vacuum.
Other relations[edit]
As shown in the Fizeau experiment, when light is transmitted through a moving medium, its speed relative to an observer traveling with speed v in the same direction as the light is:
The refractive index of a substance can be related to its polarizability with the Lorentz–Lorenz equation or to the molar refractivities of its constituents by the Gladstone–Dale relation.
Refractivity[edit]
In atmospheric applications, refractivity is defined as N = n – 1, often scaled as either[52] N = 106(n – 1)[53][54] or N = 108(n – 1);[55] the multiplication factors are used because the refractive index for air, n deviates from unity by at most a few parts per ten thousand.
Molar refractivity, on the other hand, is a measure of the total polarizability of a mole of a substance and can be calculated from the refractive index as
where ρ is the density, and M is the molar mass.[42]: 93
Nonscalar, nonlinear, or nonhomogeneous refraction[edit]
So far, we have assumed that refraction is given by linear equations involving a spatially constant, scalar refractive index. These assumptions can break down in different ways, to be described in the following subsections.
Birefringence[edit]
Birefringent materials can give rise to colors when placed between crossed polarizers. This is the basis for photoelasticity.
In some materials, the refractive index depends on the polarization and propagation direction of the light.[56] This is called birefringence or optical anisotropy.
In the simplest form, uniaxial birefringence, there is only one special direction in the material. This axis is known as the optical axis of the material.[1]: 230 Light with linear polarization perpendicular to this axis will experience an ordinary refractive index no while light polarized in parallel will experience an extraordinary refractive index ne.[1]: 236 The birefringence of the material is the difference between these indices of refraction, Δn = ne − no.[1]: 237 Light propagating in the direction of the optical axis will not be affected by the birefringence since the refractive index will be no independent of polarization. For other propagation directions the light will split into two linearly polarized beams. For light traveling perpendicularly to the optical axis the beams will have the same direction.[1]: 233 This can be used to change the polarization direction of linearly polarized light or to convert between linear, circular, and elliptical polarizations with waveplates.[1]: 237
Many crystals are naturally birefringent, but isotropic materials such as plastics and glass can also often be made birefringent by introducing a preferred direction through, e.g., an external force or electric field. This effect is called photoelasticity, and can be used to reveal stresses in structures. The birefringent material is placed between crossed polarizers. A change in birefringence alters the polarization and thereby the fraction of light that is transmitted through the second polarizer.
In the more general case of trirefringent materials described by the field of crystal optics, the dielectric constant is a rank-2 tensor (a 3 by 3 matrix). In this case the propagation of light cannot simply be described by refractive indices except for polarizations along principal axes.
Nonlinearity[edit]
The strong electric field of high intensity light (such as the output of a laser) may cause a medium’s refractive index to vary as the light passes through it, giving rise to nonlinear optics.[1]: 502 If the index varies quadratically with the field (linearly with the intensity), it is called the optical Kerr effect and causes phenomena such as self-focusing and self-phase modulation.[1]: 264 If the index varies linearly with the field (a nontrivial linear coefficient is only possible in materials that do not possess inversion symmetry), it is known as the Pockels effect.[1]: 265
Inhomogeneity[edit]
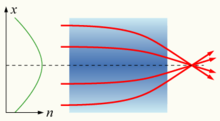
A gradient-index lens with a parabolic variation of refractive index (n) with radial distance (x). The lens focuses light in the same way as a conventional lens.
If the refractive index of a medium is not constant but varies gradually with the position, the material is known as a gradient-index or GRIN medium and is described by gradient index optics.[1]: 273 Light traveling through such a medium can be bent or focused, and this effect can be exploited to produce lenses, some optical fibers, and other devices. Introducing GRIN elements in the design of an optical system can greatly simplify the system, reducing the number of elements by as much as a third while maintaining overall performance.[1]: 276 The crystalline lens of the human eye is an example of a GRIN lens with a refractive index varying from about 1.406 in the inner core to approximately 1.386 at the less dense cortex.[1]: 203 Some common mirages are caused by a spatially varying refractive index of air.
Refractive index measurement[edit]
Homogeneous media[edit]
The principle of many refractometers
The refractive index of liquids or solids can be measured with refractometers. They typically measure some angle of refraction or the critical angle for total internal reflection. The first laboratory refractometers sold commercially were developed by Ernst Abbe in the late 19th century.[57]
The same principles are still used today. In this instrument, a thin layer of the liquid to be measured is placed between two prisms. Light is shone through the liquid at incidence angles all the way up to 90°, i.e., light rays parallel to the surface. The second prism should have an index of refraction higher than that of the liquid, so that light only enters the prism at angles smaller than the critical angle for total reflection. This angle can then be measured either by looking through a telescope,[clarification needed] or with a digital photodetector placed in the focal plane of a lens. The refractive index n of the liquid can then be calculated from the maximum transmission angle θ as n = nG sin θ, where nG is the refractive index of the prism.[58]
A handheld refractometer used to measure the sugar content of fruits
This type of device is commonly used in chemical laboratories for identification of substances and for quality control. Handheld variants are used in agriculture by, e.g., wine makers to determine sugar content in grape juice, and inline process refractometers are used in, e.g., chemical and pharmaceutical industry for process control.
In gemology, a different type of refractometer is used to measure the index of refraction and birefringence of gemstones. The gem is placed on a high refractive index prism and illuminated from below. A high refractive index contact liquid is used to achieve optical contact between the gem and the prism. At small incidence angles most of the light will be transmitted into the gem, but at high angles total internal reflection will occur in the prism. The critical angle is normally measured by looking through a telescope.[59]
Refractive index variations[edit]
A differential interference contrast microscopy image of yeast cells
Unstained biological structures appear mostly transparent under Bright-field microscopy as most cellular structures do not attenuate appreciable quantities of light. Nevertheless, the variation in the materials that constitute these structures also corresponds to a variation in the refractive index. The following techniques convert such variation into measurable amplitude differences:
To measure the spatial variation of the refractive index in a sample phase-contrast imaging methods are used. These methods measure the variations in phase of the light wave exiting the sample. The phase is proportional to the optical path length the light ray has traversed, and thus gives a measure of the integral of the refractive index along the ray path. The phase cannot be measured directly at optical or higher frequencies, and therefore needs to be converted into intensity by interference with a reference beam. In the visual spectrum this is done using Zernike phase-contrast microscopy, differential interference contrast microscopy (DIC), or interferometry.
Zernike phase-contrast microscopy introduces a phase shift to the low spatial frequency components of the image with a phase-shifting annulus in the Fourier plane of the sample, so that high-spatial-frequency parts of the image can interfere with the low-frequency reference beam. In DIC the illumination is split up into two beams that are given different polarizations, are phase shifted differently, and are shifted transversely with slightly different amounts. After the specimen, the two parts are made to interfere, giving an image of the derivative of the optical path length in the direction of the difference in the transverse shift.[46] In interferometry the illumination is split up into two beams by a partially reflective mirror. One of the beams is let through the sample before they are combined to interfere and give a direct image of the phase shifts. If the optical path length variations are more than a wavelength the image will contain fringes.
There exist several phase-contrast X-ray imaging techniques to determine 2D or 3D spatial distribution of refractive index of samples in the X-ray regime.[60]
Applications[edit]
The refractive index is an important property of the components of any optical instrument. It determines the focusing power of lenses, the dispersive power of prisms, the reflectivity of lens coatings, and the light-guiding nature of optical fiber. Since the refractive index is a fundamental physical property of a substance, it is often used to identify a particular substance, confirm its purity, or measure its concentration. The refractive index is used to measure solids, liquids, and gases. Most commonly it is used to measure the concentration of a solute in an aqueous solution. It can also be used as a useful tool to differentiate between different types of gemstone, due to the unique chatoyance each individual stone displays. A refractometer is the instrument used to measure the refractive index. For a solution of sugar, the refractive index can be used to determine the sugar content (see Brix).
See also[edit]
- Fermat’s principle
- Calculation of glass properties
- Clausius–Mossotti relation
- Ellipsometry
- Index-matching material
- Index ellipsoid
- Laser Schlieren Deflectometry
- Optical properties of water and ice
- Prism-coupling refractometry
- Phase-contrast X-ray imaging
- Velocity factor
References[edit]
- ^ a b c d e f g h i j k l m n o p q r Hecht, Eugene (2002). Optics. Addison-Wesley. ISBN 978-0-321-18878-6.
- ^ a b Attwood, David (1999). Soft X-rays and extreme ultraviolet radiation: principles and applications. p. 60. ISBN 978-0-521-02997-1.
- ^ Kinsler, Lawrence E. (2000). Fundamentals of Acoustics. John Wiley. p. 136. ISBN 978-0-471-84789-2.
- ^ Young, Thomas (1807). A course of lectures on natural philosophy and the mechanical arts. J. Johnson. p. 413.
- ^ Newton, Isaac (1730). Opticks: Or, A Treatise of the Reflections, Refractions, Inflections and Colours of Light. William Innys at the West-End of St. Paul’s. p. 247.
- ^ Hauksbee, Francis (1710). «A Description of the Apparatus for Making Experiments on the Refractions of Fluids». Philosophical Transactions of the Royal Society of London. 27 (325–336): 207. doi:10.1098/rstl.1710.0015. S2CID 186208526.
- ^ Hutton, Charles (1795). Philosophical and mathematical dictionary. p. 299. Archived from the original on 2017-02-22.
- ^ von Fraunhofer, Joseph (1817). «Bestimmung des Brechungs und Farbenzerstreuungs Vermogens verschiedener Glasarten». Denkschriften der Königlichen Akademie der Wissenschaften zu München. 5: 208. Archived from the original on 2017-02-22. Exponent des Brechungsverhältnisses is index of refraction
- ^ Brewster, David (1815). «On the structure of doubly refracting crystals». Philosophical Magazine. 45 (202): 126. doi:10.1080/14786441508638398. Archived from the original on 2017-02-22.
- ^ Herschel, John F.W. (1828). On the Theory of Light. p. 368. Archived from the original on 2015-11-24.
- ^ Malitson (1965). «Refractive Index Database». refractiveindex.info. Retrieved June 20, 2018.
- ^ Faick, C.A.; Finn, A.N. (July 1931). «The Index of Refraction of Some Soda-Lime-Silica Glasses as a Function of the Composition» (.pdf). National Institute of Standards and Technology. Archived (PDF) from the original on December 30, 2016. Retrieved 11 December 2016.
- ^ Sultanova, N.; Kasarova, S.; Nikolov, I. (October 2009). «Dispersion Properties of Optical Polymers». Acta Physica Polonica A. 116 (4): 585–587. Bibcode:2009AcPPA.116..585S. doi:10.12693/APhysPolA.116.585.
- ^ Tapping, J.; Reilly, M. L. (1 May 1986). «Index of refraction of sapphire between 24 and 1060°C for wavelengths of 633 and 799 nm». Journal of the Optical Society of America A. 3 (5): 610. Bibcode:1986JOSAA…3..610T. doi:10.1364/JOSAA.3.000610.
- ^ «Forensic Science Communications, Glass Refractive Index Determination». FBI Laboratory Services. Archived from the original on 2014-09-10. Retrieved 2014-09-08.
- ^ Tabata, M.; et al. (2005). «Development of Silica Aerogel with Any Density» (PDF). 2005 IEEE Nuclear Science Symposium Conference Record. 2: 816–818. doi:10.1109/NSSMIC.2005.1596380. ISBN 978-0-7803-9221-2. S2CID 18187536. Archived (PDF) from the original on 2013-05-18.
- ^ Naoki Sadayori and Yuji Hotta «Polycarbodiimide having high index of refraction and production method thereof» US patent 2004/0158021 A1 (2004)
- ^ Tosi, Jeffrey L., article on Common Infrared Optical Materials in the Photonics Handbook, accessed on 2014-09-10
- ^ Yue, Zengji; Cai, Boyuan; Wang, Lan; Wang, Xiaolin; Gu, Min (2016-03-01). «Intrinsically core-shell plasmonic dielectric nanostructures with ultrahigh refractive index». Science Advances. 2 (3): e1501536. Bibcode:2016SciA….2E1536Y. doi:10.1126/sciadv.1501536. ISSN 2375-2548. PMC 4820380. PMID 27051869.
- ^ Als-Nielsen, J.; McMorrow, D. (2011). Elements of Modern X-ray Physics. Wiley-VCH. p. 25. ISBN 978-0-470-97395-0.
One consequence of the real part of n being less than unity is that it implies that the phase velocity inside the material, c/n, is larger than the velocity of light, c. This does not, however, violate the law of relativity, which requires that only signals carrying information do not travel faster than c. Such signals move with the group velocity, not with the phase velocity, and it can be shown that the group velocity is in fact less than c.
- ^ a b «X-Ray Interactions With Matter». The Center for X-Ray Optics. Archived from the original on 2011-08-27. Retrieved 2011-08-30.
- ^ Lied, Finn (1967). High Frequency Radio Communications with Emphasis on Polar Problems. The Advisory Group for Aerospace Research and Development. pp. 1–7.
- ^ Veselago, V. G. (1968). «The electrodynamics of substances with simultaneously negative values of ε and μ». Soviet Physics Uspekhi. 10 (4): 509–514. Bibcode:1968SvPhU..10..509V. doi:10.1070/PU2003v046n07ABEH001614. S2CID 250862458.
- ^ Pendry, J.B; Schurig, D.;Smith D.R.»Electromagnetic compression apparatus, methods and systems», U.S. Patent 7,629,941, Date: Dec. 8, 2009
- ^ Shalaev, V. M. (2007). «Optical negative-index metamaterials». Nature Photonics. 1 (1): 41–48. Bibcode:2007NaPho…1…41S. doi:10.1038/nphoton.2006.49. S2CID 170678.
- ^ a b Feynman, Richard P. (2011). Feynman Lectures on Physics 1: Mainly Mechanics, Radiation, and Heat. Basic Books. ISBN 978-0-465-02493-3.
- ^ a b R. Paschotta, article on chromatic dispersion Archived 2015-06-29 at the Wayback Machine in the Encyclopedia of Laser Physics and Technology Archived 2015-08-13 at the Wayback Machine, accessed on 2014-09-08
- ^ a b Carl R. Nave, page on Dispersion Archived 2014-09-24 at the Wayback Machine in HyperPhysics Archived 2007-10-28 at the Wayback Machine, Department of Physics and Astronomy, Georgia State University, accessed on 2014-09-08
- ^ R. Paschotta, article on Sellmeier formula Archived 2015-03-19 at the Wayback Machine in the Encyclopedia of Laser Physics and Technology Archived 2015-08-13 at the Wayback Machine, accessed on 2014-09-08
- ^ «Search Results | SCHOTT AG». www.schott.com (in Polish). Retrieved 2022-08-15.
- ^ «Optical Properties». www.oharacorp.com. Retrieved 2022-08-15.
- ^ «HOYA GROUP Optics Division | Optical Properties |». www.hoya-opticalworld.com. Retrieved 2022-08-15.
- ^ The Properties of Optical Glass. Schott Series on Glass and Glass Ceramics. 1998. p. 30. doi:10.1007/978-3-642-57769-7. ISBN 978-3-642-63349-2.
- ^ Krey, Stefan; Off, Dennis; Ruprecht, Aiko (2014-03-08). Soskind, Yakov G; Olson, Craig (eds.). «Measuring the refractive index with precision goniometers: a comparative study». Photonic Instrumentation Engineering. SPIE. 8992: 56–65. Bibcode:2014SPIE.8992E..0DK. doi:10.1117/12.2041760. S2CID 120544352.
- ^ Rupp, Fabian; Jedamzik, Ralf; Bartelmess, Lothar; Petzold, Uwe (2021-09-12). Völkel, Reinhard; Geyl, Roland; Otaduy, Deitze (eds.). «The modern way of refractive index measurement of optical glass at SCHOTT». Optical Fabrication, Testing, and Metrology VII. SPIE. 11873: 15–22. Bibcode:2021SPIE11873E..08R. doi:10.1117/12.2597023. ISBN 9781510645905. S2CID 240561530.
- ^ «Abbe Refractometer| ATAGO CO., LTD». www.atago.net. Retrieved 2022-08-15.
- ^ «Abbe Multi-Wavelength Refractometer». Nova-Tech International. Retrieved 2022-08-15.
- ^ The Properties of Optical Glass. Schott Series on Glass and Glass Ceramics. 1998. p. 267. doi:10.1007/978-3-642-57769-7. ISBN 978-3-642-63349-2.
- ^ Dresselhaus, M. S. (1999). «Solid State Physics Part II Optical Properties of Solids» (PDF). Course 6.732 Solid State Physics. MIT. Archived (PDF) from the original on 2015-07-24. Retrieved 2015-01-05.
- ^ R. Paschotta, article on optical thickness Archived 2015-03-22 at the Wayback Machine in the Encyclopedia of Laser Physics and Technology Archived 2015-08-13 at the Wayback Machine, accessed on 2014-09-08
- ^ R. Paschotta, article on refraction Archived 2015-06-28 at the Wayback Machine in the Encyclopedia of Laser Physics and Technology Archived 2015-08-13 at the Wayback Machine, accessed on 2014-09-08
- ^ a b c d Born, Max; Wolf, Emil (1999). Principles of Optics (7th expanded ed.). CUP Archive. p. 22. ISBN 978-0-521-78449-8.
- ^ Paschotta, R. «Total Internal Reflection». RP Photonics Encyclopedia. Archived from the original on 2015-06-28. Retrieved 2015-08-16.
- ^ Swenson, Jim; Incorporates Public Domain material from the U.S. Department of Energy (November 10, 2009). «Refractive Index of Minerals». Newton BBS, Argonne National Laboratory, US DOE. Archived from the original on May 28, 2010. Retrieved 2010-07-28.
- ^ Carl R. Nave, page on the Lens-Maker’s Formula Archived 2014-09-26 at the Wayback Machine in HyperPhysics Archived 2007-10-28 at the Wayback Machine, Department of Physics and Astronomy, Georgia State University, accessed on 2014-09-08
- ^ a b c Carlsson, Kjell (2007). «Light microscopy» (PDF). Archived (PDF) from the original on 2015-04-02. Retrieved 2015-01-02.
- ^ Bleaney, B.; Bleaney, B.I. (1976). Electricity and Magnetism (Third ed.). Oxford University Press. ISBN 978-0-19-851141-0.
- ^ Wooten, Frederick (1972). Optical Properties of Solids. New York City: Academic Press. p. 49. ISBN 978-0-12-763450-0.(online pdf) Archived 2011-10-03 at the Wayback Machine
- ^ «Calculation of the Refractive Index of Glasses». Statistical Calculation and Development of Glass Properties. Archived from the original on 2007-10-15.
- ^ Stone, Jack A.; Zimmerman, Jay H. (2011-12-28). «Index of refraction of air». Engineering metrology toolbox. National Institute of Standards and Technology (NIST). Archived from the original on 2014-01-11. Retrieved 2014-01-11.
- ^ Bor, Z.; Osvay, K.; Rácz, B.; Szabó, G. (1990). «Group refractive index measurement by Michelson interferometer». Optics Communications. 78 (2): 109–112. Bibcode:1990OptCo..78..109B. doi:10.1016/0030-4018(90)90104-2.
- ^ Young, A. T. (2011), Refractivity of Air, archived from the original on 10 January 2015, retrieved 31 July 2014
- ^ Barrell, H.; Sears, J. E. (1939), «The Refraction and Dispersion of Air for the Visible Spectrum», Philosophical Transactions of the Royal Society of London, A, Mathematical and Physical Sciences, 238 (786): 1–64, Bibcode:1939RSPTA.238….1B, doi:10.1098/rsta.1939.0004, JSTOR 91351
- ^ Aparicio, Josep M.; Laroche, Stéphane (2011-06-02). «An evaluation of the expression of the atmospheric refractivity for GPS signals». Journal of Geophysical Research. 116 (D11): D11104. Bibcode:2011JGRD..11611104A. doi:10.1029/2010JD015214.
- ^ Ciddor, P. E. (1996), «Refractive Index of Air: New Equations for the Visible and Near Infrared», Applied Optics, 35 (9): 1566–1573, Bibcode:1996ApOpt..35.1566C, doi:10.1364/ao.35.001566, PMID 21085275
- ^ R. Paschotta, article on birefringence Archived 2015-07-03 at the Wayback Machine in the Encyclopedia of Laser Physics and Technology Archived 2015-08-13 at the Wayback Machine, accessed on 2014-09-09
- ^ «The Evolution of the Abbe Refractometer». Humboldt State University, Richard A. Paselk. 1998. Archived from the original on 2011-06-12. Retrieved 2011-09-03.
- ^ «Refractometers and refractometry». Refractometer.pl. 2011. Archived from the original on 2011-10-20. Retrieved 2011-09-03.
- ^ «Refractometer». The Gemology Project. Archived from the original on 2011-09-10. Retrieved 2011-09-03.
- ^ Fitzgerald, Richard (July 2000). «Phase‐Sensitive X‐Ray Imaging». Physics Today. 53 (7): 23. Bibcode:2000PhT….53g..23F. doi:10.1063/1.1292471. S2CID 121322301.
External links[edit]
Wikimedia Commons has media related to Refraction.
- NIST calculator for determining the refractive index of air
- Dielectric materials
- Science World
- Filmetrics’ online database Free database of refractive index and absorption coefficient information
- RefractiveIndex.INFO Refractive index database featuring online plotting and parameterisation of data
- LUXPOP Thin film and bulk index of refraction and photonics calculations
- The Feynman Lectures on Physics Vol. II Ch. 32: Refractive Index of Dense Materials
| Показатель преломления | |
 |
|
| Размерность |
безразмерная |
|---|---|
| Примечания | |
|
скаляр |
Показа́тель преломле́ния (абсолютный показатель преломления) вещества — величина, равная отношению фазовых скоростей света (электромагнитных волн) в вакууме и в данной среде 
Содержание
- 1 Описание
- 2 Примеры
- 3 Материалы с отрицательным показателем преломления
- 4 См. также
- 5 Примечания
- 6 Литература
- 7 Ссылки
Описание[править | править вики-текст]
Показатель преломления, как абсолютный, так и относительный (см. ниже), равен отношению синуса угла падения к синусу угла преломления (см. Закон преломления света), и зависит от природы (свойств) вещества и длины волны излучения; для некоторых веществ показатель преломления достаточно сильно меняется при изменении частоты электромагнитных волн от низких частот до оптических и далее, а также может ещё более резко меняться в определённых областях частотной шкалы. По умолчанию обычно имеется в виду оптический диапазон или диапазон, определяемый контекстом.
Существуют оптически анизотропные вещества, в которых показатель преломления зависит от направления и поляризации света. Такие вещества достаточно распространены, в частности, это все кристаллы с достаточно низкой симметрией кристаллической решётки, а также вещества, подвергнутые механической деформации.
Показатель преломления можно выразить как корень из произведения магнитной и диэлектрической проницаемостей среды
(надо при этом учитывать, что значения магнитной проницаемости 

В поглощающих средах диэлектрическая проницаемость содержит мнимую компоненту 





Падение и преломление лучей (волн) света
По закону преломления волн преломлённый луч 








где 

Аналогично, для относительного показателя преломления первой среды относительно второй 
Очевидно, что 

Относительный показатель преломления, при прочих равных условиях, обычно меньше единицы при переходе луча из среды более плотной в среду менее плотную, и больше единицы при переходе луча из среды менее плотной в среду более плотную (например, из газа или из вакуума в жидкость или твёрдое тело). Есть исключения из этого правила, и потому среду с относительным показателем преломления, бо́льшим единицы, принято называть оптически более плотной, чем другая (не путать с оптической плотностью как мерой непрозрачности среды).
Луч, падающий из вакуума на поверхность какой-нибудь среды, преломляется сильнее, чем при падении на неё из другой среды; показатель преломления среды, соответствующий лучу, падающему на неё из вакуума, называется абсолютным показателем преломления или просто показателем преломления; это и есть показатель преломления, определение которого дано в начале статьи. Абсолютный показатель преломления любого газа, в том числе воздуха, при обычных условиях мало отличается от единицы, поэтому приближенно (и со сравнительно неплохой точностью) об абсолютном показателе преломления исследуемой среды можно судить по её показателю преломления относительно воздуха.
Для измерения показателя преломления используют ручные и автоматические рефрактометры.
Примеры[править | править вики-текст]
Показатели преломления некоторых сред приведены в таблице.
Показатели преломления для длины волны 589,3 нм
| Тип среды | Среда | Температура, °С | Значение |
|---|---|---|---|
| Кристаллы[2] | LiF | 20 | 1,3920 |
| NaCl | 20 | 1,5442 | |
| KCl | 20 | 1,4870 | |
| KBr | 20 | 1,5552 | |
| Оптические стёкла[3] | ЛК3 (Лёгкий крон) | 20 | 1,4874 |
| К8 (Крон) | 20 | 1,5163 | |
| ТК4 (Тяжёлый крон) | 20 | 1,6111 | |
| СТК9 (Сверхтяжёлый крон) | 20 | 1,7424 | |
| Ф1 (Флинт) | 20 | 1,6128 | |
| ТФ10 (Тяжёлый флинт) | 20 | 1,8060 | |
| СТФ3 (Сверхтяжёлый флинт) | 20 | 2,1862[4] | |
| Драгоценные камни[2] | Алмаз белый | — | 2,417 |
| Берилл | — | 1,571—1,599 | |
| Изумруд | — | 1,588—1,595 | |
| Сапфир белый | — | 1,768—1,771 | |
| Сапфир зелёный | — | 1,770—1,779 | |
| Жидкости[2] | Вода дистиллированная | 20 | 1,3330 |
| Бензол | 20—25 | 1,5014 | |
| Глицерин | 20—25 | 1,4730 | |
| Кислота серная | 20—25 | 1,4290 | |
| Кислота соляная | 20—25 | 1,2540 | |
| Масло анисовое | 20—25 | 1,560 | |
| Масло подсолнечное | 20—25 | 1,470 | |
| Масло оливковое | 20—25 | 1,467 | |
| Спирт этиловый | 20—25 | 1,3612 |
Материалы с отрицательным показателем преломления[править | править вики-текст]
В 1967 году В. Г. Веселаго высказал гипотезу о существовании материалов с отрицательным значением показателя преломления [5]. Существование подобных материалов было практически доказано в 2000 г. Дэвидом Смитом (англ. David R. Smith) из Калифорнийского университета в Сан-Диего и Джоном Пендри из Имперского колледжа в Лондоне [6]. Подобные метаматериалы обладают рядом интересных свойств [7]:
- фазовая и групповая скорости волн имеют различное направление;
- возможно преодоление дифракционного предела при создании оптических систем («суперлинз»), повышение с их помощью разрешающей способности микроскопов, создание микросхем наномасштаба, повышение плотности записи на оптические носители информации.
См. также[править | править вики-текст]
- Преломление
- Закон Снелла
- Метаматериалы
- Метрический тензор
- Иммерсионный метод измерения показателя преломления.
Примечания[править | править вики-текст]
- ↑ Линза акустическая — статья из Физической энциклопедии
- ↑ 1 2 3 Бабичев А. П., Бабушкина Н. А., Братковский А. М. и др. Физические величины/ / Под ред. И. С. Григорьева и Е. З. Мейлихова. — Справочник. — М.: Энергоатомиздат, 1991. — 1232 с. — 50 000 экз. — ISBN 5-283-04013-5.
- ↑ ГОСТ 13659-78. Стекло оптическое бесцветное. Физико-химические характеристики. Основные параметры. — М: Издательство стандартов, 1999. — 27 с.
- ↑ Бесцветное оптическое стекло СССР. Каталог. Под ред. Петровского Г. Т. — М.: Дом оптики, 1990. — 131 с. — 3000 экз.
- ↑ Веселаго В. Г. // УФН. — 1967. — Т. 92. — С. 517.
- ↑ John B. Pendry; David R. Smith. «Reversing Light with Negative Refraction». Physics Today 57 (6).
- ↑ Дж. Пендри, Д. Смит. В поисках суперлинзы. Elementy.ru (2006). Архивировано из первоисточника 22 августа 2011.
Литература[править | править вики-текст]
- Веселаго В.Г. О формулировке принципа Ферма для света, распространяющегося в веществах с отрицательным преломлением // Успехи физических наук, 2002, т. 172, № 10, c. 1215-1218.
- Веселаго В.Г. Электродинамика материалов с отрицательным коэффициентом преломления // Успехи физических наук, 2003, т. 173, № 7, c. 790-794.
- Вашковский А.В., Локк Э.Г. Возникновение отрицательного коэффициента преломления при распространении поверхностной магнитостатической волны через границу раздела сред феррит-феррит-диэлектрик-металл // Успехи физических наук, 2004, т. 174, № 6, c. 657-662.
- Агранович В.М. Отрицательное преломление в оптическом диапазоне и нелинейное распространение волн // Успехи физических наук, 2004, т. 174, № 6, c. 683-684.
- Вашковский А.В., Локк Э.Г. Свойства обратных электромагнитных волн и возникновение отрицательного отражения в ферритовых пленках // Успехи физических наук, 2006, т. 176, № 4, c. 403-414.
- Вашковский А.В., Локк Э.Г. Прямые и обратные неколлинеарные волны в магнитных пленках // Успехи физических наук, 2006, т. 176, № 5, c. 557-562.
- Агранович В.М., Гартштейн Ю.Н. Пространственная дисперсия и отрицательное преломление света // Успехи физических наук, 2006, т. 176, № 10, c. 1051-1068.
- Воронов В. К., Подоплелов А. В. Физика на переломе тысячелетий: конденсированное состояние, 2-е изд., М.: ЛКИ, 2012, 336 стр., ISBN 978-5-382-01365-7
Ссылки[править | править вики-текст]
- Отрицательный показатель преломления
- RefractiveIndex.INFO база данных показателей преломления
- Воздушная линза в воде, видео














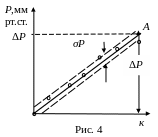












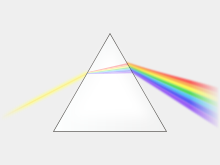
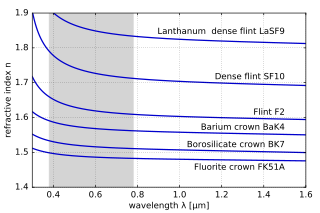


![{displaystyle mathbf {E} (s,t)=operatorname {Re} !left[mathbf {E} _{0}e^{i({underline {k}}x-omega t)}right]=operatorname {Re} !left[mathbf {E} _{0}e^{i(2pi (n+ikappa )x/lambda _{0}-omega t)}right]=e^{-2pi kappa x/lambda _{0}}operatorname {Re} !left[mathbf {E} _{0}e^{i(kx-omega t)}right].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/333b7fbfb29a7c6c8aeff046daf68f0f9e7da430)