Как найти положительное среднее геометрическое - Исправление недочетов и поиск решений вместе с Examum.ru

Загрузить PDF

Среднее геометрическое — математическая величина, которую легко спутать с более часто применяемым средним арифметическим. Для вычисления среднего геометрического следуйте методам, приведенным ниже.

1
Возьмите два числа, среднее геометрическое которых необходимо найти.
- Например, 2 и 32.
2
3

Реклама

1

Подставьте числа в приведенное уравнение. Если это, скажем, 10 и 15, то подставьте их так, как показано на рисунке.
2

Найдите «х». Начните с перемножения крест-накрест, что означает перемножение пар чисел по диагонали и расстановку результатов умножения по разные стороны знака =. Так как х*х = х², то уравнение приводится к виду к виду: х² = (результат умножения ваших чисел). Для вычисления «х» извлеките квадратный корень из результата перемножения используемых чисел. Если в результате вычисления корня получится целое число — отлично. Если нет, дайте ответ в виде десятичной дроби или запишите его со знаком корня (в зависимости от того, что требует преподаватель). Ответ, приведенный выше на рисунке, записан в виде упрощенного квадратного корня.

Реклама

1
Подставьте числа в приведенное уравнение.Среднее геометрическое = (a₁ × a₂ . . . a_n)^1/n^[3]
- a₁ — первое число, a₂ — второе число и так далее
- n — общее количество чисел
2

Перемножьте числа (a₁, a₂ и так далее).
3

Извлеките корень n степени из полученного числа. Это и будет среднее геометрическое.^[4]

Реклама

1
Найдите логарифм каждого числа и сложите полученные значения. Найдите клавишу LOG на своем калькуляторе. Затем введите: (первое число) LOG + (второе число) LOG + (третье число) LOG [+ столько чисел, сколько дано] =. Не забудьте нажать «=», или показанный вам результат будет логарифмом последнего введенного числа, а не суммой логарифмов всех чисел.
- Например, log 7 + log 9 + log 12 = 2,878521796
2
Разделите результат сложения на общее количество изначально данных чисел. Если вы сложили логарифмы трех чисел, делите полученный результат на три.
- Например, 2,878521796 / 3 = 0,959507265
3
Вычислите антилогарифм полученного результата. На калькуляторе нажмите кнопку переключения регистра (активирует функции верхнего регистра — над клавишами), а затем нажмите LOG, чтобы получить значение антилогарифма. Этот результат и будет средним геометрическим.^[5]
- Например, antilog 0,959507265 = 9,109766916. Поэтому среднее геометрическое 7, 9, и 12 равно 9,11.
Реклама

Советы

Различия между средним арифметическим и средним геометрическим:
- Для вычисления среднего арифметического, например, чисел 3, 4 и 18, необходимо их сложить 3 + 4 + 18, а затем разделить на 3 (потому что изначально даны три числа). Ответ равен 25/3 или примерно 8,333; это означает, что если сложить 8,3333 три раза подряд, то ответ будет таким же, как при сложении чисел 3, 4, и 18. Среднее арифметическое отвечает на вопрос: «Если все величины имеют одинаковое значение, то каким это значение должно быть, чтобы при суммировании получился один результат?»
- Напротив, среднее геометрическое отвечает на вопрос: «Если все величины имеют одинаковое значение, то каким это значение должно быть, чтобы при перемножении получился один результат?» Поэтому, чтобы найти среднее геометрическое чисел 3, 4 и 18, мы перемножаем эти числа: 3 x 4 x 18. Получаем 216. Затем мы берем кубический корень из полученного результата перемножения (кубический корень, так как в вычислении участвуют три числа). Ответ будет 6. Другими словами, так как 6 x 6 x 6 = 3 x 4 x 18, то 6 является средним геометрическим чисел 3, 4 и 18.
Среднее геометрическое всегда меньше или равно среднему арифметическому. Более подробно читайте тут.
Среднее геометрическое рассчитывается только для положительных чисел. Схема решения различных прикладных задач с использованием среднего геометрического не будет работать в случае наличия отрицательных чисел.

Об этой статье

Эту страницу просматривали 131 835 раз.

Была ли эта статья полезной?

Источник

Среднее геометрическое

Главная
/
Математика
/
Арифметика
/
Среднее геометрическое

Чтобы найти среднее геометрическое нескольких чисел воспользуйтесь нашим очень удобным онлайн калькулятором:

Онлайн калькулятор

Среднее геометрическое:

Округление ответа:

Просто введите положительные вещественные числа и получите среднее геометрическое этих чисел. Для того чтобы добавить в ряд более двух чисел воспользуйтесь зелёной кнопкой «+».

Теория

Среднее геометрическое нескольких положительных вещественных чисел – это такое число, которым можно заменить каждое из этих чисел так, чтобы их произведение не изменилось.

Формула

x̅_геом = ⁿ√x₁ ⋅ x₂ ⋅ … ⋅ x_n

Пример

К примеру, рассмотрим три числа 3, 8 и 9. Среднее геометрическое этих трёх чисел:

x̅_геом = ³√3 ⋅ 8 ⋅ 9 = ³√216 = 6

Таким образом:

3 ⋅ 8 ⋅ 9 = 216 = 6 ⋅ 6 ⋅ 6

См. также

Источник

В данной публикации мы рассмотрим, с помощью какой формулы можно найти среднее геометрическое чисел, а также разберем примеры задач для ее демонстрации на практике.

Расчет среднего геометрического
Пример задачи

Расчет среднего геометрического

Чтобы вычислить среднее геометрическое двух или более чисел, требуется их перемножить, а затем из полученного результата извлечь корень, степень которого равняется их количеству.

Допустим, у нас есть числа a₁, a₂, … , a_n. Среднее геометрическое находится по формуле:

Частные случаи формулы:

Пример задачи

Задание 1
Найдем среднее геометрическое чисел 3, 6 и 12.

Решение:
Воспользуемся соответствующей формулой для трех чисел:

Задание 2
Среднее геометрическое четырех чисел равняется 4, а также известны три из них – 2, 2 и 4. Найдем четвертое.

Решение:
Обозначим число, которое требуется найти буквой x. Формула выглядит следующим образом:

Помещаем число 4 под знак корня, сохранив равенство (для этого возводим его в четвертую степень, т.е. 4⁴ = 256):

Следовательно, x = 256 : 16 = 16.

Источник

In mathematics, the geometric mean is a mean or average which indicates a central tendency of a finite set of real numbers by using the product of their values (as opposed to the arithmetic mean which uses their sum). The geometric mean is defined as the nth root of the product of n numbers, i.e., for a set of numbers a₁, a₂, …, a_n, the geometric mean is defined as

${displaystyle left(prod _{i=1}^{n}a_{i}right)^{frac {1}{n}}={sqrt[{n}]{a_{1}a_{2}cdots a_{n}}}}$

or, equivalently, as the arithmetic mean in logscale:

${displaystyle exp {left({{frac {1}{n}}sum limits _{i=1}^{n}ln a_{i}}right)}}$

Most commonly the numbers are restricted to being non-negative, to avoid complications related to negative numbers not having real roots, and frequently they are restricted to being positive, to enable the use of logarithms.

For instance, the geometric mean of two numbers, say 2 and 8, is just the square root of their product, that is, . As another example, the geometric mean of the three numbers 4, 1, and 1/32 is the cube root of their product (1/8), which is 1/2, that is, .

The geometric mean is often used for a set of numbers whose values are meant to be multiplied together or are exponential in nature, such as a set of growth figures: values of the human population or interest rates of a financial investment over time. It also applies to benchmarking, where it is particularly useful for computing means of speedup ratios: since the mean of 0.5x (half as fast) and 2x (twice as fast) will be 1 (i.e., no speedup overall).

The geometric mean can be understood in terms of geometry. The geometric mean of two numbers, and , is the length of one side of a square whose area is equal to the area of a rectangle with sides of lengths and . Similarly, the geometric mean of three numbers, , , and , is the length of one edge of a cube whose volume is the same as that of a cuboid with sides whose lengths are equal to the three given numbers.

The geometric mean is one of the three classical Pythagorean means, together with the arithmetic mean and the harmonic mean. For all positive data sets containing at least one pair of unequal values, the harmonic mean is always the least of the three means, while the arithmetic mean is always the greatest of the three and the geometric mean is always in between (see Inequality of arithmetic and geometric means.)

Calculation[edit]

The geometric mean of a data set ${textstyle left{a_{1},a_{2},,ldots ,,a_{n}right}}$ is given by:

${displaystyle left(prod _{i=1}^{n}a_{i}right)^{frac {1}{n}}={sqrt[{n}]{a_{1}a_{2}cdots a_{n}}}.}$

^[3]

The above figure uses capital pi notation to show a series of multiplications. Each side of the equal sign shows that a set of values is multiplied in succession (the number of values is represented by «n») to give a total product of the set, and then the nth root of the total product is taken to give the geometric mean of the original set. For example, in a set of four numbers , the product of is , and the geometric mean is the fourth root of 24, or ~ 2.213. The exponent ${textstyle {frac {1}{n}}}$ on the left side is equivalent to the taking nth root. For example, ${textstyle 24^{frac {1}{4}}={sqrt[{4}]{24}}}$ .

Iterative means[edit]

The geometric mean of a data set is less than the data set’s arithmetic mean unless all members of the data set are equal, in which case the geometric and arithmetic means are equal. This allows the definition of the arithmetic-geometric mean, an intersection of the two which always lies in between.

The geometric mean is also the arithmetic-harmonic mean in the sense that if two sequences ( ${textstyle a_{n}}$ ) and ( ${textstyle h_{n}}$ ) are defined:

${displaystyle a_{n+1}={frac {a_{n}+h_{n}}{2}},quad a_{0}=x}$

and

${displaystyle h_{n+1}={frac {2}{{frac {1}{a_{n}}}+{frac {1}{h_{n}}}}},quad h_{0}=y}$

where ${textstyle h_{n+1}}$ is the harmonic mean of the previous values of the two sequences, then ${textstyle a_{n}}$ and ${textstyle h_{n}}$ will converge to the geometric mean of and . The sequences converge to a common limit, and the geometric mean is preserved:

${displaystyle {sqrt {a_{i}h_{i}}}={sqrt {frac {a_{i}+h_{i}}{frac {a_{i}+h_{i}}{h_{i}a_{i}}}}}={sqrt {frac {a_{i}+h_{i}}{{frac {1}{a_{i}}}+{frac {1}{h_{i}}}}}}={sqrt {a_{i+1}h_{i+1}}}}$

Replacing the arithmetic and harmonic mean by a pair of generalized means of opposite, finite exponents yields the same result.

Relationship with logarithms[edit]

The geometric mean can also be expressed as the exponential of the arithmetic mean of logarithms.^[4] By using logarithmic identities to transform the formula, the multiplications can be expressed as a sum and the power as a multiplication:

When ${displaystyle a_{1},a_{2},dots ,a_{n}>0}$

${displaystyle left(prod _{i=1}^{n}a_{i}right)^{frac {1}{n}}=exp left[{frac {1}{n}}sum _{i=1}^{n}ln a_{i}right];}$

As:

${displaystyle {begin{aligned}left(prod _{i=1}^{n}a_{i}right)^{frac {1}{n}}&={sqrt[{n}]{a_{1}a_{2}cdots a_{n}}}\&=e^{ln(a_{1}a_{2}cdots a_{n})^{1/n}}\&=e^{{frac {1}{n}}left(ln a_{1}+ln a_{2}+cdots +ln a_{n}right)}\&=e^{{frac {1}{n}}sum _{i=1}^{n}ln a_{i}}\{text{geometric mean(}}a{text{)}}&=e^{{text{arithmetic mean(ln(}}a{text{))}}}end{aligned}}}$

alternatively, use any positive real number base, for both the logarithms and the number you are raising to the power of the arithmetic mean of the individual logarithms at that same base.

additionally, if negative values of the $a_{i}$ are allowed,

${displaystyle left(prod _{i=1}^{n}a_{i}right)^{frac {1}{n}}=left(left(-1right)^{m}right)^{frac {1}{n}}exp left[{frac {1}{n}}sum _{i=1}^{n}ln left|a_{i}right|right],}$

where m is the number of negative numbers.

This is sometimes called the log-average (not to be confused with the logarithmic average). It is simply computing the arithmetic mean of the logarithm-transformed values of $a_{i}$ (i.e., the arithmetic mean on the log scale) and then using the exponentiation to return the computation to the original scale, i.e., it is the generalised f-mean with . For example, the geometric mean of 2 and 8 can be calculated as the following, where is any base of a logarithm (commonly 2, or 10):

${displaystyle b^{{frac {1}{2}}left[log _{b}(2)+log _{b}(8)right]}=4}$

Related to the above, it can be seen that for a given sample of points , the geometric mean is the minimizer of

${displaystyle f(a)=sum _{i=1}^{n}(log(a_{i})-log(a))^{2}=sum _{i=1}^{n}(log(a_{i}/a))^{2}}$

whereas the arithmetic mean is the minimizer of

${displaystyle f(a)=sum _{i=1}^{n}(a_{i}-a)^{2}}$

Thus, the geometric mean provides a summary of the samples whose exponent best matches the exponents of the samples (in the least squares sense).

The log form of the geometric mean is generally the preferred alternative for implementation in computer languages because calculating the product of many numbers can lead to an arithmetic overflow or arithmetic underflow. This is less likely to occur with the sum of the logarithms for each number.

Comparison to arithmetic mean[edit]

The geometric mean of a non-empty data set of (positive) numbers is always at most their arithmetic mean. Equality is only obtained when all numbers in the data set are equal; otherwise, the geometric mean is smaller. For example, the geometric mean of 2 and 3 is 2.45, while their arithmetic mean is 2.5. In particular, this means that when a set of non-identical numbers is subjected to a mean-preserving spread — that is, the elements of the set are «spread apart» more from each other while leaving the arithmetic mean unchanged — their geometric mean decreases.^[5]

Average growth rate[edit]

In many cases the geometric mean is the best measure to determine the average growth rate of some quantity. (For example, if in one year sales increases by 80% and the next year by 25%, the end result is the same as that of a constant growth rate of 50%, since the geometric mean of 1.80 and 1.25 is 1.50.) In order to determine the average growth rate, it is not necessary to take the product of the measured growth rates at every step. Let the quantity be given as the sequence ${displaystyle a_{0},a_{1},...,a_{n}}$ , where is the number of steps from the initial to final state. The growth rate between successive measurements $a_{k}$ and $a_{k+1}$ is $a_{k+1}/a_{k}$ . The geometric mean of these growth rates is then just:

${displaystyle left({frac {a_{1}}{a_{0}}}{frac {a_{2}}{a_{1}}}cdots {frac {a_{n}}{a_{n-1}}}right)^{frac {1}{n}}=left({frac {a_{n}}{a_{0}}}right)^{frac {1}{n}}.}$

Application to normalized values[edit]

The fundamental property of the geometric mean, which does not hold for any other mean, is that for two sequences and of equal length,

${displaystyle operatorname {GM} left({frac {X_{i}}{Y_{i}}}right)={frac {operatorname {GM} (X_{i})}{operatorname {GM} (Y_{i})}}}$

This makes the geometric mean the only correct mean when averaging normalized results; that is, results that are presented as ratios to reference values.^[6] This is the case when presenting computer performance with respect to a reference computer, or when computing a single average index from several heterogeneous sources (for example, life expectancy, education years, and infant mortality). In this scenario, using the arithmetic or harmonic mean would change the ranking of the results depending on what is used as a reference. For example, take the following comparison of execution time of computer programs:

Table 1

	Computer A	Computer B	Computer C
Program 1	1	10	20
Program 2	1000	100	20
Arithmetic mean	500.5	55	20
Geometric mean	31.622 . . .	31.622 . . .	20
Harmonic mean	1.998 . . .	18.182 . . .	20

The arithmetic and geometric means «agree» that computer C is the fastest. However, by presenting appropriately normalized values and using the arithmetic mean, we can show either of the other two computers to be the fastest. Normalizing by A’s result gives A as the fastest computer according to the arithmetic mean:

Table 2

	Computer A	Computer B	Computer C
Program 1	1	10	20
Program 2	1	0.1	0.02
Arithmetic mean	1	5.05	10.01
Geometric mean	1	1	0.632 . . .
Harmonic mean	1	0.198 . . .	0.039 . . .

while normalizing by B’s result gives B as the fastest computer according to the arithmetic mean but A as the fastest according to the harmonic mean:

Table 3

	Computer A	Computer B	Computer C
Program 1	0.1	1	2
Program 2	10	1	0.2
Arithmetic mean	5.05	1	1.1
Geometric mean	1	1	0.632
Harmonic mean	0.198 . . .	1	0.363 . . .

and normalizing by C’s result gives C as the fastest computer according to the arithmetic mean but A as the fastest according to the harmonic mean:

Table 4

	Computer A	Computer B	Computer C
Program 1	0.05	0.5	1
Program 2	50	5	1
Arithmetic mean	25.025	2.75	1
Geometric mean	1.581 . . .	1.581 . . .	1
Harmonic mean	0.099 . . .	0.909 . . .	1

In all cases, the ranking given by the geometric mean stays the same as the one obtained with unnormalized values.

However, this reasoning has been questioned.^[7]
Giving consistent results is not always equal to giving the correct results. In general, it is more rigorous to assign weights to each of the programs, calculate the average weighted execution time (using the arithmetic mean), and then normalize that result to one of the computers. The three tables above just give a different weight to each of the programs, explaining the inconsistent results of the arithmetic and harmonic means (Table 4 gives equal weight to both programs, the Table 2 gives a weight of 1/1000 to the second program, and the Table 3 gives a weight of 1/100 to the second program and 1/10 to the first one). The use of the geometric mean for aggregating performance numbers should be avoided if possible, because multiplying execution times has no physical meaning, in contrast to adding times as in the arithmetic mean. Metrics that are inversely proportional to time (speedup, IPC) should be averaged using the harmonic mean.

The geometric mean can be derived from the generalized mean as its limit as goes to zero. Similarly, this is possible for the weighted geometric mean.

Geometric mean of a continuous function[edit]

If is a positive continuous real-valued function, its geometric mean over this interval is

${displaystyle {text{GM}}[f]=exp left({frac {1}{b-a}}int _{a}^{b}ln f(x)dxright)}$

For instance, taking the identity function f(x)=x over the unit interval shows that the geometric mean of the positive numbers between 0 and 1 is equal to ${frac {1}{e}}$ .

Applications[edit]

Proportional growth[edit]

The geometric mean is more appropriate than the arithmetic mean for describing proportional growth, both exponential growth (constant proportional growth) and varying growth; in business the geometric mean of growth rates is known as the compound annual growth rate (CAGR). The geometric mean of growth over periods yields the equivalent constant growth rate that would yield the same final amount.

Suppose an orange tree yields 100 oranges one year and then 180, 210 and 300 the following years, so the growth is 80%, 16.6666% and 42.8571% for each year respectively. Using the arithmetic mean calculates a (linear) average growth of 46.5079% (80% + 16.6666% + 42.8571%, that sum then divided by 3). However, if we start with 100 oranges and let it grow 46.5079% each year, the result is 314 oranges, not 300, so the linear average over-states the year-on-year growth.

Instead, we can use the geometric mean. Growing with 80% corresponds to multiplying with 1.80, so we take the geometric mean of 1.80, 1.166666 and 1.428571, i.e. ; thus the «average» growth per year is 44.2249%. If we start with 100 oranges and let the number grow with 44.2249% each year, the result is 300 oranges.

Financial[edit]

The geometric mean has from time to time been used to calculate financial indices (the averaging is over the components of the index). For example, in the past the FT 30 index used a geometric mean.^[8] It is also used in the recently introduced «RPIJ» measure of inflation in the United Kingdom and in the European Union.

This has the effect of understating movements in the index compared to using the arithmetic mean.^[8]

[edit]

Although the geometric mean has been relatively rare in computing social statistics, starting from 2010 the United Nations Human Development Index did switch to this mode of calculation, on the grounds that it better reflected the non-substitutable nature of the statistics being compiled and compared:

The geometric mean decreases the level of substitutability between dimensions [being compared] and at the same time ensures that a 1 percent decline in say life expectancy at birth has the same impact on the HDI as a 1 percent decline in education or income. Thus, as a basis for comparisons of achievements, this method is also more respectful of the intrinsic differences across the dimensions than a simple average.^[9]

Not all values used to compute the HDI (Human Development Index) are normalized; some of them instead have the form ${displaystyle left(X-X_{text{min}}right)/left(X_{text{norm}}-X_{text{min}}right)}$ . This makes the choice of the geometric mean less obvious than one would expect from the «Properties» section above.

The equally distributed welfare equivalent income associated with an Atkinson Index with an inequality aversion parameter of 1.0 is simply the geometric mean of incomes. For values other than one, the equivalent value is an Lp norm divided by the number of elements, with p equal to one minus the inequality aversion parameter.

Geometry[edit]

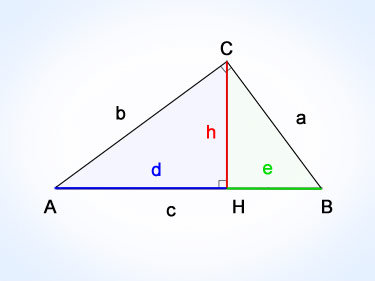
The altitude of a right triangle from its right angle to its hypotenuse is the geometric mean of the lengths of the segments the hypotenuse is split into. Using Pythagoras’ theorem on the 3 triangles of sides (p + q, r, s ), (r, p, h ) and (s, h, q ),
${displaystyle {begin{aligned}(p+q)^{2};;&=quad r^{2};;,+quad s^{2}\p^{2}!!+!2pq!+!q^{2}&=overbrace {p^{2}!!+!h^{2}} +overbrace {h^{2}!!+!q^{2}} \2pqquad ;;;&=2h^{2};therefore h!=!{sqrt {pq}}\end{aligned}}}$

In the case of a right triangle, its altitude is the length of a line extending perpendicularly from the hypotenuse to its 90° vertex. Imagining that this line splits the hypotenuse into two segments, the geometric mean of these segment lengths is the length of the altitude. This property is known as the geometric mean theorem.

In an ellipse, the semi-minor axis is the geometric mean of the maximum and minimum distances of the ellipse from a focus; it is also the geometric mean of the semi-major axis and the semi-latus rectum. The semi-major axis of an ellipse is the geometric mean of the distance from the center to either focus and the distance from the center to either directrix.

Another way to think about it is as follows:

Consider a circle with radius . Now take two diametrically opposite points on the circle and apply pressure from both ends to deform it into an ellipse with semi-major and semi-minor axes of lengths and .

Since the area of the circle and the ellipse stays the same, we have:

${displaystyle {begin{aligned}pi r^{2}&=pi ab\r^{2}&=ab\r&={sqrt {ab}}end{aligned}}}$

The radius of the circle is the geometric mean of the semi-major and the semi-minor axes of the ellipse formed by deforming the circle.

Distance to the horizon of a sphere (ignoring the effect of atmospheric refraction when atmosphere is present) is equal to the geometric mean of the distance to the closest point of the sphere and the distance to the farthest point of the sphere.

The geometric mean is used in both in the approximation of squaring the circle by S.A. Ramanujan^[10] and in the construction of the heptadecagon with «mean proportionals».^[11]

Aspect ratios[edit]

Equal area comparison of the aspect ratios used by Kerns Powers to derive the SMPTE 16:9 standard.^[12] TV 4:3/1.33 in red,

1.66 in orange,

16:9/1.77 in blue,

1.85 in yellow,

Panavision/2.2 in mauve and

CinemaScope/2.35 in purple.

The geometric mean has been used in choosing a compromise aspect ratio in film and video: given two aspect ratios, the geometric mean of them provides a compromise between them, distorting or cropping both in some sense equally. Concretely, two equal area rectangles (with the same center and parallel sides) of different aspect ratios intersect in a rectangle whose aspect ratio is the geometric mean, and their hull (smallest rectangle which contains both of them) likewise has the aspect ratio of their geometric mean.

In the choice of 16:9 aspect ratio by the SMPTE, balancing 2.35 and 4:3, the geometric mean is ${textstyle {sqrt {2.35times {frac {4}{3}}}}approx 1.7701}$ , and thus … was chosen. This was discovered empirically by Kerns Powers, who cut out rectangles with equal areas and shaped them to match each of the popular aspect ratios. When overlapped with their center points aligned, he found that all of those aspect ratio rectangles fit within an outer rectangle with an aspect ratio of 1.77:1 and all of them also covered a smaller common inner rectangle with the same aspect ratio 1.77:1.^[12] The value found by Powers is exactly the geometric mean of the extreme aspect ratios, 4:3 (1.33:1) and CinemaScope (2.35:1), which is coincidentally close to (). The intermediate ratios have no effect on the result, only the two extreme ratios.

Applying the same geometric mean technique to 16:9 and 4:3 approximately yields the 14:9 (…) aspect ratio, which is likewise used as a compromise between these ratios.^[13] In this case 14:9 is exactly the arithmetic mean of and , since 14 is the average of 16 and 12, while the precise geometric mean is ${textstyle {sqrt {{frac {16}{9}}times {frac {4}{3}}}}approx 1.5396approx 13.8:9,}$ but the two different means, arithmetic and geometric, are approximately equal because both numbers are sufficiently close to each other (a difference of less than 2%).

Paper formats[edit]

The geometric mean is also used to calculate B and C series paper formats. The $B_{n}$ format has an area which is the geometric mean of the areas of $A_{n}$ and ${displaystyle A_{n-1}}$ . For example, the area of a B1 paper is ${displaystyle {frac {sqrt {2}}{2}}mathrm {m} ^{2}}$ , because it is the geometric mean of the areas of an A0 ( ${displaystyle 1mathrm {m} ^{2}}$ ) and an A1 ( ${displaystyle {frac {1}{2}}mathrm {m} ^{2}}$ ) paper ( ${displaystyle {sqrt {1mathrm {m} ^{2}cdot {frac {1}{2}}mathrm {m} ^{2}}}={sqrt {{frac {1}{2}}mathrm {m} ^{4}}}={frac {1}{sqrt {2}}}mathrm {m} ^{2}={frac {sqrt {2}}{2}}mathrm {m} ^{2}}$ ).

The same principle applies with the C series, whose area is the geometric mean of the A and B series. For example, the C4 format has an area which is the geometric mean of the areas of A4 and B4.

An advantage that comes from this relationship is that an A4 paper fits inside a C4 envelope, and both fit inside a B4 envelope.

Other applications[edit]

Spectral flatness: in signal processing, spectral flatness, a measure of how flat or spiky a spectrum is, is defined as the ratio of the geometric mean of the power spectrum to its arithmetic mean.
Anti-reflective coatings: In optical coatings, where reflection needs to be minimised between two media of refractive indices n₀ and n₂, the optimum refractive index n₁ of the anti-reflective coating is given by the geometric mean: $n_{1}={sqrt {n_{0}n_{2}}}$ .
Subtractive color mixing: The spectral reflectance curve for paint mixtures (of equal tinting strength, opacity and dilution) is approximately the geometric mean of the paints’ individual reflectance curves computed at each wavelength of their spectra.^[14]
Image processing: The geometric mean filter is used as a noise filter in image processing.
Labor compensation: The geometric mean of a subsistence wage and market value of the labor using capital of employer was suggested as the natural wage by Johann von Thünen in 1875.^[15]

Notes[edit]

^ If AC = a and BC = b. OC = AM of a and b, and radius r = QO = OG.
Using Pythagoras’ theorem, QC² = QO² + OC² ∴ QC = √QO² + OC² = QM.
Using Pythagoras’ theorem, OC² = OG² + GC² ∴ GC = √OC² − OG² = GM.
Using similar triangles, HC/GC = GC/OC ∴ HC = GC²/OC = HM.

References[edit]

^ Matt Friehauf, Mikaela Hertel, Juan Liu, and Stacey Luong «On Compass and Straightedge Constructions: Means» (PDF). UNIVERSITY of WASHINGTON, DEPARTMENT OF MATHEMATICS. 2013. Retrieved 14 June 2018.
^ «Euclid, Book VI, Proposition 13». David E. Joyce, Clark University. 2013. Retrieved 19 July 2019.
^ «2.5: Geometric Mean». Statistics LibreTexts. 2019-04-20. Retrieved 2021-08-16.
^ Crawley, Michael J. (2005). Statistics: An Introduction using R. John Wiley & Sons Ltd. ISBN 9780470022986.
^ Mitchell, Douglas W. (2004). «More on spreads and non-arithmetic means». The Mathematical Gazette. 88: 142–144. doi:10.1017/S0025557200174534. S2CID 168239991.
^ Fleming, Philip J.; Wallace, John J. (1986). «How not to lie with statistics: the correct way to summarize benchmark results». Communications of the ACM. 29 (3): 218–221. doi:10.1145/5666.5673. S2CID 1047380.
^ Smith, James E. (1988). «Characterizing computer performance with a single number». Communications of the ACM. 31 (10): 1202–1206. doi:10.1145/63039.63043. S2CID 10805363.
^ ^a ^b Rowley, Eric E. (1987). The Financial System Today. Manchester University Press. ISBN 0719014875.
^ «Frequently Asked Questions — Human Development Reports». hdr.undp.org. Archived from the original on 2011-03-02.
^ Ramanujan, S. (1914). «Modular equations and approximations to π» (PDF). Quarterly Journal of Mathematics. 45: 350–372.
^ T.P. Stowell Extract from Leybourn’s Math. Repository, 1818 in The Analyst via Google Books
^ ^a ^b «TECHNICAL BULLETIN: Understanding Aspect Ratios» (PDF). The CinemaSource Press. 2001. Archived (PDF) from the original on 2009-09-09. Retrieved 2009-10-24.
^ US 5956091, «Method of showing 16:9 pictures on 4:3 displays», issued September 21, 1999
^ MacEvoy, Bruce. «Colormaking Attributes: Measuring Light & Color». handprint.com/LS/CVS/color.html. Colorimetry. Archived from the original on 2019-07-14. Retrieved 2020-01-02.
^ Henry Ludwell Moore (1895). Von Thünen’s Theory of Natural Wages. G. H. Ellis.

External links[edit]

Calculation of the geometric mean of two numbers in comparison to the arithmetic solution
Arithmetic and geometric means
When to use the geometric mean
Practical solutions for calculating geometric mean with different kinds of data Archived 2010-11-12 at the Wayback Machine
Geometric Mean on MathWorld
Geometric Meaning of the Geometric Mean
Geometric Mean Calculator for larger data sets
Computing Congressional apportionment using Geometric Mean
Non-Newtonian calculus website
Geometric Mean Definition and Formula
The Distribution of the Geometric Mean
The geometric mean?

Источник

Среднее геометрическое

Предлагаемая здесь программа, помимо расчета среднего геометрического, умеет еще и приводить исходные данные к стандартному виду, а так же упорядочивать их по возрастанию или убыванию…

Содержание:

Определение среднего геометрического
Расчет среднего геометрического
Свойства среднего геометрического
Прикладное значение среднего геометрического

Среднее геометрическое или среднее пропорциональное используется человечеством в архитектурных, землемерных и инженерных расчетах не менее 2500 лет. Об этом достоверно известно благодаря математическому трактату Евклида «Начала».
В своей второй теореме Евклид доказывает, что в прямоугольном треугольнике высота проведенная из прямого угла (рисунок) делит противоположную сторону так что:

h =

√

Собственно говоря, благодаря второй теореме Евклида среднее геометрическое и получило свое название. В древнем мире математики ограничивалось только использованием корня квадратного (геометрия) и корня кубического (стереометрия).
Вообще говоря, извлечение корня с различными целыми показателями является частным случаем дробной степени. Но к такому пониманию этих алгебраических операции математики подошли только в семнадцатом веке. Неоценимый вклад в достижении обобщенного понимания степенных алгебраических операции внес Рене Декарт.

В свете современных представлений:

Среднее геометрическое значение множества положительных вещественных чисел определяется как результат взаимного умножения этих чисел и извлечения из произведения корня с показателем равным количеству чисел:

a_{ср.геом} = ⁿ

√

Таким образом, мы имеем дело исключительно с положительными вещественными числами и находим такое число, что при замене каждого из этих чисел их произведение не изменяется.

Расчет среднего геометрического

Для того чтобы начать онлайн расчет среднего геометрического введите исходные числа в одно из полей ввода-вывода данных.
В первое поле можно ввести последовательность чисел, разделенных точкой с запятой (программа попытается так же преобразовать к стандартному виду, например, вставленную копию последовательности чисел с плавающей точкой, разделенных пробелами, запятой или точкой с запятой).
Во второе поле можно вводить числа по одному — они автоматически будут добавляться к данным первого поля, если расчет не запустился автоматически, кликните по зеленой кнопке, показывающей количество чисел в исследуемом массиве:

Введите исходные данные

Введите число

Что-то пошло не так…
Прямое восхождение не может быть больше 24 часов,
минуты и секунды больше 60,
а склонение по абсолютной величине не должно быть больше 90°

Среднее геометрическое, a_{ср. геом}

Для наглядной демонстрации правила о средних

a_{ср. геом} ≤ a _ср. _арифм

выводим так же результат расчета среднего арифметического:

Среднее арифметическое^[1], a_{ср. арифм}

a_{среднее}_{геометрическое} ≤ a _{среднее}_{арифметическое}

Design by Sergey Ov for abc2home.ru

ВНИМАНИЕ! При перезагрузке страницы введенная информация не сохраняется, если Вы не сгенерировали код для записи результатов работы в командной строке:

Сохранить расчет среднего геометрического в истории браузера

Адресную строку с кодом из Ваших данных Вы можете переслать на любое устройство и воспроизвести на нем результаты расчетов

После того как будут введены хотя бы два исходных числа, цвет квадратной кнопки на поле ввода данных должен поменяться с оранжевого на зеленый, и автоматически начнется расчет среднего геометрического и сопутствующих параметров, если это не произошло, то кликните по зеленому полю кнопки.

Страницы по теме «Расчет средних значений»

Среднее арифметическое — расчет онлайн, определение, формула
Среднеквадратическое отклонение — расчет онлайн, определение, формула
Среднее геометрическое — расчет онлайн, определение, формула
Среднее гармоническое и среднее степенное — расчет онлайн, определения, формулы
Среднее квадратическое — расчет онлайн, определение, формула

Свойства среднего геометрического

1. Среднее геометрическое значение множества заданных неотрицательных чисел лежит между минимальным и максимальным числами из этого множества.

2. Кроме того среднее геометрическое подчиняется неравенству о средних для множества положительных вещественных чисел

a_min ≤ a_{ср. гарм} ≤ a_{ср. геом} ≤ a_{ср. арифм} ≤ a _{ср.квадр} ≤ a _max ^[2] ,

то есть для любого множества положительных чисел среднее геометрическое никогда не бывает больше среднего арифметического^[1]:

ⁿ

√

≤

Прикладное значение среднего геометрического

Среднее геометрическое широко используется в демографической статистике, моделирований социального развития общества.
С применением среднего геометрического в экономике
расcчитываются финансовые индексы, в физике — коэффициент преломления антибликового напыления, а в вычислительной математике осуществляется сглаживание шумов.

P.S. На этой странице используется Бета версия программы расчета среднего геометрического, об обнаруженных недочетах, а так же возможных пожеланиях просьба сообщить на форум сайта (окно для входа на форум находится в нижней части страницы).

1. Среднее арифметическоезначение (чаще используется термин, просто, «среднее арифметическое» или «среднее») множества заданных чисел определяется как число равное сумме всех чисел множества, делённой на их количество:

a_{ср.арифм} =

2. Среднее степенное значение s_d порядка (степени) d от множества заданных чисел a₁+ a₂+ …+ a_n определяется формулой:

s_d =

(

)

1
d

Среднее арифметическое является степенным средним c d = 1, среднее квадратическое — d = 2, среднее гармоническое можно считать степенным средним порядка d = -1.

● Главная
▸ Статьи
▸ Блог
▸ Копилка
✔ Среднее геометрическое — расчет онлайн

Источник

Советы

Об этой статье

Была ли эта статья полезной?

Среднее геометрическое

Онлайн калькулятор

Теория

Формула

Пример

См. также

Расчет среднего геометрического

Пример задачи

Calculation[edit]

Iterative means[edit]

Relationship with logarithms[edit]

Comparison to arithmetic mean[edit]

Average growth rate[edit]

Application to normalized values[edit]

Geometric mean of a continuous function[edit]

Applications[edit]

Proportional growth[edit]

Financial[edit]

[edit]

Geometry[edit]

Aspect ratios[edit]

Paper formats[edit]

Other applications[edit]

See also[edit]

Notes[edit]

References[edit]

External links[edit]

Среднее геометрическое

Расчет среднего геометрического

Что-то пошло не так… Прямое восхождение не может быть больше 24 часов, минуты и секунды больше 60, а склонение по абсолютной величине не должно быть больше 90°

Страницы по теме «Расчет средних значений»

Свойства среднего геометрического

Прикладное значение среднего геометрического

Что-то пошло не так…
Прямое восхождение не может быть больше 24 часов,
минуты и секунды больше 60,
а склонение по абсолютной величине не должно быть больше 90°