Как найти радиус окружности зная угол дуги - Исправление недочетов и поиск решений вместе с Examum.ru

Нахождение длины дуги сектора круга

В данной публикации мы рассмотрим формулы, с помощью которых можно вычислить длину дуги сектора круга, а также разберем примеры решения задач для демонстрации их применения на практике.

Определение дуги сектора круга

Дуга – это участок между двумя точками на окружности.

Дуга сектора круга – это участок между двумя точками на окружности, которые получены в результате пересечения этой окружности двумя радиусами, образовавшими сектор круга.

На рисунке ниже: AB – это дуга зеленого сектора круга с радиусом R (или r).

OA = OB = R (r);
α – угол сектора или центральный угол.

Формулы для нахождения длины дуги сектора

Через центральный угол в градусах и радиус

Длина (L) дуги сектора равняется числу π , умноженному на радиус круга (r), умноженному на центральный угол в градусах ( α°), деленному на 180°.

Примечание: в расчетах используется число π , приблизительно равное 3,14.

Через угол сектора в радианах и радиус

Длина (L) дуги сектора равна произведению радиуса (r) и центрального угла, выраженного в радианах (a_рад).

Примеры задач

Задание 1
Дан круг с радиусом 15 см. Найдите длину дуги сектора, угол которого равен 30°.

Решение
Воспользуемся формулой расчета, в которой используется центральный угол в градусах:

Задание 2
Длина дуги сектора равняется 24 см. Найдите, чему равен его угол (в радианах и градусах), если радиус круга составляет 12 см.

Решение
Для начала вычислим угол в радианах:

1 радиан ≈ 57,2958°

Следовательно, центральный угол приблизительно равняется 114,59 ° (2 рад ⋅ 57,2958°).

Сегмент круга

Вычисляет площадь, длину дуги, длину хорды, высоту и периметр сегмента круга. Описывается несколько вариантов расчета по параметрам сегмента — по углу, по хорде, по радиусу, по высоте и длине дуги.

Сегмент круга

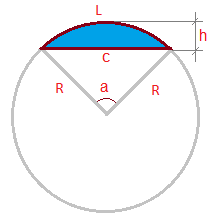
Круговой сегмент — часть круга ограниченная дугой и секущей (хордой).

На рисунке:
L — длина дуги сегмента
c — хорда
R — радиус
a — угол сегмента
h — высота

Первый калькулятор рассчитывает параметры сегмента, если известен радиус и угол по следующим формулам:

Формулы вычисления параметров сегмента

Площадь сегмента:
[1]
Длина дуги:

Как найти радиус окружности

О чем эта статья:

Статья находится на проверке у методистов Skysmart.
Если вы заметили ошибку, сообщите об этом в онлайн-чат (в правом нижнем углу экрана).

Основные понятия

Прежде чем погружаться в последовательность расчетов, важно понять разницу между понятиями.

Окружность — замкнутая плоская кривая, все точки которой равноудалены от центра, которая лежит в той же плоскости. Если говорить проще, то это замкнутая линия, как, например, обруч и кольцо.

Круг — множество точек на плоскости, которые удалены от центра на расстоянии равном радиусу. Иначе говоря, плоская фигура, ограниченная окружностью, как мяч и блюдце.

Радиус — это отрезок, который соединяет центр окружности и любую точку на ней. Общепринятое обозначение радиуса — латинская буква R.

Возможно тебе интересно узнать — как найти длину окружности?

Формула радиуса окружности

Определить способ вычисления проще, отталкиваясь от исходных данных. Далее рассмотрим девять формул разной степени сложности.

Если известна площадь круга

R = √ S : π, где S — площадь круга, π — это константа, которая выражает отношение длины окружности к диаметру, она всегда равна 3,14.

Если известна длина

R = P : 2 * π, где P — длина (периметр круга).

Для тех, кто хочет связать свою жизнь с точными науками, Skysmart предлагает курс подготовки к ЕГЭ по математике (профиль).

Если известен диаметр окружности

R = D : 2, где D — диаметр.

Диаметр — отрезок, который соединяет две точки окружности и проходит через центр. Радиус всегда равен половине диаметра.

Если известна диагональ вписанного прямоугольника

R = d : 2, где d — диагональ.

Диагональ вписанного прямоугольник делит фигуру на два прямоугольных треугольника и является их гипотенузой — стороной, лежащей напротив прямого угла. Если диагональ неизвестна, теорема Пифагора поможет её вычислить:

d = √ a 2 + b 2 , где a, b — стороны вписанного прямоугольника.

Если известна сторона описанного квадрата

R = a : 2, где a — сторона.

Сторона описанного квадрата равна диаметру окружности.

Если известны стороны и площадь вписанного треугольника

R = (a * b * c) : (4 * S), где a, b, с — стороны, S — площадь треугольника.

Если известна площадь и полупериметр описанного треугольника

R = S : p, где S — площадь треугольника, p — полупериметр треугольника.

Полупериметр треугольника — это сумма длин всех его сторон, деленная на два.

Если известна площадь сектора и его центральный угол

R = √ (360° * S) : (π * α), где S — площадь сектора круга, α — центральный угол.

Площадь сектора круга — это часть S всей фигуры, ограниченной окружностью с радиусом.

Если известна сторона вписанного правильного многоугольника

R = a : (2 * sin (180 : N)), где a — сторона правильного многоугольника, N — количество сторон.

В правильном многоугольнике все стороны равны.

Скачать онлайн таблицу

У каждой геометрической фигуры много формул — запомнить все сразу бывает действительно сложно. В этом деле поможет регулярное решение задач и частый просмотр формул. Можно распечатать эту таблицу и использовать, как закладку в тетрадке или учебнике, и обращаться к ней по необходимости.

источники:

http://planetcalc.ru/1421/

http://skysmart.ru/articles/mathematic/radius-okruzhnosti

Источник

как найти радиус окружности, если известна градусная мера и длина дуги.

Обозначим L — длина дуги, α — градусная мера дуги, R — радиус окружности.

L = πR · α / 180°

πR · α = L · 180°

R = L · 180° / (π·α)
- Комментировать
- Жалоба
- Ссылка

Найди верный ответ на вопрос ✅ «как найти радиус окружности, если известна градусная мера и длина дуги. …» по предмету 📙 Геометрия, а если ответа нет или никто не дал верного ответа, то воспользуйся поиском и попробуй найти ответ среди похожих вопросов.

Искать другие ответы

Новые вопросы по геометрии

Главная » Геометрия » как найти радиус окружности, если известна градусная мера и длина дуги.

Источник

Радиус окружности при заданной длине дуги Решение

ШАГ 0: Сводка предварительного расчета

ШАГ 1. Преобразование входов в базовый блок

Длина дуги окружности: 15 метр —> 15 метр Конверсия не требуется
Центральный угол окружности: 170 степень —> 2.9670597283898 Радиан (Проверьте преобразование здесь)

ШАГ 2: Оцените формулу

ШАГ 3: Преобразуйте результат в единицу вывода

5.05550995703763 метр —> Конверсия не требуется

4 Радиус круга Калькуляторы

Радиус окружности при заданной длине дуги формула

Радиус круга = Длина дуги окружности/Центральный угол окружности

r = l_Arc/∠_Central

Что такое Круг?

Окружность — это базовая двухмерная геометрическая фигура, которая определяется как совокупность всех точек на плоскости, находящихся на фиксированном расстоянии от фиксированной точки. Фиксированная точка называется центром круга, а фиксированное расстояние называется радиусом круга. Когда два радиуса становятся коллинеарными, эта общая длина называется диаметром круга. То есть диаметр — это длина отрезка внутри круга, проходящего через центр, и он будет в два раза больше радиуса.

Источник

Как найти радиус окружности, если известна градусная мера и длина дуги.

Если вам необходимо получить ответ на вопрос Как найти радиус окружности, если известна градусная мера и длина дуги?, относящийся
к уровню подготовки учащихся 5 — 9 классов, вы открыли нужную страницу.
В категории Геометрия вы также найдете ответы на похожие вопросы по
интересующей теме, с помощью автоматического «умного» поиска. Если после
ознакомления со всеми вариантами ответа у вас остались сомнения, или
полученная информация не полностью освещает тематику, создайте свой вопрос с
помощью кнопки, которая находится вверху страницы, или обсудите вопрос с
посетителями этой страницы.

Источник

Download Article

The radius of a circle is the distance from the center of the circle to any point on its circumference.^[1] The easiest way to find the radius is by dividing the diameter in half. If you don’t know the diameter but you know other measurements, such as the circle’s circumference () or area ( ${displaystyle A=pi r^{2}}$ ), you can still find the radius by using the formulas and isolating the variable.

1

Write down the circumference formula. The formula is

, where equals the circle’s circumference, and equals its radii^[2]
2

Solve for r. Use algebra to change the circumference formula until r (radius) is alone on one side of the equation:

Example

${frac {C}{2pi }}={frac {2pi r}{2pi }}$
${frac {C}{2pi }}=r$
$r={frac {C}{2pi }}$

Advertisement
3

Plug the circumference into the formula. Whenever a math problem tells you the circumference C of a circle, you can use this equation to find the radius r. Replace C in the equation with the circumference of the circle in your problem:

Example
If the circumference is 15 centimeters, your formula will look like this: $r={frac {15}{2pi }}$ centimeters
4

Round to a decimal answer. Enter your result in a calculator with the button and round the result. If you don’t have a calculator, calculate it by hand, using 3.14 as a close estimate for .

Example
$r={frac {15}{2pi }}=$ about ${frac {7.5}{2*3.14}}=$ approximately 2.39 centimeters

1

Set up the formula for the area of a circle. The formula is

$A=pi r^{{2}}$

, where equals the area of the circle, and equals the radius.^[3]
2

Solve for the radius. Use algebra to get the radius r alone on one side of the equation:

Example
Divide both sides by :
$A=pi r^{{2}}$
${frac {A}{pi }}=r^{{2}}$
Take the square root of both sides:
${sqrt {{frac {A}{pi }}}}=r$
$r={sqrt {{frac {A}{pi }}}}$
3

Plug the area into the formula. Use this formula to find the radius when the problem tells you the area of the circle. Substitute the area of the circle for the variable .

Example
If the area of the circle is 21 square centimeters, the formula will look like this: $r={sqrt {{frac {21}{pi }}}}$
4

Divide the area by . Begin solving the problem by simplifying the portion under the square root ( ${frac {A}{pi }})$ . Use a calculator with a key if possible. If you don’t have a calculator, use 3.14 as an estimate for .

Example
If using 3.14 for , you would calculate:
$r={sqrt {{frac {21}{3.14}}}}$

If your calculator allows you to enter the whole formula on one line, that will give you a more accurate answer.
5

Take the square root.

You will likely need a calculator to do this

, because the number will be a decimal. This value will give you the radius of the circle.

Example
. So, the radius of a circle with an area of 21 square centimeters is about 2.59 centimeters.
Areas always use square units (like square centimeters), but the radius always uses units of length (like centimeters). If you keep track of units in this problem, you’ll notice that ${sqrt {cm^{{2}}}}=cm$ .

1
Check the problem for a diameter. If the problem tells you the diameter of the circle, it’s easy to find the radius. If you are working with an actual circle,

measure the diameter by placing a ruler so its edge passes straight through the circle’s center

, touching the circle on both sides.^[4]
- If you’re not sure where the circle center is, put the ruler down across your best guess. Hold the zero mark of the ruler steady against the circle, and slowly move the other end back and forth around the circle’s edge. The highest measurement you can find is the diameter.
- For example, you might have a circle with a diameter of 4 centimeters.
2
Divide the diameter by two. A circle’s

radius is always half the length of its diameter.

^[5]
- For example, if the diameter is 4 cm, the radius equals 4 cm ÷ 2 = 2 cm.
- In math formulas, the radius is r and the diameter is d. You might see this step in your textbook as $r={frac {d}{2}}$ .

1

Set up the formula for the area of a sector. The formula is

$A_{{sector}}={frac {theta }{360}}(pi )(r^{{2}})$

, where $A_{{sector}}$ equals the area of the sector, equals the central angle of the sector in degrees, and equals the radius of the circle.^[6]
2

Plug the sector’s area and central angle into the formula. This information should be given to you.

Make sure you have the area of the sector, not the area for the circle.

Substitute the area for the variable $A_{{sector}}$ and the angle for the variable .

Example
If the area of the sector is 50 square centimeters, and the central angle is 120 degrees, you would set up the formula like this:
$50={frac {120}{360}}(pi )(r^{{2}})$ .
3

Divide the central angle by 360. This will tell you what fraction of the entire circle the sector represents.

Example
${frac {120}{360}}={frac {1}{3}}$ . This means that the sector is ${frac {1}{3}}$ of the circle.
Your equation should now look like this: $50={frac {1}{3}}(pi )(r^{{2}})$
4

Isolate $(pi )(r^{{2}})$ . To do this, divide both sides of the equation by the fraction or decimal you just calculated.

Example
$50={frac {1}{3}}(pi )(r^{{2}})$
${frac {50}{{frac {1}{3}}}}={frac {{frac {1}{3}}(pi )(r^{{2}})}{{frac {1}{3}}}}$
$150=(pi )(r^{{2}})$
5

Divide both sides of the equation by . This will isolate the variable. For a more precise result, use a calculator. You can also round to 3.14.

Example
$150=(pi )(r^{{2}})$
${frac {150}{pi }}={frac {(pi )(r^{{2}})}{pi }}$
$47.7=r^{{2}}$
6

Take the square root of both sides. This will give you the radius of the circle.

Example
$47.7=r^{{2}}$
${sqrt {47.7}}={sqrt {r^{{2}}}}$

So, the radius of the circle is about 6.91 centimeters.

Practice Problems and Answers

Add New Question

Question

How do I find the radius of a circle when I know the chord length?

It is possible to have quite a few circles, all with different radii, in which one could draw a chord of a given, fixed length. Hence, the chord length by itself cannot determine the radius of the circle.
Question

How do I find the radius of a circle when I know the arc length and the central angle?

Divide the central angle into 360°. Multiply the resulting number by the arc length. That gives you the circumference of the circle. Divide the circumference by pi. That’s the diameter. Half of the diameter is the radius of the circle.
Question

How do I calculate the radius of a circle when no other values are known?

Technically you can’t «calculate» the radius in such a situation. However, it is possible, by construction, to locate the center of such a circle, and then, simply by physically measuring, determine the radius. To do the construction, draw any two chords and construct their perpendicular bisectors; their point of intersection is the center of the circle. Then draw in any radius and measure it with a ruler. Not technically a «calculation.»

See more answers

Ask a Question

200 characters left

Include your email address to get a message when this question is answered.

Submit

About This Article

Article SummaryX

To calculate the radius of a circle by using the circumference, take the circumference of the circle and divide it by 2 times π. For a circle with a circumference of 15, you would divide 15 by 2 times 3.14 and round the decimal point to your answer of approximately 2.39. Be sure to include the units in your answer. To learn more, such as how to calculate the radius with the area or diameter, keep reading the article!

Did this summary help you?

Thanks to all authors for creating a page that has been read 3,354,374 times.

Did this article help you?

Источник