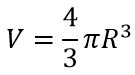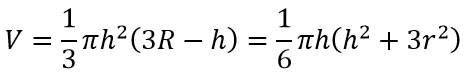Как найти радиус полусферы - Исправление недочетов и поиск решений вместе с Examum.ru

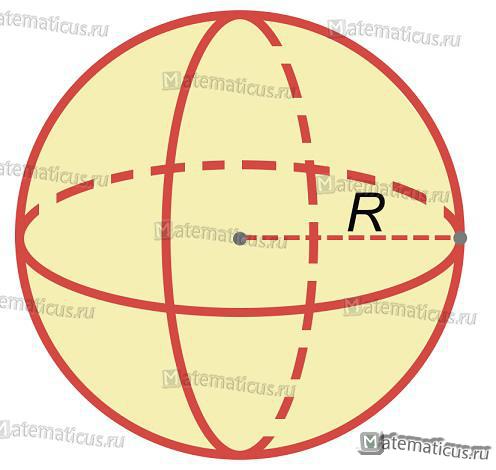
Сфера

Сфера (от греч. «сфайра» — «шар», «мяч») — это геометрическое место точек в пространстве, равноудаленных от некоторой заданной точки в центре сферы.

Шар — это тело, ограниченное сферой.

Радиус сферы — отрезок, соединяющий центр и любую точку сферы.

Получить сферу можно вращением полуокружности вокруг ее диаметра.

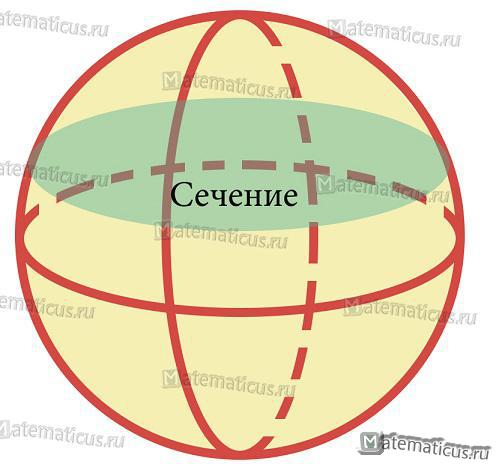
Секущая плоскость делит сферу на два шаровых сегмента.

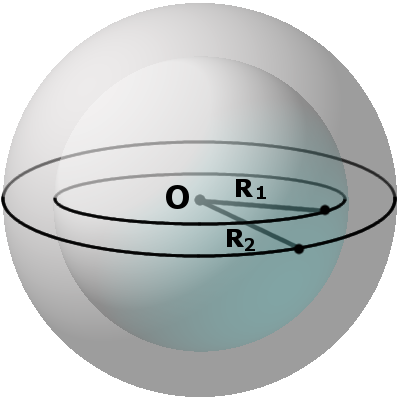
Любое сечение шара или сферы плоскостью есть круг или окружность.

R – радиус сферы или шара;

Формула объёма сферы:

Формула площади сферы (поверхности шара):

S=4πR²

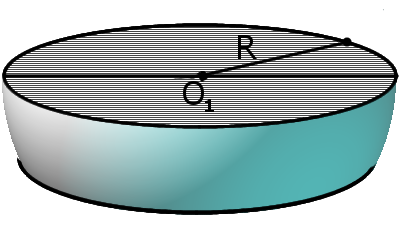
Полусфера

Формула объёма полусферы:

Формула площади полусферы:

S= 2πRh = π(r²+h²)

S= 2πR²

Расчет площади полусферы онлайн.

См. также
Шаровой сегмент, шаровой сектор и шаровой слой

7795

Источник

Сфера, шар, сегмент и сектор. Формулы и свойства сферы

Определение.

Сфера (поверхность шара) — это совокупность всех точек в трехмерном пространстве, которые находятся на одинаковом расстоянии от одной точки, называемой центром сферы (О).

Сферу можно описать, как объёмную фигуру, которая образуется вращением окружности вокруг своего диаметра на 180° или полуокружности вокруг своего диаметра на 360°.

Определение.

Шар — это совокупность всех точек в трехмерном пространстве, расстояние от которых не превышает определенного расстояния до точки, называемой центром шара (О) (совокупность всех точек трехмерного пространства ограниченных сферой).

Шар можно описать как объёмную фигуру, которая образуется вращением круга вокруг своего диаметра на 180° или полуокружности вокруг своего диаметра на 360°.

Определение. Радиус сферы (шара) (R) — это расстояние от центра сферы (шара) O к любой точке сферы (поверхности шара).

Определение. Диаметр сферы (шара) (D) — это отрезок, соединяющий две точки сферы (поверхности шара) и проходящий через ее центр.

Формула. Объём шара:

V =	4	πR³ =	1	πD³
3	6

Формула. Площадь поверхности сферы через радиус или диаметр:

S = 4πR² = πD²

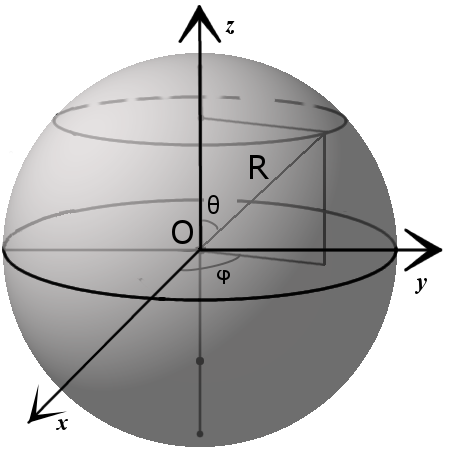
Уравнение сферы

1. Уравнение сферы с радиусом R и центром в начале декартовой системе координат:

x² + y² + z² = R²

2. Уравнение сферы с радиусом R и центром в точке с координатами (x₀, y₀, z₀) в декартовой системе координат:

(x — x₀)² + (y — y₀)² + (z — z₀)² = R²

3. Параметрическое уравнение сферы с центром в точке (x₀, y₀, z₀):

x = x₀ + R · sin θ · cos φ
y = y₀ + R · sin θ · sin φ
z = z₀ + R · cos θ

где θ ϵ [0,π], φ ϵ [0,2π].

Определение. Диаметрально противоположными точками называются любые две точки на поверхности шара (сфере), которые соединены диаметром.

Основные свойства сферы и шара

1. Все точки сферы одинаково удалены от центра.

2. Любое сечение сферы плоскостью является окружностью.

3. Любое сечение шара плоскостью есть кругом.

4. Сфера имеет наибольший объём среди всех пространственных фигур с одинаковой площадью поверхности.

5. Через любые две диаметрально противоположные точки можно провести множество больших окружностей для сферы или кругов для шара.

6. Через любые две точки, кроме диаметрально противоположных точек, можно провести только одну большую окружность для сферы или большой круг для шара.

7. Любые два больших круга одного шара пересекаются по прямой, проходящей через центр шара, а окружности пересекаются в двух диаметрально противоположных точках.

8. Если расстояние между центрами любых двух шаров меньше суммы их радиусов и больше модуля разности их радиусов, то такие шары пересекаются, а в плоскости пересечения образуется круг.

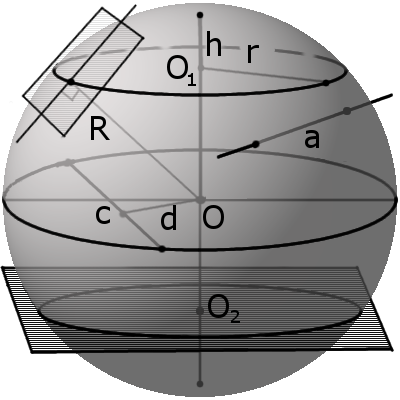
Секущая, хорда, секущая плоскость сферы и их свойства

Определение. Секущая сферы — это прямая, которая пересекает сферу в двух точках. Точки пересечения называются точками протыкания поверхности или точками входа и выхода на поверхности.

Определение. Хорда сферы (шара) — это отрезок, соединяющий две точки сферы (поверхности шара).

Определение. Секущая плоскость — это плоскость, которая пересекает сферу.

Определение. Диаметральная плоскость — это секущая плоскость, проходящая через центр сферы или шара, сечение образует соответственно большую окружность и большой круг. Большая окружность и большой круг имеют центр, который совпадают с центром сферы (шара).

Любая хорда, проходящая через центр сферы (шара) является диаметром.

Хорда является отрезком секущей прямой.

Расстояние d от центра сферы до секущей всегда меньше чем радиус сферы:

d < R

Расстояние m между секущей плоскостью и центром сферы всегда меньше радиуса R:

m < R

Местом сечения секущей плоскости на сфере всегда будет малая окружность, а на шаре местом сечения будет малый круг. Малая окружность и малый круг имеют свои центры, не совпадающих с центром сферы (шара). Радиус r такого круга можно найти по формуле:

r = √R² — m²,

где R — радиус сферы (шара), m — расстояние от центра шара до секущей плоскости.

Определение. Полусфера (полушар) — это половина сферы (шара), которая образуется при ее сечении диаметральной плоскостью.

Касательная, касательная плоскость к сфере и их свойства

Определение.Касательная к сфере — это прямая, которая касается сферы только в одной точке.

Определение.Касательная плоскость к сфере — это плоскость, которая соприкасается со сферой только в одной точке.

Касательная пряма (плоскость) всегда перпендикулярна радиусу сферы проведенному к точке соприкосновения

Расстояние от центра сферы до касательной прямой (плоскости) равно радиусу сферы.

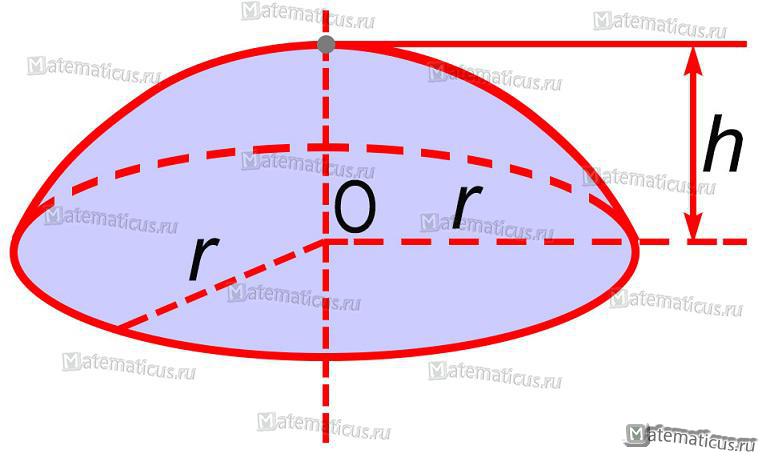
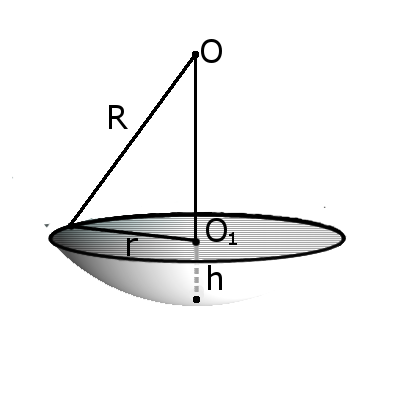
Определение. Сегмент шара — это часть шара, которая отсекается от шара секущей плоскостью. Основой сегмента называют круг, который образовался в месте сечения. Высотой сегмента h называют длину перпендикуляра проведенного с середины основы сегмента к поверхности сегмента.

Формула. Площадь внешней поверхности сегмента сферы с высотой h через радиус сферы R:

S = 2πRh

Формула. Объём сегмента сферы с высотой h через радиус сферы R:

Определение. Срез шара — это часть шара, которая образуется в результате его сечения двумя параллельными плоскостями и находится между ними.

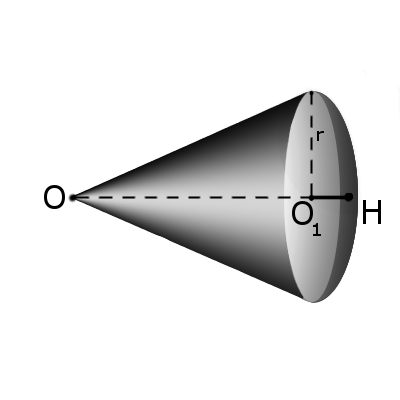
Определение. Сектором называется часть шара, ограниченная совокупностью всех лучей, исходящих из центра шара О и образующих круг на его поверхности с радиусом r.

Формула. Площадь поверхности сектора S с высотой O₁H (h) через радиус шара OH (R):

S = πR(2h + √2hR — h²)

Формула. Объём сектора V с высотой O₁H (h) через радиус шара OH (R):

Определение. Касательными сферами (шарами) называются любые две сферы (шара), которые имеют одну общую точку соприкосновения. Если расстояние между центрами больше суммы радиусов, то фигуры не касаются и не пересекаются.

Определение. Концентрическими сферами называются любые две сферы, которые имеют общий центр и радиусы различной длины.

Источник

Download Article

The radius of a sphere (abbreviated as the variable r or R) is the distance from the exact center of the sphere to a point on the outside edge of that sphere. As with circles, the radius of a sphere is often an essential piece of starting information for calculating the shape’s diameter, circumference, surface area, and/or volume. However, you can also work backward from the diameter, circumference, etc. to find the sphere’s radius. Use the formula that works with the information you have.

1
Find the radius if you know the diameter. The radius is half the diameter, so use the formula r = D/2. This is identical to the method used for calculating the radius of a circle from its diameter.^[1]
- If you have a sphere with a diameter of 16 cm, find the radius by dividing 16/2 to get 8 cm. If the diameter is 42, then the radius is 21.
2
Find the radius if you know the circumference. Use the formula C/2π. Since the circumference is equal to πD, which is equal to 2πr, dividing the circumference by 2π will give the radius.^[2]
- If you have a sphere with a circumference of 20 m, find the radius by dividing 20/2π = 3.183 m.
- Use the same formula to convert between the radius and circumference of a circle.
Advertisement
3
Calculate the radius if you know the volume of a sphere. Use the formula ((V/π)(3/4))^1/3.^[3] The volume of a sphere is derived from the equation V = (4/3)πr³. Solving for the r variable in this equation gets ((V/π)(3/4))^1/3 = r, meaning that the radius of a sphere is equal to the volume divided by π, times 3/4, all taken to the 1/3 power (or the cube root.)^[4]
- If you have a sphere with a volume of 100 inches³, solve for the radius as follows:
  - ((V/π)(3/4))^1/3 = r
  - ((100/π)(3/4))^1/3 = r
  - ((31.83)(3/4))^1/3 = r
  - (23.87)^1/3 = r
  - 2.88 in = r
4
Find the radius from the surface area. Use the formula r = √(A/(4π)). The surface area of a sphere is derived from the equation A = 4πr². Solving for the r variable yields √(A/(4π)) = r, meaning that the radius of a sphere is equal to the square root of the surface area divided by 4π. You can also take (A/(4π)) to the 1/2 power for the same result.^[5]
- If you have a sphere with a surface area of 1,200 cm², solve for the radius as follows:
  - √(A/(4π)) = r
  - √(1200/(4π)) = r
  - √(300/(π)) = r
  - √(95.49) = r
  - 9.77 cm = r

1
Identify the basic measurements of a sphere. The radius (r) is the distance from the exact center of the sphere to any point on the surface of the sphere. Generally speaking, you can find the radius of a sphere if you know the diameter, the circumference, the volume, or the surface area.
- Diameter (D): the distance across the sphere – double the radius. Diameter is the length of a line through the center of the sphere: from one point on the outside of the sphere to a corresponding point directly across from it. In other words, the greatest possible distance between two points on the sphere.
- Circumference (C): the one-dimensional distance around the sphere at its widest point. In other words, the perimeter of a spherical cross-section whose plane passes through the center of the sphere.
- Volume (V): the three-dimensional space contained inside the sphere. It is the «space that the sphere takes up.»^[6]
- Surface Area (A): the two-dimensional area on the outside surface of the sphere. The amount of flat space that covers the outside of the sphere.
- Pi (π): a constant that expresses the ratio of the circle’s circumference to the circle’s diameter. The first ten digits of Pi are always 3.141592653, although it is usually rounded to 3.14.
2
Use various measurements to find the radius. You can use the diameter, circumference, volume, and surface area to calculate the radius of a sphere. You can also calculate each of these numbers if you know the length of the radius itself. Thus, to find the radius, try reversing the formulas for these components’ calculations. Learn the formulas that use the radius to find diameter, circumference, volume, and surface area.^[7]
- D = 2r. As with circles, the diameter of a sphere is twice the radius.
- C = πD or 2πr. As with circles, the circumference of a sphere is equal to π times the diameter. Since the diameter is twice the radius, we can also say that the circumference is twice the radius times π.
- V = (4/3)πr³. The volume of a sphere is the radius cubed (times itself twice), times π, times 4/3.
- A = 4πr². The surface area of a sphere is the radius squared (times itself), times π, times 4. Since the area of a circle is πr², it can also be said that the surface area of a sphere is four times the area of the circle formed by its circumference.

1
Find the (x,y,z) coordinates of the central point of the sphere. One way to think of the radius of a sphere is as the distance between the point at the center of the sphere and any point on the surface of the sphere. Because this is true, if you know the coordinates of the point at the center of the sphere and of any point on the surface, you can find the radius of the sphere simply by calculating the distance between the two points with a variant of the basic distance formula. To begin, find the coordinates of the sphere’s center point. Note that because spheres are three-dimensional, this will be an (x,y,z) point rather than an (x,y) point.
- This process is easier to understand by following along with an example. For our purposes, let’s say that we have a sphere centered around the (x,y,z) point (4, -1, 12). In the next few steps, we’ll use this point to help find the radius.
2
Find the coordinates of a point on the surface of the sphere. Next, you’ll need to find the (x,y,z) coordinates of a point on the surface of the sphere. This can be any point on the surface of the sphere. Because the points on the surface of a sphere are equidistant from the center point by definition, any point will work for determining the radius.
- For our example problem, let’s say that we know that the point (3, 3, 0) lies on the surface of the sphere. By calculating the distance between this point and the center point, we can find the radius.
3
Find the radius with the formula d = √((x₂ — x₁)² + (y₂ — y₁)² + (z₂ — z₁)²).^[8] Now that you know the center of the sphere and a point on the surface, calculating the distance between the two will find the radius. Use the three-dimensional distance formula d = √((x₂ — x₁)² + (y₂ — y₁)² + (z₂ — z₁)²), where d equals distance, (x₁,y₁,z₁) equals the coordinates of the center point, and (x₂,y₂,z₂) equals the coordinates of the point on the surface to find the distance between the two points.
- In our example, we would plug in (4, -1, 12) for (x₁,y₁,z₁) and (3, 3, 0) for (x₂,y₂,z₂), solving as follows:
  - d = √((x₂ — x₁)² + (y₂ — y₁)² + (z₂ — z₁)²)
  - d = √((3 — 4)² + (3 — -1)² + (0 — 12)²)
  - d = √((-1)² + (4)² + (-12)²)
  - d = √(1 + 16 + 144)
  - d = √(161)
  - d = 12.69. This is the radius of our sphere.
4
Know that, in general cases, r = √((x₂ — x₁)² + (y₂ — y₁)² + (z₂ — z₁)²).^[9] In a sphere, every point on the surface of the sphere is the same distance from the center point. If we take the three-dimensional distance formula above and replace the «d» variable with the «r» variable for radius, we get a form of the equation that can can find the radius given any center point (x₁,y₁,z₁) and any corresponding surface point (x₂,y₂,z₂).
- By squaring both sides of this equation, we get r² = (x₂ — x₁)² + (y₂ — y₁)² + (z₂ — z₁)². Note that this is essentially equal to the basic sphere equation r² = x² + y² + z² which assumes a center point of (0,0,0).

Add New Question

Question

How do I find the radius of a sphere if I know its volume is three times its surface area?

Write an equation whereby the volume [(4πr³) / 3] is set equal to three times the surface area (4πr²). Thus, [(4πr³) / 3] = 12πr². Divide both sides by 4π, so that r³/3 = r². Multiply by 3: r³ = 3r². Divide by r²: r = 3. In other words, a sphere’s volume can be three times its surface area only if its radius is 3 units.
Question

How do I calculate the radius of a sphere in my hand by using a ruler?

You can get a very close approximation by carefully measuring the circumference and dividing by twice-pi (6.28).
Question

Two solid spheres A & B are made of the same material. The radius of B is 3 times the radius of A, and the surface area of A is 20 cubic cm. How do I calculate the surface area of B?

The surface area (S) of a sphere equals 4πr², where r is the radius. Using that equation to solve for r: r = √(S / 4π). Now substitute 20 for S, and solve for the radius of sphere A: r = √(20 / 4π) = √(20 / 12.56) = √ 1.59 = 1.26 cm. That’s the radius of sphere A. The radius of sphere B is three times the radius of sphere A: (3)(1.26) = 3.79 cm. So for sphere B, the surface area is 4πr² = (4)(3.14)(3.79)² = 180.4 square centimeters. (That answer makes sense, because when you multiply the radius of a sphere by 3, you multiply its surface area by 3² or 9.) (We didn’t exactly triple the original surface area, because we rounded off some numbers along the way.)

See more answers

Ask a Question

200 characters left

Include your email address to get a message when this question is answered.

Submit

Video

This article was published on demand. However, if you are trying to get to grips with solid geometry for the first time, it’s arguably better to start the other end: calculating the properties of the sphere from the radius.
The order in which the operations are performed matters. If you are uncertain how priorities work, and your calculating device supports parentheses, then make sure to use them.
π or pi is a Greek letter that represents the ratio of the diameter of a circle to its circumference. It’s an irrational number and cannot be written as a ratio of 2 integers. Many approximations exist, 333/106 gives pi to four decimal places. Today most people memorize the approximation 3.14 which is usually sufficiently accurate for everyday purposes.

Show More Tips

Thanks for submitting a tip for review!

About This Article

Article SummaryX

If you know the diameter, you can find the radius of a sphere by dividing the diameter in half. If you know the circumference, you can find the radius by dividing the circumference by 2 times pi. To learn how to calculate the radius of a sphere using two points on the sphere, keep reading!

Did this summary help you?

Thanks to all authors for creating a page that has been read 522,598 times.

Did this article help you?

Источник

Радиус полусферы с учетом отношения поверхности к объему Решение

ШАГ 0: Сводка предварительного расчета

Используемая формула

Радиус полушария = 9/(2*Отношение поверхности к объему полушария)
r = 9/(2*R_A/V)
В этой формуле используются 2 Переменные

Используемые переменные

Радиус полушария — (Измеряется в метр) — Радиус полушария — это расстояние между центром и любой точкой на окружности полушария.
Отношение поверхности к объему полушария — (Измеряется в 1 на метр) — Отношение поверхности к объему полушария — это численное отношение общей площади поверхности полушария к объему полушария.

ШАГ 1. Преобразование входов в базовый блок

Отношение поверхности к объему полушария: 0.9 1 на метр —> 0.9 1 на метр Конверсия не требуется

ШАГ 2: Оцените формулу

Подстановка входных значений в формулу

r = 9/(2*R_A/V) —> 9/(2*0.9)

Оценка … …

r = 5

ШАГ 3: Преобразуйте результат в единицу вывода

5 метр —> Конверсия не требуется

ОКОНЧАТЕЛЬНЫЙ ОТВЕТ

5 метр <— Радиус полушария

(Расчет завершен через 00.003 секунд)

Источник

Перед тем, как смело броситься на амбразуру решения задачи по нахождению радиуса сферы, нужно узнать, что вообще такое сфера и шар. Стереометрия говорит нам, что сфера – это поверхность, состоящая из массы точек пространства, которые находятся на одном расстоянии от центра. Эта точка – центр сферы, а радиус сферы (R) – это расстояние, на которое каждая точка удалена от центра сферы. Шар – это тело, которое ограничено поверхностью сферы.

Безусловно, способ определения того самого радиуса сферы будет зависеть от данных, которые у нас есть.

Способ 1. Определение радиуса сферы при помощи площади ее поверхности

Допустим, нам дана сфера вместе с площадью её поверхности. В таком случае мы будем использовать формулу площади её поверхности для того, чтобы вычислить радиус.