Download Article
Download Article
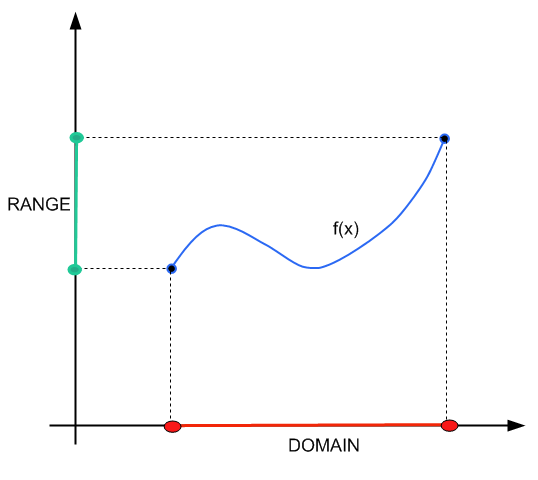
The range of a function is the set of numbers that the function can produce. In other words, it is the set of y-values that you get when you plug all of the possible x-values into the function. This set of possible x-values is called the domain. If you want to know how to find the range of a function, just follow these steps.
-
1
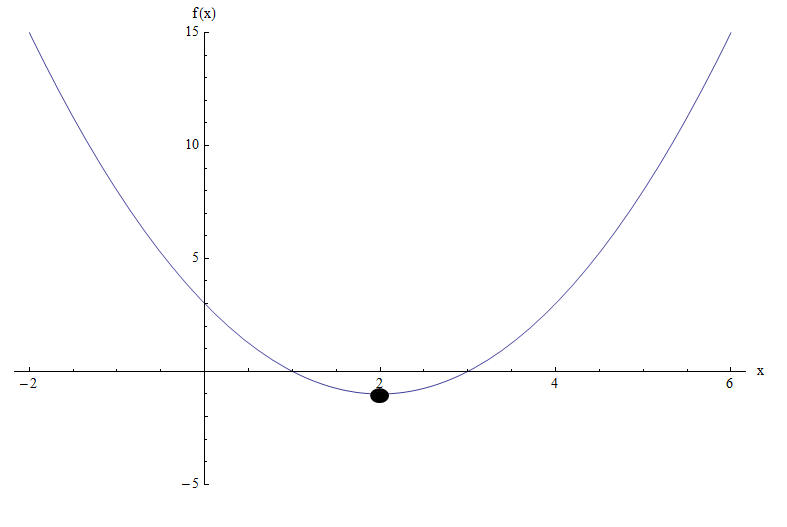
Write down the formula. Let’s say the formula you’re working with is the following: f(x) = 3x2 + 6x -2. This means that when you place any x into the equation, you’ll get your y value. This is the function of a parabola.
-
2
Find the vertex of the function if it’s quadratic. If you’re working with a straight line or any function with a polynomial of an odd number, such as f(x) = 6x3+2x + 7, you can skip this step. But if you’re working with a parabola, or any equation where the x-coordinate is squared or raised to an even power, you’ll need to plot the vertex. To do this, just use the formula -b/2a to get the x coordinate of the function 3x2 + 6x -2, where 3 = a, 6 = b, and -2 = c. In this case -b is -6, and 2a is 6, so the x-coordinate is -6/6, or -1.
- Now, plug -1 into the function to get the y-coordinate. f(-1) = 3(-1)2 + 6(-1) -2 = 3 — 6 -2 = -5.
- The vertex is (-1,-5). Graph it by drawing a point where the x coordinate is -1 and where the y-coordinate is -5. It should be in the third quadrant of the graph.
Advertisement
-
3
Find a few other points in the function. To get a sense of the function, you should plug in a few other x-coordinates so you can get a sense of what the function looks like before you start to look for the range. Since it’s a parabola and the x2 coordinate is positive, it’ll be pointing upward. But just to cover your bases, let’s plug in some x-coordinates to see what y coordinates they yield:
- f(-2) = 3(-2)2 + 6(-2) -2 = -2. One point on the graph is (-2, -2)
- f(0) = 3(0)2 + 6(0) -2 = -2. Another point on the graph is (0,-2)
- f(1) = 3(1)2 + 6(1) -2 = 7. A third point on the graph is (1, 7).
-
4
Find the range on the graph. Now, look at the y-coordinates on the graph and find the lowest point at which the graph touches a y-coordinate. In this case, the lowest y-coordinate is at the vertex, -5, and the graph extends infinitely above this point. This means that the range of the function is y = all real numbers ≥ -5.
Advertisement
-
1
Find the minimum of the function. Look for the lowest y-coordinate of the function.[1]
Let’s say the function reaches its lowest point at -3. This function could also get smaller and smaller infinitely, so that it doesn’t have a set lowest point — just infinity. -
2
Find the maximum of the function. Let’s say the highest y-coordinate that the function reaches is 10.[2]
This function could also get larger and larger infinitely, so it doesn’t have a set highest point — just infinity. -
3
State the range. This means that the range of the function, or the range of y-coordinates, ranges from -3 to 10. So, -3 ≤ f(x) ≤ 10. That’s the range of the function.
- But let’s say the graph reaches its lowest point at y = -3, but goes upward forever. Then the range is f(x) ≥ -3 and that’s it.
- Let’s say the graph reaches its highest point at 10 but goes downward forever. Then the range is f(x) ≤ 10.
Advertisement
-
1
Write down the relation. A relation is a set of ordered pairs with of x and y coordinates. You can look at a relation and determine its domain and range. Let’s say you’re working with the following relation: {(2, –3), (4, 6), (3, –1), (6, 6), (2, 3)}.[3]
-
2
List the y-coordinates of the relation. To find the range of the relation, simply write down all of the y-coordinates of each ordered pair: {-3, 6, -1, 6, 3}.[4]
-
3
Remove any duplicate coordinates so that you only have one of each y-coordinate. You’ll notice that you have listed «6» two times. Take it out so that you are left with {-3, -1, 6, 3}.[5]
-
4
Write the range of the relation in ascending order. Now, reorder the numbers in the set so that you’re moving from the smallest to the largest, and you have your range. The range of the relation {(2, –3), (4, 6), (3, –1), (6, 6), (2, 3)} is {-3,-1, 3, 6}. You’re all done.[6]
-
5
Make sure that the relation is a function. For a relation to be a function, every time you put in one number of an x coordinate, the y coordinate has to be the same. For example, the relation {(2, 3) (2, 4) (6, 9)} is not a function, because when you put in 2 as an x the first time, you got a 3, but the second time you put in a 2, you got a four. For a relation to be a function, if you put in the same input, you should always get the same output. If you put in a -7, you should get the same y coordinate (whatever it may be) every single time.[7]
Advertisement
-
1
Read the problem. Let’s say you’re working with the following problem: «Becky is selling tickets to her school’s talent show for 5 dollars each. The amount of money she collects is a function of how many tickets she sells. What is the range of the function?»
-
2
Write the problem as a function. In this case, M represents the amount of money she collects, and t represents the amount of tickets she sells. However, since each ticket will cost 5 dollars, you’ll have to multiply the amount of tickets sold by 5 to find the amount of money. Therefore, the function can be written as M(t) = 5t.
- For example, if she sells 2 tickets, you’ll have to multiply 2 by 5 to get 10, the amount of dollars she’ll get.
-
3
Determine the domain. To determine the range, you must first find the domain. The domain is all of the possible values of t that work in the equation. In this case, Becky can sell 0 or more tickets — she can’t sell negative tickets. Since we don’t know the number of seats in her school auditorium, we can assume that she can theoretically sell an infinite number of tickets. And she can only sell whole tickets; she can’t sell 1/2 of a ticket, for example. Therefore, the domain of the function is t = any non-negative integer.
-
4
Determine the range. The range is the possible amount of money that Becky can make from her sale. You have to work with the domain to find the range. If you know that the domain is any non-negative integer and that the formula is M(t) = 5t, then you know that you can plug any non-negative integer into this function to get the output, or the range. For example, if she sells 5 tickets, then M(5) = 5 x 5, or 25 dollars. If she sells 100, then M(100) = 5 x 100, or 500 dollars. Therefore, the range of the function is any non-negative integer that is a multiple of five.
- That means that any non-negative integer that is a multiple of five is a possible output for the input of the function.
Advertisement
Practice Problems and Answers
Our Most Loved Articles & Quizzes
Add New Question
-
Question
What is range on a graph?
David Jia is an Academic Tutor and the Founder of LA Math Tutoring, a private tutoring company based in Los Angeles, California. With over 10 years of teaching experience, David works with students of all ages and grades in various subjects, as well as college admissions counseling and test preparation for the SAT, ACT, ISEE, and more. After attaining a perfect 800 math score and a 690 English score on the SAT, David was awarded the Dickinson Scholarship from the University of Miami, where he graduated with a Bachelor’s degree in Business Administration. Additionally, David has worked as an instructor for online videos for textbook companies such as Larson Texts, Big Ideas Learning, and Big Ideas Math.
Academic Tutor
Expert Answer
The range is all of the possible y values that can exist on a graph. What are all of the numbers that could successfully be plugged in as y coordinates? What are all of the places that the graph vertically touches? Answering these questions helps you find the range of a graph.
-
Question
How can I find range of a function using limits?
If a function doesn’t have a maximum (or a minimum), then you might have to evaluate a limit to find its range. For example, f(x) = 2^x doesn’t have a minimum but the limit as x approaches negative infinity is 0, and the limit as x approaches positive infinity is infinity. So the range is (0,infinity) using open intervals because neither limit is ever reached, only approached.
-
Question
What is AM = GM concept for finding range?
This refers to the Arithmetic Mean (AM) — Geometric Mean (GM) inequality, which states that for positive numbers, the AM is always at least as large as the GM. In some cases, this can be used to find upper or lower bounds for the range of a function.
For example, find the range of f(x) = x^2 + 1/x^2. It obviously has a minimum, but where? Many calculus students will immediately take a derivative. This works fine, but if you know the AM-GM inequality, there is no need for the heavy artillery of calculus.
f(x) = 2 * AM(x^2, 1/x^2). The GM of (x^2, 1/x^2) is 1, and the since the AM is more than that, f(x) is always at least 2, and the range of f is [2, infinity).
See more answers
Ask a Question
200 characters left
Include your email address to get a message when this question is answered.
Submit
Advertisement
Video
-
For more difficult cases, it may be easier to draw the graph first using the domain (if possible) and then determine the range graphically.
-
See if you can find the inverse function. The domain of a function’s inverse function is equal to that function’s range.
-
Check to see if the function repeats. Any function which repeats along the x-axis will have the same range for the entire function. For instance, f(x) = sin(x) has a range between -1 and 1.
Advertisement
About This Article
Article SummaryX
To find the range of a function in math, first write down whatever formula you’re working with. Then, if you’re working with a parabola or any equation where the x-coordinate is squared or raised to an even power, use the formula -b divided by 2a to get the x- and then y-coordinates. You can skip this step if you’re working with a straight line or any function with a polynomial of an odd number. Next, plug in a few other x-coordinates and solve for their y-coordinates. Finally, plot those points on a graph to see the range of your function. For more on finding the range of a function, including for a relation and in a word problem, scroll down!
Did this summary help you?
Thanks to all authors for creating a page that has been read 1,009,271 times.
Did this article help you?
There are different ways to Find the Range of a Function Algebraically. But before that, we take a short overview of the Range of a Function.
In the first chapter What is a Function? we have learned that a function is expressed as
y=f(x),
where x is the input and y is the output.
For every input x (where the function f(x) is defined) there is a unique output.
The set of all outputs of a function is the Range of a Function.
![How to Find the Range of a Function Algebraically [15 Ways] How to Find the Range of a Function Algebraically](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/How-to-Find-the-Range-of-a-Function-Algebraically-2.png?resize=482%2C271&ssl=1)
The Range of a Function is the set of all y values or outputs i.e., the set of all f(x) when it is defined.
We suggest you read this article “9 Ways to Find the Domain of a Function Algebraically” first. This will help you to understand the concepts of finding the Range of a Function better.
In this article, you will learn
- 5 Steps to Find the Range of a Function,
and in the end you will be able to
- Find the Range of 10 different types of functions
Table of Contents — What you will learn
Suppose we have to find the range of the function f(x)=x+2.
We can find the range of a function by using the following steps:
#1. First label the function as y=f(x)
y=x+2
#2. Express x as a function of y
Here x=y-2
#3. Find all possible values of y for which f(y) is defined
See that x=y-2 is defined for all real values of y.
#4. Element values of y by looking at the initial function f(x)
Our initial function y=x+2 is defined for all real values of x i.e., xepsilon mathbb{R}.
So here we do not need to eliminate any value of y i.e., yepsilon mathbb{R}.
#5. Write the Range of the function f(x)
Therefore the Range of the function y=x+2 is {yepsilon mathbb{R}}.
Maybe you are getting confused and don’t understand all the steps now.
But believe me, you will get a clear concept in the next examples.
How to Find the Range of a Function Algebraically
There are different types of functions. Here you will learn 10 ways to find the range for each type of function.
#1. Find the range of a Rational function
Example 1: Find the range
f(x)=frac{x-2}{3-x},xneq3
Solution:
Step 1: First we equate the function with y
y=frac{x-2}{3-x}
Step 2: Then express x as a function of y
y=frac{x-2}{3-x}
or, y(3-x)=x-2
or, 3y-xy=x-2
or, x+xy=3y+2
or, x(1+y)=3y+2
or, x=frac{3y+2}{y+1}
Step 3: Find possible values of y for which x=f(y) is defined
x=frac{3y+2}{y+1} is defined when y+1 can not be equal to 0,
i.e., y+1neq0
i.e., yneq-1
i.e., yepsilon mathbb{R}-{-1}
Step 4: Eliminate the values of y
See that f(x)=frac{x-2}{3-x} is defined on mathbb{R}-{3} and we do not need to eliminate any value of y from yepsilon mathbb{R}-{-1}.
Step 5: Write the Range
therefore the range of f(x)=frac{x-2}{3-x} is {xepsilon mathbb{R}:xneq-1}.
Example 2: Find the range
f(x)=frac{3}{2-x^{2}}
Solution:
Step 1:
y=frac{3}{2-x^{2}}
Step 2:
y=frac{3}{2-x^{2}}
or, 2y-xy^{2}=3
or, 2y-3=x^{2y}
or, x^{2}=frac{2y-3}{y}
Step 3:
The function x^{2}=frac{2y-3}{y} is defined when yneq 0 …(1)
Also since x^{2}geq 0,
therefore
frac{2y-3}{y}geq 0
or, frac{2y-3}{y}times {color{Magenta} frac{y}{y}}geq 0
or, frac{y(2y-3)}{y^{2}}geq 0
or, y(2y-3)geq 0 (because y^{2}geq 0)
or, (y-0){color{Magenta} 2}(y-frac{3}{{color{Magenta} 2}})
or, (y-0)(y-frac{3}{2})geq 0
Next we find the values of y for which (y-0)(y-frac{3}{2})geq 0 i.e., y(2y-3)geq 0 is satisfied.
Now see the table:
| Value of y | Sign of (y-0) | Sign of (2y-3) | Sign of y(2y-3) | y(2y-3)geq 0 satisfied or not |
|---|---|---|---|---|
| y=-1<0 i.e., yepsilon (-infty,0) |
-ve | -ve | +ve i.e., >0 |
✅ |
| y=0 | 0 | -ve | =0 | ✅ |
| y=1 i.e., yepsilon (0,frac{3}{2}) |
+ve | -ve | -ve i.e., <0 |
❌ |
| y=frac{3}{2} | +ve | 0 | =0 | ✅ |
| y=2>frac{3}{2} i.e., yepsilon (frac{3}{2},infty) |
+ve | +ve | +ve i.e., >0 |
✅ |
Therefore from the above table and using (1) we get,
yepsilon (-infty,0)cup [frac{3}{2},infty) (because yneq 0)
Step 4:
y=frac{3}{2-x^{2}} is not a square function,
therefore we do not need to eliminate any value of y except 0 because if y be zero then the function y=frac{3}{2-x^{2}} will be undefined.
Step 5:
Therefore the range of the function f(x)=frac{3}{2-x^{2}} is
(-infty,0)cup [frac{3}{2},infty).
Example 3: Find the range of a rational equation using inverse
f(x)=frac{2x-1}{x+4}
Solution:
#2. Find the range of a function with square root
Example 4: Find the range
f(x)=sqrt{4-x^{2}}
Solution:
Step 1: First we equate the function with y
y=sqrt{4-x^{2}}
Step 2: Then express x as a function of y
y=sqrt{4-x^{2}}
or, y^{2}=4-x^{2}
or, x^{2}=4-y^{2}
Step 3: Find possible values of y for which x=f(y) is defined
Since x^{2}geq 0,
therefore 4-y^{2}geq 0
or, (2-y)(2+y)geq 0
or, (y-2)(y+2)leq 0
Now we find possible values for which (y-2)(y+2)leq 0
| Value of y | Sign of (y-2) | Sign of (y+2) | Sign of (y-2)(y+2) | (y-2)(y+2)leq 0 is satisfied or not |
|---|---|---|---|---|
| y=-3<-2 i.e., yepsilon (-infty,-2) |
-ve | -ve | +ve i.e., >0 |
❌ |
| y=-2 | -ve | 0 | =0 | ✅ |
| y=0 i.e., -2<y<2 i.e., yepsilon (-2,2) |
-ve | +ve | -ve i.e., <0 |
✅ |
| y=2 | 0 | +ve | =0 | ✅ |
| y=3>2 i.e., yepsilon (2,infty) |
+ve | +ve | +ve i.e., >0 |
❌ |
i.e., y=-2, yepsilon (-2,2) and y=2
i.e., yepsilon [-2,2]
Step 4: Eliminate the values of y
As y=sqrt{4-x^{2}}, a square root function,
so y can not take any negative value i.e., ygeq 0
Therefore yepsilon [0,2].
Step 5: Write the range
The range of the function f(x)=sqrt{4-x^{2}} is [0,2] in interval notation.
We can also write the range of the function f(x)=sqrt{4-x^{2}} as R(f)={xepsilon mathbb{R}:0leq y leq 2}
Example 5: Find the range of a function f(x) =sqrt{x^{2}-4}.
Solution:
Step 1: First we equate the function with y
y=sqrt{x^{2}-4}
Step 2: Then express x as a function of y
y=sqrt{x^{2}-4}
or, y^{2}=x^{2}-4
or, x^{2}=y^{2}+4
Step 3: Find possible values of y for which x=f(y) is defined
Since x^{2}geq 0,
therefore y^{2}+4geq 0
i.e., y^{2}geq -4
i.e., ygeq sqrt{-4}
i.e, ygeq isqrt{2}, a complex number
therefore y^{2}+4geq 0 for all yepsilon mathbb{R}
Step 4: Eliminate the values of y
Since y=sqrt{x^{2}-4} is a square root function,
therefore y can not take any negative value i.e., ygeq 0
Step 5: Write the Range
The range of f(x) =sqrt{x^{2}-4} is (0,infty).
Example 6: Find the range for the square root function
f(x)=3-sqrt{x}
Solution:
#3. Find the range of a function with a square root in the denominator
Example 7: Find the range
f(x)=frac{1}{sqrt{x-3}}
Solution:
Step 1:
y=frac{1}{sqrt{x-3}}
Step 2:
y=frac{1}{sqrt{x-3}}
or, y=frac{1}{sqrt{x-3}}
or, y^{2}=frac{1}{x-3}
or, y^{2}(x-3)=1
or, xy^{2}-3y^{2}=1
or, xy^{2}=1+3y^{2}
or, x=frac{1+3y^{2}}{y^{2}}
Step 3:
For x=frac{1+3y^{2}}{y^{2}} to be defined,
y^{2}neq 0
i.e., yneq 0
Step 4:
As f(x)=frac{1}{sqrt{x-3}}, so y can not be negative (-ve).
Step 5:
The range of f(x)=frac{1}{sqrt{x-3}} is (0,infty).
Example 8: Find the range
f(x)=frac{1}{sqrt{4-x^{2}}}
Solution:
Step 1:
y=frac{1}{sqrt{4-x^{2}}}
Step 2:
y=frac{1}{sqrt{4-x^{2}}}
or, y=frac{1}{sqrt{4-x^{2}}}
or, y^{2}=frac{1}{4-x^{2}}
or, 4y^{2}-x^{2}y^{2}=1
or, x^{2}y^{2}=4y^{2}-1
or, x^{2}=frac{4y^{2}-1}{y^{2}}
Step 3:
For x^{2}=frac{4y^{2}-1}{y^{2}} to be defined, y can not be equal to zero
i.e., yneq 0
Also since x^{2}geq 0,
therefore frac{4y^{2}-1}{y^{2}}geq 0
or, 4y^{2}-1geq 0 (because y^{2}geq 0)
or, (2y-1)(2y+1)geq 0
or, 4(y-frac{1}{2})(y+frac{1}{2})geq 0
| Value of y | Sign of (2y-1) | Sign of (2y+1) | Sign of (2y-1)(2y+1) | (2y-1)(2y+1)geq 0 is satisfied or not |
|---|---|---|---|---|
| y=-1<-frac{1}{2} i.e., yepsilon left ( -infty,-frac{1}{2} right ) |
-ve | -ve | +ve i.e., >0 |
✅ |
| y=-frac{1}{2} | -ve | 0 | 0 | ✅ |
| y=0 i.e., yepsilon left (-frac{1}{2},frac{1}{2} right ) |
-ve | +ve | -ve i.e., <0 |
❌ |
| y=frac{1}{2} | 0 | +ve | +ve i.e., >0 |
✅ |
| y=1>frac{1}{2} i.e., yepsilon left (frac{1}{2},infty right ) |
+ve | +ve | +ve i.e., >0 |
✅ |
The above table implies that
yepsilon left ( -infty,-frac{1}{2} right )cup left (frac{1}{2},infty right ) …..(1)
Step 4:
Since y=frac{1}{sqrt{4-x^{2}}} is a square root function,
therefore y can not be negative (-ve).
i.e., ygeq 0 …..(2)
Now from (1) and (2), we get
yepsilon ( frac{1}{2},infty )
Step 5:
Therefore the range of the function f(x)=frac{1}{sqrt{4-x^{2}}} is [ frac{1}{2},infty )
Example 9: Find the range of the function
f(x)=sqrt{frac{(x-3)(x+2)}{x-1}}
Solution:
#4. Find the range of modulus function or absolute value function
Example 10: Find the range of the absolute value function
f(x)=left | x right |
Solution:
We can find the range of the absolute value function f(x)=left | x right | on a graph.
If we draw the graph then we get
![How to Find the Range of a Function Algebraically [15 Ways] Find the range of modulus function or absolute value function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/Find-the-range-of-modulus-function-absolute-value-function-1.png?resize=700%2C375&ssl=1)
Here you can see that the y value starts at y=0 and extended to infinity.
therefore the range of the absolute value function f(x)=left | x right | is [0,infty).
Example 11: Find the range of the absolute value function
f(x)=-left | x-1 right |
Solution:
The graph of f(x)=-left | x-1 right | is
![How to Find the Range of a Function Algebraically [15 Ways] Find the range of modulus function or absolute value function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/Find-the-range-of-modulus-function-absolute-value-function-2.png?resize=700%2C375&ssl=1)
From the graph, it is clear that the y value starts from y=0 and extended to -infty.
Therefore the range of f(x)=-left | x-1 right | is (-infty,0].
Shortcut Trick:
- If the sign before modulus is positive (+ve) i.e., of the form +left | x-a right |, then the range will be [a,infty),
- If the sign before modulus is negative (-ve) i.e., of the form -left | x-a right |, then the range will be (-infty,a].
We can also find the range of the absolute value functions f(x)=left | x right | and f(x)=-left | x-1 right | using the above short cut trick:
The function f(x)=left | x right | can be written as f(x)=+left | x-0 right |
Now using trick 1 we can say, the range of f(x)=left | x right | is [0,infty)
Also using trick 2 we can say, the range of f(x)=-left | x-1 right | is (-infty,0].
Example 12: Find the range of the following absolute value functions
- f(x)=left | x right |+6,
- f(x)=left | x+4 right |
Solution:
#5. Find the range of a Step function
Example 13: Find the range of the step function f(x)=[x],xepsilon mathbb{R}.
Solution:
The step function f(x)=[x],xepsilon mathbb{R} is expressed as
f(x)=0, 0leq x<1
=1, 1leq x<2
=2,2leq x<3
………
=-1,-1leq x<0
=-2,-2leq x<-1
………
You can verify this result from the graph of f(x)=[x],xepsilon mathbb{R}
![How to Find the Range of a Function Algebraically [15 Ways] Find the range of a Step function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/Find-the-range-of-a-Step-function-1.png?resize=700%2C375&ssl=1)
i.e., yepsilon {…,-2,-1,0,1,2,…}
i.e., yepsilon mathbb{Z}, the set of all integers.
therefore the range of the step function f(x)=[x],xepsilon mathbb{R} is mathbb{Z}, the set of all integers.
Example 14: Find the range of the step function f(x)=[x-3],xepsilon mathbb{R}.
Solution:
By using the definition of step function, we can express f(x)=[x-3],xepsilon mathbb{R} as
f(x)=1,3leq x<4
=2,4leq x<5
=3,5leq x<6
………
=0,2leq x<3
=-1,1leq x<2
=-2, 0leq x<1
=-3, -1leq x<0
………
You can verify this result from the graph of f(x)=[x-3],xepsilon mathbb{R}
![How to Find the Range of a Function Algebraically [15 Ways] Find the range of a Step function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/Find-the-range-of-a-Step-function-2.png?resize=700%2C375&ssl=1)
i.e., yepsilon {…,-3,-2,-1,0,1,2,3,…}
i.e., yepsilon mathbb{Z}, the set of all integers.
therefore the range of the step function f(x)=[x-3],xepsilon mathbb{R} is mathbb{Z}, the set of all integers.
Example 15: Find the range of the step function f(x)=left [ frac{1}{4x} right ],xepsilon mathbb{R}.
Solution:
#6. Find the range of an Exponential function
Example 16: Find the range of the exponential function f(x)=2^{x}.
Solution:
The graph of the function f(x)=2^{x} is
![How to Find the Range of a Function Algebraically [15 Ways] Find the range of an Exponential function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/Find-the-range-of-an-Exponential-function-1.png?resize=700%2C375&ssl=1)
Here y=0 is an asymptote of f(x)=2^{x} i.e., the graph is going very close and close to the y=0 straight line but it will never touch y=0.
Also, you can see on the graph that the function is extended to +infty.
So we can say y>0.
therefore the range of the exponential function f(x)=2^{x} is (0,infty).
Example 17: Find the range of the exponential function
f(x)=-3^{x+1}+2.
Solution:
The graph of the exponential function f(x)=-3^{x+1}+2 is
![How to Find the Range of a Function Algebraically [15 Ways] Find the range of an Exponential function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/Find-the-range-of-an-Exponential-function-2.png?resize=700%2C375&ssl=1)
From the graph of f(x)=-3^{x+1}+2 you can see that y=2 is an asymptote of f(x)=-3^{x+1}+2 i.e., on the graph f(x)=-3^{x+1}+2 is going very close and close to y=2 towards -ve x-axis but it will never touch the straight line y=2 and extended to -infty towards +ve x-axis.
i.e., y<2
therefore the range of the exponential function f(x)=-3^{x+1}+2 is (-infty,2).
There is a shortcut trick to find the range of any exponential function. This trick will help you find the range of any exponential function in just 2 seconds.
Shortcut trick:
Let f(x)=atimes b^{x-h}+k be an exponential function.
Then
- If a>0, then R(f)=(k,infty),
- If a<0, then R(f)=(-infty,k).
Now we try to find the range of the exponential functions f(x)=2^{x} and f(x)=-3^{x+1}+2 with the above shortcut trick:
We can write f(x)=2^{x} as f(x)=1times 2^{x}+0, 1>0 and comparing this result with trick 1 we directly say
The range of f(x)=2^{x} is (0,infty).
Also f(x)=-3^{x+1}+2 can be written as f(x)=-1times 3^{x+1}+2, -1<0 and comparing with trick 2 we get
The range of f(x)=-3^{x+1}+2 is (-infty,2).
Example 18: Find the range of the exponential functions given below
f(x)=-2^{x+1}+3
Solution:
#7. Find the range of a Logarithmic function
The range of any logarithmic function is (-infty,infty).
We can verify this fact from the graph.
f(x)=log_{2}x^{3} is a logarithmic function and the graph of this function is
![How to Find the Range of a Function Algebraically [15 Ways] Find the range of a Logarithmic function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/Find-the-range-of-a-Logarithmic-function-1.png?resize=700%2C375&ssl=1)
Here you can see that the y value starts from -infty and extended to +infty,
i.e., the range of f(x)=log_{2}x^{3} is (-infty,infty).
Example 19: Find the range of the logarithmic function
f(x)=log_{2}(x+4)+3
Solution:
#8. Find the range of a function relation of ordered pairs
A relation is the set of ordered pairs i.e., the set of (x,y) where the set of all x values is called the domain and the set of all y values is called the range of the relation.
In the previous chapter, we have learned how to find the domain of a function using relation.
Now we learn how to find the range of a function using relation.
For that we have to remember 2 rules which are given below:
Rules:
- Before finding the range of a function first we check the given relation (i.e., the set of ordered pairs) is a function or not
- Find all the y values and form a set. This set is the range of the relation.
Now see the examples given below to understand this concept:
Example 20: Find the range of the relation
{(1,3), (5,9), (8,23), (12,14)}
Solution:
In the relation {(1,3), (5,9), (8,23), (12,14)}, the set of x coordinates is {1, 5, 8, 12} and the set of y coordinates is {3, 9, 14, 23}.
If we draw the diagram of the given relation it will look like this
![How to Find the Range of a Function Algebraically [15 Ways] How to Find the Range of a Function Relation, How to Find the Range of a Function Ordered Pairs](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/How-to-Find-the-range-of-a-function-relation-of-ordered-pairs-1.png?resize=560%2C315&ssl=1)
Here we can clearly see that each element of the set {1, 5, 8, 12} is related to a unique element of the set {3, 9, 14, 23}.
Therefore the given relation is a Function.
Also, we know that the range of a function relation is the set of y coordinates.
Therefore the range of the relation {(1,3), (5,9), (8,23), (12,14)} is the set {3, 9, 14, 23}.
Example 21: Find the range of the set of ordered pairs
{(5,2), (7,6), (9,4), (9,13), (12,19)}.
Solution:
The diagram of the given relation is
![How to Find the Range of a Function Algebraically [15 Ways] How to Find the Range of a Function Relation, How to Find the Range of a Function Ordered Pairs](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/How-to-Find-the-range-of-a-function-relation-of-ordered-pairs-2.png?resize=560%2C315&ssl=1)
Here we can see that element 9 is related to two different elements and they are 4 and 13 i.e., 9 is not related to a unique element and this goes against the definition of the function.
Therefore the relation {(5,2), (7,6), (9,4), (9,13), (12,19)} is not a Function.
Example 22: Determine the range of the relation described by the table
| x | y |
|---|---|
| -1 | 3 |
| 3 | -2 |
| 3 | 2 |
| 4 | 8 |
| 6 | -1 |
Solution:
#9. Find the range of a Discrete function
A Discrete Function is a collection of some points on the Cartesian plane and the range of a discrete function is the set of y-coordinates of the points.
Example 23: How do you find the range of the discrete function from the graph
![How to Find the Range of a Function Algebraically [15 Ways] How to find the Range of a Discrete Function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/how-to-find-the-range-of-a-discrete-function-1.png?resize=700%2C375&ssl=1)
Solution:
From the graph, we can see that there are five points on the discrete function and they are A (2,2), B (4,4), C (6,6), D (8,8), and E (10,10).
![How to Find the Range of a Function Algebraically [15 Ways] How to find the Range of a Discrete Function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/how-to-find-the-range-of-a-discrete-function-2.png?resize=700%2C375&ssl=1)
The set of the y-coordinates of the points A, B, C, D, and E is {2,4,6, 8, 10}.
therefore the range of the discrete function is {2,4,6,8,10}.
Example 24: Find the range of the discrete function from the graph
![How to Find the Range of a Function Algebraically [15 Ways] How to find the Range of a Discrete Function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/how-to-find-the-range-of-a-discrete-function-3.png?resize=700%2C375&ssl=1)
Solution:
The discrete function is made of the five points A (-3,2), B (-2,4), C (2,3), D (3,1), and E (5,5).
![How to Find the Range of a Function Algebraically [15 Ways] How to find the Range of a Discrete Function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/how-to-find-the-range-of-a-discrete-function-4.png?resize=700%2C375&ssl=1)
The set of the y coordinates of the discrete function is {2,4,3,1,5} = {1,2,3,4,5}.
therefore the range of the discrete function is {1,2,3,4,5}.
#10. Find the range of a trigonometric function
| Trigonometric Function | Expresion | Range |
|---|---|---|
| Sine function | sin x | [-1,1] |
| Cosine function | cos x | [-1,1] |
| Tangent function | tan x | (-infty,+infty) |
| CSC function (Cosecant function) |
csc x | (-infty,-1]cup[1,+infty) |
| Secant function | sec x | (-infty,-1]cup[1,+infty) |
| Cotangent function | sec x | (-infty,+infty) |
#11. Find the range of an inverse trigonometric function
| Inverse trigonometric function | Expression | Range |
|---|---|---|
| Arc Sine function / Inverse Sine function |
arcsin x or, sin^{-1}x |
[-frac{pi}{2},+frac{pi}{2}] |
| Arc Cosine function / Inverse Cosine function |
arccos x or, cos^{-1}x |
[0,pi] |
| Arc Tangent function / Inverse Tangent function |
arctan x or, tan^{-1}x |
(-frac{pi}{2},+frac{pi}{2}) |
| Arc CSC function / Inverse CSC function |
textrm{arccsc}x or, csc^{-1}x |
[-frac{pi}{2},0)cup(0,frac{pi}{2}] |
| Arc Secant function / Inverse Secant function |
textrm{arcsec}x or, sec^{-1}x |
[0,frac{pi}{2})cup(frac{pi}{2},pi] |
| Arc Cotangent function / Inverse Cotangent function |
textrm{arccot}x or, cot^{-1}x |
(0,pi) |
#12. Find the range of a hyperbolic function
| Hyperbolic function | Expression | Range |
|---|---|---|
| Hyperbolic Sine function | sinh x=frac{e^{x}-e^{-x}}{2} | (-infty,+infty) |
| Hyperbolic Cosine function | cosh x=frac{e^{x}+e^{-x}}{2} | [1,infty) |
| Hyperbolic Tangent function | tanh x=frac{e^{x}-e^{-x}}{e^{x}+e^{-x}} | (-1,+1) |
| Hyperbolic CSC function | csch x=frac{2}{e^{x}-e^{-x}} | (-infty,0)cup(0,infty) |
| Hyperbolic Secant function | sech x=frac{2}{e^{x}+e^{-x}} | (0,1) |
| Hyperbolic Cotangent function | tanh x=frac{e^{x}+e^{-x}}{e^{x}-e^{-x}} | (-infty,-1)cup(1,infty) |
#13. Find the range of an inverse hyperbolic function
| Inverse hyperbolic function | Expression | Range |
|---|---|---|
| Inverse hyperbolic sine function | sinh^{-1}x=ln(x+sqrt{x^{2}+1}) | (-infty,infty) |
| Inverse hyperbolic cosine function | cosh^{-1}x=ln(x+sqrt{x^{2}-1}) | [0,infty) |
| Inverse hyperbolic tangent function | tanh^{-1}x=frac{1}{2}lnleft (frac{1+x}{1-x}right ) | (-infty,infty) |
| Inverse hyperbolic CSC function | csch^{-1}x=ln left ( frac{1+sqrt{1+x^{2}}}{x} right ) | (-infty,0)cup(0,infty) |
| Inverse hyperbolic Secant function | sech^{-1}x=ln left ( frac{1+sqrt{1-x^{2}}}{x} right ) | [0,infty) |
| Inverse hyperbolic Cotangent function | coth^{-1}x=frac{1}{2}lnleft (frac{x+1}{x-1}right ) | (-infty,0)cup(0,infty) |
#14. Find the range of a piecewise function
Example 25: Find the range of the piecewise function
![How to Find the Range of a Function Algebraically [15 Ways] Piecewise function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/piecewise-function-1.png?resize=373%2C105&ssl=1)
Solution:
The piecewise function consists of two function:
- f(x)=x-3 when xleq -1,
- f(x)=x+1 when x>1.
If we plot these two functions on the graph then we get,
![How to Find the Range of a Function Algebraically [15 Ways] Find the range of a piecewise function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/find-the-range-of-a-piecewise-function-1.png?resize=700%2C375&ssl=1)
This is the graph of the piecewise function.
From the graph, we can see that
- the range of the function f(x)=x-3 is (-infty,-2] when xleq -1,
- the range of the function f(x)=x+1 is (2,infty) when x>1,
Therefore from the above results we can say that
The range of the piecewise function f(x) is
(-infty,-2]cup (2,infty).
Example 26: Find the range of a piecewise function given below
![How to Find the Range of a Function Algebraically [15 Ways] Piecewise function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/piecewise-function-2.png?resize=431%2C149&ssl=1)
Solution:
If you notice the piecewise function then you can see there are functions:
- f(x)=x defined when xleq -1,
- f(x)=2 defined when -1<x<1),
- f(x)=sqrt{x} defined when xgeq 1.
Now if we draw the graph of these three functions we get,
![How to Find the Range of a Function Algebraically [15 Ways] Find the range of a piecewise function](https://i0.wp.com/mathculus.com/wp-content/uploads/2020/10/find-the-range-of-a-piecewise-function-2.png?resize=700%2C375&ssl=1)
This is the graph of the piecewise function.
Here you can see that
The function f(x)=x starts y=-1 and extended to -infty when xleq -1.
So the range of the function f(x)=x,xleq -1 is (-infty,-1]……..(1)
The functional value of the function f(x)=2, -1<x<1 is 2.
The range of the function f(x)=x is {2}……..(2)
The function f(x)=sqrt{x} starts at y=1 and extended to infty when xgeq 1.
The range of the function f(x)=sqrt{x} is [1,infty) when xgeq 1……..(3)
From (1), (2), and (3), we get,
the range of the piecewise function is
(-infty,-1]cup {2}cup [1,infty)
= (-infty,-1]cup [1,infty)
#15. Find the range of a composite function
Example 27: Let f(x)=2x-6 and g(x)=sqrt{x} be two functions.
Find the range of the following composite functions:
(a) fcirc g(x)
(b) gcirc f(x)
Solution of (a)
First we need to find the function gcirc f(x).
We know that,
fcirc g(x)
=f(g(x))
=f(sqrt{x}) (because g(x)=sqrt{x})
=2sqrt{x}-6
Now see that 2sqrt{x}-6 is a function with a square root and at the beginning of this article, we already learned how to find the range of a function with a square root.
Following these steps, we can get,
the range of the composite function f of g is
R(fcirc g)=[-6,infty).
Solution of (b):
gcirc f(x)
=g(f(x))
=g(2x-6) (because f(x)=2x-6)
=sqrt{2x-6}, a function with a square root
Using the previous method we get,
the range of the composite function gcirc f(x) is
R(gcirc f(x))=[0,infty)
Example 28: Let f(x)=3x-12 and g(x)=sqrt{x} be two functions.
Find the range of the following composite functions
- fcirc g(x),
- gcirc f(x)
Solution:
Anil Kumar (Duration: 3 minutes 35 seconds)
Also read:
- How to Find the Domain of a Function Algebraically – Best 9 Ways
- 3 ways to find the zeros of a function
- How to find the zeros of a quadratic function?
- 13 ways to find the limit of a function
- How to use the Squeeze theorem to find a limit?
Изучение того, как найти диапазон функции, может оказаться очень важным в алгебре и исчислении, потому что это дает вам возможность оценить, какие значения достигаются функцией. Или, другими словами, позволяет найти набор всех изображений с помощью функции
Задача поиска точек, которые могут быть достигнуты функцией, очень полезна. Например, у вас может быть производственная функция (q(x)), которая дает вам объем вывода, полученный для (x) единиц ввода.
Мы хотели бы знать, сколько единиц ввода необходимо для производства (b) единиц вывода. Другими словами, нам нужно найти (x), чтобы (q(x) = b), что является еще одним способом узнать, находится ли (b) в диапазоне функции (q(x)).
В этом уроке мы больше сконцентрируемся на механике нахождения диапазона. Для более концептуального подхода к домену и диапазону вы можете
проверьте этот учебник
.
Алгебраический способ определения диапазона функции
Так же, как когда мы узнали, как вычислить домен, не существует единого рецепта для нахождения диапазона, это действительно зависит от структуры функции (f(x)).
Тем не менее, всегда будет использоваться один алгебраический прием. ТАКИМ способом вы найдете диапазон. Обращать внимание:
Скажем, нам нужно получить диапазон заданной функции (f(x)). Затем мы рассмотрим типичное действительное число (y) и попытаемся решить для (x) следующее уравнение:
[f(x) = y]
Нам нужно определить, для каких значений (y) приведенное выше уравнение может быть решено для (x). Вот и все. Конечно, это может быть сложно сделать, в зависимости от структуры функции (f(x)), но это то, что вам нужно сделать.
Итак, это алгебраический способ, способ найти диапазон функции без построения графиков.
ПРИМЕР 1
Найдите диапазон функции (displaystyle f(x) = frac{x+1}{x-3}):
ОТВЕЧАТЬ:
Мы продолжаем использовать алгебраический способ: пусть (y) будет числом, и мы решим для (x) в следующем уравнении: (f(x) = y). Значение (y) находится в диапазоне, если (f(x) = y) может быть решено для (x).
В этом случае мы имеем:
[large f(x) = y Leftrightarrow frac{x+1}{x-3} = y]
[Rightarrow ,,,x+1 = yleft( x-3 right)]
[Rightarrow ,,,x+1 = yx-3y]
[Rightarrow ,,,x-yx=-1-3y]
[Rightarrow ,,,xleft( 1-y right)=-1-3y]
[Rightarrow ,,,x=frac{3y+1}{y-1}]
Следовательно, когда будет четко определено (x)? Почти для всех (y), кроме случая (y = 1), потому что в этом случае у нас есть деление на (0). Следовательно, диапазон (f) в этом случае — это вся вещественная линия, кроме 1.
Если мы используем обозначение интервалов, мы можем написать (Range(f) = (-infty, 1) cup (1, +infty)).
ПРИМЕР 2
Найдите диапазон функции (f(x) = x^2 — 4x + 3):
ОТВЕЧАТЬ:
Опять же, мы продолжаем использовать алгебраический способ, поэтому вы знаете, что упражнение: Пусть (y) будет числом, и мы решим для (x) в следующем уравнении: (f(x) = y). Значение (y) находится в диапазоне, если (f(x) = y) может быть решено для (x).
В этом случае мы имеем:
[large f(x) = y Leftrightarrow x^2 — 4x + 3 = y]
[Rightarrow ,,, x^2 — 4x + 3 — y = 0 text{ (This is a quadratic equation in x)}]
[Rightarrow displaystyle ,,, x = frac{-b pm sqrt{b^2 — 4ac}}{2a}]
[Rightarrow displaystyle ,,, x = frac{-(-4) pm sqrt{(-4)^2 — 4(1)(3-y)}}{2(1)}]
[Rightarrow displaystyle ,,, x = frac{4 pm sqrt{16 — 4(3-y)}}{2}]
[Rightarrow displaystyle ,,, x = frac{4 pm sqrt{16 — 12 + 4y}}{2}]
[Rightarrow displaystyle ,,, x = frac{4 pm sqrt{4 + 4y}}{2}]
[Rightarrow displaystyle ,,, x = 2 pm sqrt{1+y}]
Теперь, видя это последнее выражение, когда будет хорошо определено (x)? Нам нужно, чтобы аргумент квадратного корня был неотрицательным, поэтому нам нужно:
[1+y ge 0]
что означает, что (y ge -1). Если мы используем обозначение интервалов, мы можем написать (Range(f) = [-1, +infty)).
В этом примере мы могли бы решить эту проблему, используя тот факт, что (f(x) = x^2 — 4x + 3) является квадратичной функцией, а ее график представляет собой параболу, которая открывается вверх.
Точка минимума этой параболы достигается в вершине. Координата x вершины:
[displaystyle x_V = frac{-b}{2a} =frac{-(-4)}{2(1)} =frac{4}{2} = 2]
Теперь координата y вершины просто определяется путем вставки значения (x_V = 2) в квадратичную функцию:
[y_V = f(x_V) = 2^2 — 4(2) + 3 = -1]
Поскольку минимальное значение, достигаемое параболой, равно (-1), мы заключаем, что диапазон равен ([-1, +infty)), что является тем же выводом, что и найденный алгебраически.
График функции (f(x) = x^2 — 4x + 3) делает это еще более понятным:
Мы можем видеть, что на основе графика минимум достигается в (x = 2), что в точности соответствует координате x вершины.
Риск использования графика для определения диапазона заключается в том, что вы потенциально можете неправильно прочитать критические точки на графике и дать неточную оценку того, где функция достигает своего максимума или минимума.
Другие стратегии поиска диапазона функции
Как мы видели в предыдущем примере, иногда мы можем найти диапазон функции, просто взглянув на ее график.
Например, вы хотите найти диапазон функции (f(x) = x + 3). График показан ниже:
На графике выше не показаны минимальные или максимальные точки. Более того, когда (x) большое и положительное значение, значение функции также большое и положительное. И аналогично, когда (x) очень отрицательно, значение функции также очень отрицательное.
Интуиция подсказывает, что функция может принимать любые отрицательные и положительные значения, выбирая достаточно большие (положительные или отрицательные) значения (x). И тогда можно сделать вывод, что диапазон — это вся реальная линия, которая равна ((-infty, +infty)) с использованием обозначения интервала.
Такой анализ верен с точки зрения результата, но ненадежен с точки зрения аргументации. У «графического метода» поиска диапазона есть та же проблема: он привлекателен с интуитивной точки зрения, но довольно скуден с точки зрения содержания.
Обычно, если возможно, мы должны предпочесть аналитический / алгебраический путь. В этом примере нам нужно найти (x):
[x + 3 = y]
[Rightarrow ,, x = y — 3]
Итак, есть ли какие-либо ограничения на (y) для правильного определения (x)? Вовсе нет, поэтому нет никаких ограничений на (y), и можно сделать вывод, что диапазон — это вся реальная линия.
Вы можете проверить эту статью, если хотите
знать, как найти домен функции
вместо.
Есть много веских алгебраических причин для нахождения диапазона, одна из них состоит в том, что он является частью процессов для
найти обратную функцию
.

Вид на пустую аудиторию колледжа с задней стороны.
Для любого человека, заинтересованного в карьере математика или человека, который использует математику, например, в бизнесе, важно уметь четко объяснять формулы и решения. В случае нахождения диапазона функции, вы можете найти это значение несколькими способами. Умение объяснить эти методы может оказаться полезным по мере развития ваших математических и коммуникативных навыков.
В этой статье мы дадим определение математического диапазона, области и функции, а затем расскажем, как найти диапазон функции с помощью формулы, графика и отношения.
Основные выводы:
-
В математике функция представляет собой определенную связь между независимой переменной (x) и зависимой переменной (y).
-
Диапазон функции относится ко всем возможным значениям y.
-
Формула для нахождения диапазона функции: y = f(x). Отношение является функцией только в том случае, если каждому значению x соответствует только одно значение y.
Что такое функция?
Функция — это прикладной математический термин, используемый для описания взаимосвязи между двумя переменными. В формуле вы можете представить функцию в виде:
y = f(x)
В этой формуле y является функцией x, то есть при изменении значения x изменяется и значение y (или диапазон, или зависимая переменная). Например:
Если x равен 2 в уравнении y = x -1, то значение y равно 1: y = 2-1
Но если x имеет значение 10, то y также изменится — до 9, или y=10-1
Что такое диапазон функции?
Значения переменных меняются, что можно представить в виде набора значений, называемых областью и диапазоном функции:
-
Домен: Область функции — это набор чисел, представляющих все значения, которые может иметь x.
-
Диапазон: Диапазон — это набор чисел, которые представляют все потенциальные значения, которые y может иметь на основе функции.
3 способа найти диапазон функции
Для x в упорядоченной паре (x, y) может соответствовать только одно значение y. Для y, однако, существует больше возможностей. Нахождение диапазона функции означает нахождение всех возможных значений, которые может иметь y в зависимости от x. Вы можете найти диапазон функции тремя способами: формула, график или зависимость.
1. Нахождение диапазона функции с помощью формулы
Формула может представлять, как переменная x взаимодействует с переменной y. Эти формулы могут выглядеть по-разному в зависимости от того, какое взаимодействие имеют значения. Ниже приведены шаги, которые можно использовать для алгебраического нахождения диапазона функции:
1. Запишите формулу
Запись формулы — где y = f(x)- может помочь вам определить некоторые аспекты связи между двумя переменными.
Пример: Если вы продаете журналы по 10 долларов за штуку, то ваш общий объем продаж, f(x), равен количеству проданных журналов, x, умноженному на 10. Итак, формула f(x) = 10(x). Если вы продаете ноль, 2, 4 или 10 журналов, то ваши общие продажи составляют $0, $20, $40 и $100.
2. Найдите другие пары координат
Если применить формулу y = f(x), то она показывает положительную зависимость между x и y для всех журналов продаж. Чтобы перепроверить эту информацию, вы можете нарисовать переменные в виде упорядоченных пар на графике. Полученный график является линейным и имеет тенденцию к росту. Это подтверждает вывод о том, что функция положительна.
3. Напишите диапазон
Зная, что вы не можете продавать отрицательные журналы, вы можете определить, что диапазон функции никогда не бывает меньше нуля. Поскольку вы всегда можете продать больше журналов, вы знаете, что диапазон может постоянно увеличиваться на интервалы в 10 раз. Таким образом, вы можете записать диапазон функции в виде эквивалентности.
В данном примере диапазон f(x) = все кратные 10 ? 0.
2. Нахождение диапазона функции с помощью графика
График может обеспечить визуальное представление формы, которую принимает функция, позволяя увидеть, как координаты y взаимодействуют с координатами x. Вот шаги для нахождения диапазона функции с помощью графика:
1. Нарисуйте функцию на графике
Чтобы найти диапазон функции на графике, отметьте (или постройте) координаты области (x) и диапазона (y) на листе бумаги с помощью маленьких точек. Это поможет вам увидеть форму функции. Вы можете увидеть прямую линию, изогнутую линию в форме u или n или что-то похожее на волны.
При построении графика перемещайтесь влево или вправо по оси x, в зависимости от того, отрицательна или положительна координата x. Затем вы двигаетесь вверх или вниз по оси y, в зависимости от того, положительна или отрицательна координата y.
После завершения работы проследите за формой графика. Например, если вы нарисуете координаты {(2, 1), (3, 2), (4, 3)}, то они образуют прямую линию, которая идет вверх (1, 2, 3).
2. Найдите минимум функции
Как только вы получите функцию в виде графика, вы сможете увидеть важные особенности, например, минимум. Это самая низкая точка, которую функция достигает визуально. Минимум может быть бесконечным, то есть график неограниченно расширяется вниз. Если это так, то нижний конец диапазона может быть представлен символом бесконечности (?).
3. Найдите максимум функции
Максимум — это наивысшая точка, которую функция достигает визуально. Как и минимум, это число может быть бесконечным. Это также может быть конкретное место на графике, которое можно записать в виде упорядоченной пары. Например, если максимум находится при 3 на оси x и 10 на оси y, то его координаты будут (3, 10).
4. Запишите диапазон в виде эквивалентности
Иногда невозможно написать каждую y-координату функции. Здесь вы можете указать диапазон как эквивалентность, используя символ меньше, чем (<), символ больше чем (>), меньше или равно символу (?) или символ больше или равно (?).
Пример: Для диапазона {-1, 1, 2, 3} вы можете использовать утверждение как:
-1 ? f(x) ? 3
Если ваш диапазон функции имеет бесконечную составляющую, например {-?, 10}, вы можете записать эквивалентность как:
f(x) ? 10
3. Нахождение диапазона функции с помощью отношения
Третий способ найти диапазон функции — записать ее в виде отношения. Отношение — это набор упорядоченных пар, представляющих координаты на графике. Вы можете записать пары отношения в форме (x, y). Ниже описаны шаги, которые можно использовать для нахождения диапазона функции, записанной в виде отношения:
1. Напишите отношение
Когда вы видите набор упорядоченных пар (x, y), вам может быть проще работать с отношением после того, как вы запишете пары на бумаге. Запишите весь набор в фигурных скобках. Например:
{(2, 1), (4, 5), (9, 21) (7, 14), (5, 14)}
2. Составьте список y-координат отношения
Вы можете перечислить y-координаты отношения, взяв второе число из каждой пары координат и записав их в фигурных скобках. Это поможет вам легче представить диапазон значений y.
Это также поможет вам сократить объем информации, с которой вы работаете при нахождении диапазона, или y. Используя приведенный выше пример, вы бы записали y-координаты как:
{1, 5, 21, 14, 14}
3. Удалите все дублирующиеся числа
В этом наборе отношений число 14 встречается дважды. Для нахождения диапазона функции второе 14 не имеет значения, поэтому его можно убрать. Вы можете записать новый список y-координат в виде:
{1, 5, 21, 14}
4. Напишите диапазон от наименьшего до наибольшего
Поскольку числа расположены не по порядку, трудно определить диапазон. Вы можете изменить порядок чисел, чтобы облегчить определение диапазона. Упорядоченный от наименьшего к наибольшему, набор y-координат отношения является таковым:
{1, 5, 14, 21}
Как только вы измените порядок чисел, вы получите диапазон функции с помощью отношения. Итак, для набора отношений:
{(2, 1), (4, 5), (9, 21) (7, 14), (5, 14)}
Диапазон после вычитания:
{1, 5, 14, 21}
5. Убедитесь, что отношение является функцией
Проверив, что каждое значение x дает одно и то же число y, вы можете подтвердить, что отношение является функцией. Отношение является функцией, только если каждому значению x соответствует только одно значение y.
Пример: Если вы вводите x как 2 и получаете на выходе 4, но в следующий раз, когда вы вводите x как 2, вы получаете на выходе 7, то это отношение не является функцией. Если вы каждый раз получаете одно и то же число, то отношение является функцией.
Для примера набора отношений {(2, 1), (4, 5), (9, 21) (7, 14), (5, 14)}, значения x 2, 4, 9, 7 и 5 имеют только одно связанное выходное число каждое, и поэтому это функция, а найденный диапазон функции проверяется.
From Wikipedia, the free encyclopedia


In mathematics, the range of a function may refer to either of two closely related concepts:
- The codomain of the function
- The image of the function
Given two sets X and Y, a binary relation f between X and Y is a (total) function (from X to Y) if for every x in X there is exactly one y in Y such that f relates x to y. The sets X and Y are called domain and codomain of f, respectively. The image of f is then the subset of Y consisting of only those elements y of Y such that there is at least one x in X with f(x) = y.
Terminology[edit]
As the term «range» can have different meanings, it is considered a good practice to define it the first time it is used in a textbook or article. Older books, when they use the word «range», tend to use it to mean what is now called the codomain.[1][2] More modern books, if they use the word «range» at all, generally use it to mean what is now called the image.[3] To avoid any confusion, a number of modern books don’t use the word «range» at all.[4]
Elaboration and example[edit]
Given a function
with domain 










As an example of the two different usages, consider the function 






In many cases, the image and the codomain can coincide. For example, consider the function 
See also[edit]
- Bijection, injection and surjection
- Essential range
Notes and References[edit]
- ^ Hungerford 1974, page 3.
- ^ Childs 1990, page 140.
- ^ Dummit and Foote 2004, page 2.
- ^ Rudin 1991, page 99.
- ^ Weisstein, Eric W. «Range». mathworld.wolfram.com. Retrieved 2020-08-28.
- ^ Nykamp, Duane. «Range definition». Math Insight. Retrieved August 28, 2020.
{{cite web}}: CS1 maint: url-status (link)
Bibliography[edit]
- Childs (2009). A Concrete Introduction to Higher Algebra. Undergraduate Texts in Mathematics (3rd ed.). Springer. ISBN 978-0-387-74527-5. OCLC 173498962.
- Dummit, David S.; Foote, Richard M. (2004). Abstract Algebra (3rd ed.). Wiley. ISBN 978-0-471-43334-7. OCLC 52559229.
- Hungerford, Thomas W. (1974). Algebra. Graduate Texts in Mathematics. Vol. 73. Springer. ISBN 0-387-90518-9. OCLC 703268.
- Rudin, Walter (1991). Functional Analysis (2nd ed.). McGraw Hill. ISBN 0-07-054236-8.