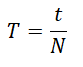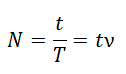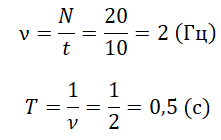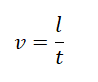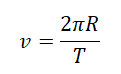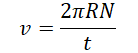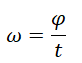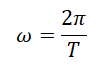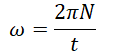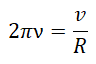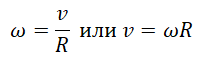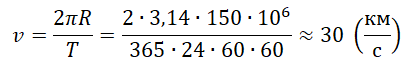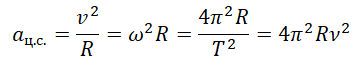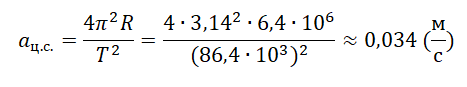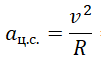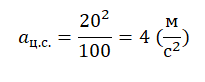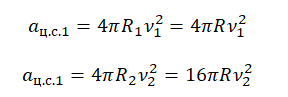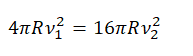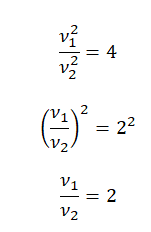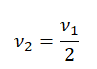I. Механика
Тестирование онлайн
Так как линейная скорость равномерно меняет направление, то движение по окружности нельзя назвать равномерным, оно является равноускоренным.
Угловая скорость
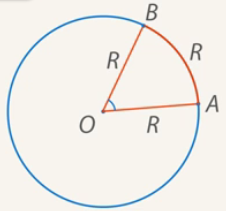
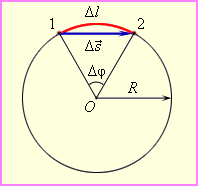
Выберем на окружности точку 1. Построим радиус. За единицу времени точка переместится в пункт 2. При этом радиус описывает угол. Угловая скорость численно равна углу поворота радиуса за единицу времени.
Период и частота
Период вращения T — это время, за которое тело совершает один оборот.
Частота вращение — это количество оборотов за одну секунду.
Частота и период взаимосвязаны соотношением
Связь с угловой скоростью
Линейная скорость
Каждая точка на окружности движется с некоторой скоростью. Эту скорость называют линейной. Направление вектора линейной скорости всегда совпадает с касательной к окружности. Например, искры из-под точильного станка двигаются, повторяя направление мгновенной скорости.
Рассмотрим точку на окружности, которая совершает один оборот, время, которое затрачено — это есть период T. Путь, который преодолевает точка — это есть длина окружности.
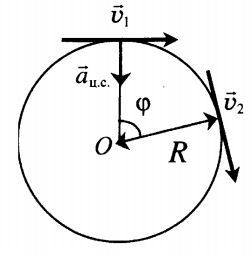
Центростремительное ускорение
При движении по окружности вектор ускорения всегда перпендикулярен вектору скорости, направлен в центр окружности.
Используя предыдущие формулы, можно вывести следующие соотношения
Точки, лежащие на одной прямой исходящей из центра окружности (например, это могут быть точки, которые лежат на спице колеса), будут иметь одинаковые угловые скорости, период и частоту. То есть они будут вращаться одинаково, но с разными линейными скоростями. Чем дальше точка от центра, тем быстрей она будет двигаться.
Закон сложения скоростей справедлив и для вращательного движения. Если движение тела или системы отсчета не является равномерным, то закон применяется для мгновенных скоростей. Например, скорость человека, идущего по краю вращающейся карусели, равна векторной сумме линейной скорости вращения края карусели и скорости движения человека.
Вращение Земли
Связь со вторым законом Ньютона
Как вывести формулу центростремительного ускорения
Движение по циклоиде*
In physics, circular motion is a movement of an object along the circumference of a circle or rotation along a circular path. It can be uniform, with a constant angular rate of rotation and constant speed, or non-uniform with a changing rate of rotation. The rotation around a fixed axis of a three-dimensional body involves the circular motion of its parts. The equations of motion describe the movement of the center of mass of a body. In circular motion, the distance between the body and a fixed point on the surface remains the same.
Examples of circular motion include: an artificial satellite orbiting the Earth at a constant height, a ceiling fan’s blades rotating around a hub, a stone that is tied to a rope and is being swung in circles, a car turning through a curve in a race track, an electron moving perpendicular to a uniform magnetic field, and a gear turning inside a mechanism.
Since the object’s velocity vector is constantly changing direction, the moving object is undergoing acceleration by a centripetal force in the direction of the center of rotation. Without this acceleration, the object would move in a straight line, according to Newton’s laws of motion.
Uniform circular motion[edit]
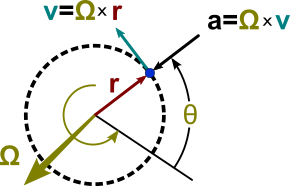
Figure 1: Velocity v and acceleration a in uniform circular motion at angular rate ω; the speed is constant, but the velocity is always tangent to the orbit; the acceleration has constant magnitude, but always points toward the center of rotation.
Figure 2: The velocity vectors at time t and time t + dt are moved from the orbit on the left to new positions where their tails coincide, on the right. Because the velocity is fixed in magnitude at v = r ω, the velocity vectors also sweep out a circular path at angular rate ω. As dt → 0, the acceleration vector a becomes perpendicular to v, which means it points toward the center of the orbit in the circle on the left. Angle ω dt is the very small angle between the two velocities and tends to zero as dt → 0.
Figure 3: (Left) Ball in a circular motion – rope provides centripetal force to keep the ball in a circle (Right) Rope is cut and the ball continues in a straight line with the velocity at the time of cutting the rope, in accord with Newton’s law of inertia, because centripetal force is no longer there.
In physics, uniform circular motion describes the motion of a body traversing a circular path at a constant speed. Since the body describes circular motion, its distance from the axis of rotation remains constant at all times. Though the body’s speed is constant, its velocity is not constant: velocity, a vector quantity, depends on both the body’s speed and its direction of travel. This changing velocity indicates the presence of an acceleration; this centripetal acceleration is of constant magnitude and directed at all times toward the axis of rotation. This acceleration is, in turn, produced by a centripetal force which is also constant in magnitude and directed toward the axis of rotation.
In the case of rotation around a fixed axis of a rigid body that is not negligibly small compared to the radius of the path, each particle of the body describes a uniform circular motion with the same angular velocity, but with velocity and acceleration varying with the position with respect to the axis.
Formulas[edit]
Figure 1: Vector relationships for uniform circular motion; vector ω representing the rotation is normal to the plane of the orbit.
For motion in a circle of radius r, the circumference of the circle is C = 2πr. If the period for one rotation is T, the angular rate of rotation, also known as angular velocity, ω is:

and the units are radians/second.
The speed of the object traveling the circle is:

The angle θ swept out in a time t is:

The angular acceleration, α, of the particle is:

In the case of uniform circular motion, α will be zero.
The acceleration due to change in the direction is:

The centripetal and centrifugal force can also be found using acceleration:

The vector relationships are shown in Figure 1. The axis of rotation is shown as a vector ω perpendicular to the plane of the orbit and with a magnitude ω = dθ / dt. The direction of ω is chosen using the right-hand rule. With this convention for depicting rotation, the velocity is given by a vector cross product as
which is a vector perpendicular to both ω and r(t), tangential to the orbit, and of magnitude ω r. Likewise, the acceleration is given by
which is a vector perpendicular to both ω and v(t) of magnitude ω |v| = ω2 r and directed exactly opposite to r(t).[1]
In the simplest case the speed, mass, and radius are constant.
Consider a body of one kilogram, moving in a circle of radius one metre, with an angular velocity of one radian per second.
- The speed is 1 metre per second.
- The inward acceleration is 1 metre per square second, v2/r.
- It is subject to a centripetal force of 1 kilogram metre per square second, which is 1 newton.
- The momentum of the body is 1 kg·m·s−1.
- The moment of inertia is 1 kg·m2.
- The angular momentum is 1 kg·m2·s−1.
- The kinetic energy is 1 joule.
- The circumference of the orbit is 2π (~6.283) metres.
- The period of the motion is 2π seconds per turn.
- The frequency is (2π)−1 hertz.
In polar coordinates[edit]
During circular motion, the body moves on a curve that can be described in the polar coordinate system as a fixed distance R from the center of the orbit taken as the origin, oriented at an angle θ(t) from some reference direction. See Figure 4. The displacement vector 
where 



The velocity is the time derivative of the displacement:
Because the radius of the circle is constant, the radial component of the velocity is zero. The unit vector 


where the direction of the change must be perpendicular to 






Hence the velocity becomes:
The acceleration of the body can also be broken into radial and tangential components. The acceleration is the time derivative of the velocity:
The time derivative of 






where a negative sign is necessary to keep 



The centripetal acceleration is the radial component, which is directed radially inward:
while the tangential component changes the magnitude of the velocity:
Using complex numbers[edit]
Circular motion can be described using complex numbers. Let the x axis be the real axis and the 

where i is the imaginary unit, and 
Since the radius is constant:
where a dot indicates differentiation in respect of time.
With this notation, the velocity becomes:
and the acceleration becomes:
The first term is opposite in direction to the displacement vector and the second is perpendicular to it, just like the earlier results shown before.
Velocity[edit]
Figure 1 illustrates velocity and acceleration vectors for uniform motion at four different points in the orbit. Because the velocity v is tangent to the circular path, no two velocities point in the same direction. Although the object has a constant speed, its direction is always changing. This change in velocity is caused by an acceleration a, whose magnitude is (like that of the velocity) held constant, but whose direction also is always changing. The acceleration points radially inwards (centripetally) and is perpendicular to the velocity. This acceleration is known as centripetal acceleration.
For a path of radius r, when an angle θ is swept out, the distance traveled on the periphery of the orbit is s = rθ. Therefore, the speed of travel around the orbit is
where the angular rate of rotation is ω. (By rearrangement, ω = v/r.) Thus, v is a constant, and the velocity vector v also rotates with constant magnitude v, at the same angular rate ω.
Relativistic circular motion[edit]
In this case, the three-acceleration vector is perpendicular to the three-velocity vector,
and the square of proper acceleration, expressed as a scalar invariant, the same in all reference frames,
becomes the expression for circular motion,
or, taking the positive square root and using the three-acceleration, we arrive at the proper acceleration for circular motion:
Acceleration[edit]
The left-hand circle in Figure 2 is the orbit showing the velocity vectors at two adjacent times. On the right, these two velocities are moved so their tails coincide. Because speed is constant, the velocity vectors on the right sweep out a circle as time advances. For a swept angle dθ = ω dt the change in v is a vector at right angles to v and of magnitude v dθ, which in turn means that the magnitude of the acceleration is given by
|
|v| r |
1 m/s 3.6 km/h 2.2 mph |
2 m/s 7.2 km/h 4.5 mph |
5 m/s 18 km/h 11 mph |
10 m/s 36 km/h 22 mph |
20 m/s 72 km/h 45 mph |
50 m/s 180 km/h 110 mph |
100 m/s 360 km/h 220 mph |
|
|---|---|---|---|---|---|---|---|---|
| Slow walk | Bicycle | City car | Aerobatics | |||||
| 10 cm 3.9 in |
Laboratory centrifuge |
10 m/s2 1.0 g |
40 m/s2 4.1 g |
250 m/s2 25 g |
1.0 km/s2 100 g |
4.0 km/s2 410 g |
25 km/s2 2500 g |
100 km/s2 10000 g |
| 20 cm 7.9 in |
5.0 m/s2 0.51 g |
20 m/s2 2.0 g |
130 m/s2 13 g |
500 m/s2 51 g |
2.0 km/s2 200 g |
13 km/s2 1300 g |
50 km/s2 5100 g |
|
| 50 cm 1.6 ft |
2.0 m/s2 0.20 g |
8.0 m/s2 0.82 g |
50 m/s2 5.1 g |
200 m/s2 20 g |
800 m/s2 82 g |
5.0 km/s2 510 g |
20 km/s2 2000 g |
|
| 1 m 3.3 ft |
Playground carousel |
1.0 m/s2 0.10 g |
4.0 m/s2 0.41 g |
25 m/s2 2.5 g |
100 m/s2 10 g |
400 m/s2 41 g |
2.5 km/s2 250 g |
10 km/s2 1000 g |
| 2 m 6.6 ft |
500 mm/s2 0.051 g |
2.0 m/s2 0.20 g |
13 m/s2 1.3 g |
50 m/s2 5.1 g |
200 m/s2 20 g |
1.3 km/s2 130 g |
5.0 km/s2 510 g |
|
| 5 m 16 ft |
200 mm/s2 0.020 g |
800 mm/s2 0.082 g |
5.0 m/s2 0.51 g |
20 m/s2 2.0 g |
80 m/s2 8.2 g |
500 m/s2 51 g |
2.0 km/s2 200 g |
|
| 10 m 33 ft |
Roller-coaster vertical loop |
100 mm/s2 0.010 g |
400 mm/s2 0.041 g |
2.5 m/s2 0.25 g |
10 m/s2 1.0 g |
40 m/s2 4.1 g |
250 m/s2 25 g |
1.0 km/s2 100 g |
| 20 m 66 ft |
50 mm/s2 0.0051 g |
200 mm/s2 0.020 g |
1.3 m/s2 0.13 g |
5.0 m/s2 0.51 g |
20 m/s2 2 g |
130 m/s2 13 g |
500 m/s2 51 g |
|
| 50 m 160 ft |
20 mm/s2 0.0020 g |
80 mm/s2 0.0082 g |
500 mm/s2 0.051 g |
2.0 m/s2 0.20 g |
8.0 m/s2 0.82 g |
50 m/s2 5.1 g |
200 m/s2 20 g |
|
| 100 m 330 ft |
Freeway on-ramp |
10 mm/s2 0.0010 g |
40 mm/s2 0.0041 g |
250 mm/s2 0.025 g |
1.0 m/s2 0.10 g |
4.0 m/s2 0.41 g |
25 m/s2 2.5 g |
100 m/s2 10 g |
| 200 m 660 ft |
5.0 mm/s2 0.00051 g |
20 mm/s2 0.0020 g |
130 m/s2 0.013 g |
500 mm/s2 0.051 g |
2.0 m/s2 0.20 g |
13 m/s2 1.3 g |
50 m/s2 5.1 g |
|
| 500 m 1600 ft |
2.0 mm/s2 0.00020 g |
8.0 mm/s2 0.00082 g |
50 mm/s2 0.0051 g |
200 mm/s2 0.020 g |
800 mm/s2 0.082 g |
5.0 m/s2 0.51 g |
20 m/s2 2.0 g |
|
| 1 km 3300 ft |
High-speed railway |
1.0 mm/s2 0.00010 g |
4.0 mm/s2 0.00041 g |
25 mm/s2 0.0025 g |
100 mm/s2 0.010 g |
400 mm/s2 0.041 g |
2.5 m/s2 0.25 g |
10 m/s2 1.0 g |
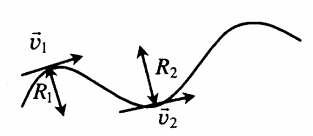
Non-uniform[edit]
In a non-uniform circular motion, an object is moving in a circular path with a varying speed. Since the speed is changing, there is tangential acceleration in addition to normal acceleration.
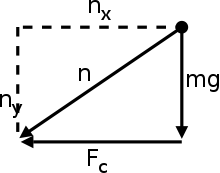
In a non-uniform circular motion, the net acceleration (a) is along the direction of Δv, which is directed inside the circle but does not pass through its center (see figure). The net acceleration may be resolved into two components: tangential acceleration and normal acceleration also known as the centripetal or radial acceleration. Unlike tangential acceleration, centripetal acceleration is present in both uniform and non-uniform circular motion.
In a non-uniform circular motion, normal force does not always point in the opposite direction of weight. Here is an example with an object traveling in a straight path then looping a loop back into a straight path again.
This diagram shows the normal force pointing in other directions rather than opposite to the weight force. The normal force is actually the sum of the radial and tangential forces. The component of weight force is responsible for the tangential force here (We have neglected frictional force). The radial force (centripetal force) is due to the change in the direction of velocity as discussed earlier.
In a non-uniform circular motion, normal force and weight may point in the same direction. Both forces can point down, yet the object will remain in a circular path without falling straight down. First, let’s see why normal force can point down in the first place. In the first diagram, let’s say the object is a person sitting inside a plane, the two forces point down only when it reaches the top of the circle. The reason for this is that the normal force is the sum of the tangential force and centripetal force. The tangential force is zero at the top (as no work is performed when the motion is perpendicular to the direction of force applied. Here weight force is perpendicular to the direction of motion of the object at the top of the circle) and centripetal force points down, thus normal force will point down as well. From a logical standpoint, a person who is travelling in the plane will be upside down at the top of the circle. At that moment, the person’s seat is actually pushing down on the person, which is the normal force.
The reason why the object does not fall down when subjected to only downward forces is a simple one. Think about what keeps an object up after it is thrown. Once an object is thrown into the air, there is only the downward force of Earth’s gravity that acts on the object. That does not mean that once an object is thrown in the air, it will fall instantly. What keeps that object up in the air is its velocity. The first of Newton’s laws of motion states that an object’s inertia keeps it in motion, and since the object in the air has a velocity, it will tend to keep moving in that direction.
A varying angular speed for an object moving in a circular path can also be achieved if the rotating body does not have a homogeneous mass distribution. For inhomogeneous objects, it is necessary to approach the problem as in.[2]
Applications[edit]
Solving applications dealing with non-uniform circular motion involves force analysis. With a uniform circular motion, the only force acting upon an object traveling in a circle is the centripetal force. In a non-uniform circular motion, there are additional forces acting on the object due to a non-zero tangential acceleration. Although there are additional forces acting upon the object, the sum of all the forces acting on the object will have to be equal to the centripetal force.
Radial acceleration is used when calculating the total force. Tangential acceleration is not used in calculating total force because it is not responsible for keeping the object in a circular path. The only acceleration responsible for keeping an object moving in a circle is the radial acceleration. Since the sum of all forces is the centripetal force, drawing centripetal force into a free body diagram is not necessary and usually not recommended.
Using 


In a uniform circular motion, the total acceleration of an object in a circular path is equal to the radial acceleration. Due to the presence of tangential acceleration in a non uniform circular motion, that does not hold true any more. To find the total acceleration of an object in a non uniform circular, find the vector sum of the tangential acceleration and the radial acceleration.
Radial acceleration is still equal to 





See also[edit]
- Angular momentum
- Equations of motion for circular motion
- Time derivative § Example: circular motion
- Fictitious force
- Geostationary orbit
- Geosynchronous orbit
- Pendulum (mathematics)
- Reactive centrifugal force
- Reciprocating motion
- Simple harmonic motion § Uniform circular motion
- Sling (weapon)
References[edit]
- ^ Knudsen, Jens M.; Hjorth, Poul G. (2000). Elements of Newtonian mechanics: including nonlinear dynamics (3 ed.). Springer. p. 96. ISBN 3-540-67652-X.
- ^ Gomez, R W; Hernandez-Gomez, J J; Marquina, V (25 July 2012). «A jumping cylinder on an inclined plane». Eur. J. Phys. IOP. 33 (5): 1359–1365. arXiv:1204.0600. Bibcode:2012EJPh…33.1359G. doi:10.1088/0143-0807/33/5/1359. S2CID 55442794. Retrieved 25 April 2016.
External links[edit]
- Physclips: Mechanics with animations and video clips from the University of New South Wales
- Circular Motion – a chapter from an online textbook
- Circular Motion Lecture – a video lecture on CM
- [1] – an online textbook with different analysis for circular motion
Равномерное движение по окружности.
Автор — профессиональный репетитор, автор учебных пособий для подготовки к ЕГЭ Игорь Вячеславович Яковлев
Темы кодификатора ЕГЭ: движение по окружности с постоянной по модулю скоростью, центростремительное ускорение.
Равномерное движение по окружности — это достаточно простой пример движения с вектором ускорения, зависящим от времени.
Пусть точка вращается по окружности радиуса . Скорость точки постоянна по модулю и равна
. Скорость
называется линейной скоростью точки.
Период обращения — это время одного полного оборота. Для периода имеем очевидную формулу:
. (1)
Частота обращения — это величина, обратная периоду:
.
Частота показывает, сколько полных оборотов точка совершает за секунду. Измеряется частота в об/с (обороты в секунду).
Пусть, например, . Это означает, что за время
точка совершает один полный
оборот. Частота при этом получается равна: об/с; за секунду точка совершает 10 полных оборотов.
Угловая скорость.
Рассмотрим равномерное вращение точки в декартовой системе координат. Поместим начало координат в центре окружности (рис. 1).
 |
| Рис. 1. Равномерное движение по окружности |
Пусть — начальное положение точки; иными словами, при
точка имела координаты
. Пусть за время
точка повернулась на угол
и заняла положение
.
Отношение угла поворота ко времени называется угловой скоростью вращения точки:
. (2)
Угол , как правило, измеряется в радианах, поэтому угловая скорость измеряется в рад/с. За время, равное периоду вращения, точка поворачивается на угол
. Поэтому
. (3)
Сопоставляя формулы (1) и (3), получаем связь линейной и угловой скоростей:
. (4)
Закон движения.
Найдём теперь зависимость координат вращающейся точки от времени. Видим из рис. 1, что
.
Но из формулы (2) имеем: . Следовательно,
. (5)
Формулы (5) являются решением основной задачи механики для равномерного движения точки по окружности.
Центростремительное ускорение.
Теперь нас интересует ускорение вращающейся точки. Его можно найти, дважды продифференцировав соотношения (5):
С учётом формул (5) имеем:
(6)
Полученные формулы (6) можно записать в виде одного векторного равенства:
(7)
где — радиус-вектор вращающейся точки.
Мы видим, что вектор ускорения направлен противоположно радиус-вектору, т. е. к центру окружности (см. рис. 1). Поэтому ускорение точки, равномерно движущейся по окружности, называется центростремительным.
Кроме того, из формулы (7) мы получаем выражение для модуля центростремительного ускорения:
(8)
Выразим угловую скорость из (4)
и подставим в (8). Получим ещё одну формулу для центростремительного ускорения:
.
Благодарим за то, что пользуйтесь нашими публикациями.
Информация на странице «Равномерное движение по окружности.» подготовлена нашими редакторами специально, чтобы помочь вам в освоении предмета и подготовке к ЕГЭ и ОГЭ.
Чтобы успешно сдать необходимые и поступить в ВУЗ или техникум нужно использовать все инструменты: учеба, контрольные, олимпиады, онлайн-лекции, видеоуроки, сборники заданий.
Также вы можете воспользоваться другими материалами из данного раздела.
Публикация обновлена:
08.05.2023
1. Вспоминай формулы по каждой теме
2. Решай новые задачи каждый день
3. Вдумчиво разбирай решения
Движение по окружности
Криволинейное движение — механическое движение, траектория которого — кривые линии с произвольным ускорением и произвольной скоростью.
Угловая скорость — величина, характеризующая скорость вращения материальной точки вокруг центра вращения. [omega=dfracvarphi t=dfrac{2pi}{t}]
Период обращения — это время одного полного оборота.
Частота обращения — величина, обратная периоду. Частота показывает, сколько полных оборотов совершает материальная точка за секунду. [nu=dfrac1T]
Тангенциальное ускорение — компонента ускорения, направленная по касательной к траектории движения.
Нормальное (центростремительное) ускорение — компонента ускорения, характеризующая быстроту изменения направления вектора скорости для траектории с кривизной, направленное к центру кривизны траектории. [a_text{цс}=dfrac{v^2}{r}]
Полное ускорение тела, движущегося по окружности равно векторной сумме тангенциального и нормального ускорений. [overrightarrow a_text{полн}=overrightarrow a_text{тан}+overrightarrow a_text{цс}]
Шарик движется по окружности радиусом (R_1 = 2) м со скоростью ( upsilon_1 = 3) м/с. Во сколько раз изменится его центростремительное ускорение, если радиус его окружности уменьшить в (n = 3) раза, а скорость увеличить в (k = 5) раз?
По определению центростремительное ускорение равно: [a_{text{ц1}} = frac{upsilon_1^2}{R_1} qquad (1)] [a_{text{ц2}} = frac{upsilon_2^2}{R_2} qquad (2)]
По условию задачи: [upsilon_2=kupsilon_1 qquad (3)] [R_2 = frac{R_1}{n} qquad (4)]
Подставляя (3), (4) и (1) в (2) получаем: [a_{text{ц2}} = dfrac{(kupsilon_1)^2}{dfrac{R_1}{n}} = nk^2frac{upsilon_1^2}{R_1} = nk^2a_{text{ц1}}] [frac{a_{text{ц2}}}{a_{text{ц1}}} = nk^2 = 3cdot5^2 = 75]
Ответ: 75
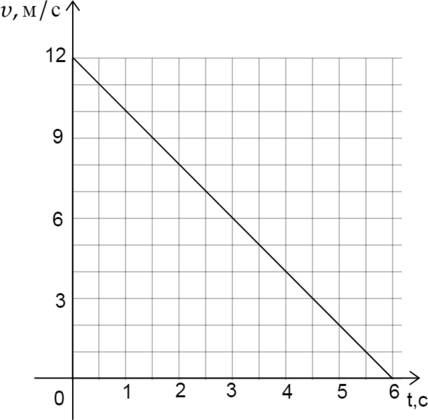
Тело движется по окружности радиусом (R=4) м. В какой момент времени центростремительное ускорение (a_text{цс} = 1 text{ м/с$^2$})?
При движении по окужности центростремительное ускорение можно найти по следующей формуле: [a_text{цс} = frac{upsilon^2}{R}] где (v) – скорость тела
Отсюда: [upsilon = sqrt{a_text{цс} cdot R} = sqrt{1 cdot 4} = 2text{ м/с }] По графику видно, что (upsilon = 2) м/с в момент времени (t=5) с.
Ответ: 5
Верхнюю точку моста радиусом 100 м автомобиль проходит со скоростью 20 м/с. Чему равно центростремительное ускорение автомобиля? (Ответ дайте в метрах в секунду в квадрате.)
Центростремительное ускорение (нормальное): (displaystyle a_{text{цс}}=frac{upsilon^2}R=frac{(20 text{ м/с})^2}{100 text{ м}}=4) м/с(^2)
Ответ: 4
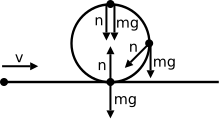
Велосипедист едет по круговому треку и замедляется. На рисунке указано направление скорости велосипедиста. Под каким номером верно указано направление центростремительного ускорения? Тангенциального ускорения? Куда направлено полное ускорение? (В ответе укажите последовательность цифр, например: 153)
Нормальная составляющая ускорения (центростремительное ускорение) характеризует быстроту изменения скорости по направлению. Нормальное ускорение всегда перпендикулярно скорости и направлено к центру по радиусу траектории, по которой движется тело.
Тангенциальная составляющая ускорения характеризует быстроту изменения величины (модуля) скорости. Тангенциальное ускорение всегда коллинеарно скорости:
1) Если тангенциальная составляющая ускорения сонаправлена со скоростью, то движение будет ускоренное
2) Если тангенциальная составляющая ускорения противонаправлена скорости, то движение будет замедленным
Полное ускорение – это сумма нормального и тангенциального: [vec{a}_{text{полн}}=vec{a}_{text{норм}}+vec{a}_{text{танг}}]
Центростремительное ускорение направлено к центру (4). Так как велосепидист замедляется, то тангенциальное направлено против скорости (2). Полное ускорение является суммой 2 и 4, следовательно полное ускорение под номером 3.
Ответ: 423
Тело равномерно движется по окружности. Угловая скорость тела равна (w=6,5) рад/с. За какое время (t) тело совершит 5,5 оборотов? Принять (pi=3,14). Ответ округлить до десятых.
Cпособ 1:
Найдем длину дуги окружности: [l=2pi r,] где (r) — радиус окружности.
Т.к. тело прошло эту длину 5,5 раз, оно прошло путь: [S=5,5l=11pi rquad(1)] Выразим формульно линейную скорость (v) и угловую скорость тела:
[begin{cases}
v=2pi rnu\
w=2pinu
end{cases}
Rightarrow
v=wrquad(2)]
Т.к. тело движется равномерно, (v=const). По закону равномерного движения: [S=vt] Подставим ((1)) и ((2)): [11pi r=wrt] Осталось выразить (t): [t=dfrac{11pi}{w}=dfrac{11cdot3,14}{6,5text{ рад/с}}approx5,3text{ c}]
Cпособ 2 :
Выразим формульно (w): [w=dfrac{Deltavarphi}{t},quad(1)] где (Deltavarphi) — угол поворота тела. [Deltavarphi=dfrac{S}{r},] где (S=5,5l=5,5cdot2pi r=11pi r) — путь, пройденный телом. [Deltavarphi=dfrac{11pi r}{r}=11piquad(2)] Подставим (2) в (1): [w=dfrac{11pi}{t}] Осталось выразить (t): [t=dfrac{11pi}{w}=dfrac{11cdot3,14}{6,5text{ рад/с}}approx5,3text{ c}]
Ответ: 5,3
Две материальные точки движутся по окружностям радиусами (R_1) и (R_2), причем (R_2 = 3R_1). Скорости тел равны. Чему равно отношение их центростремительных ускорений?
По определению центростремительное ускорение равно: [a_{text{ц1}} = frac{upsilon^2}{R_1}] [a_{text{ц2}} = frac{upsilon^2}{R_2}]
Тогда искомое отношение равно: [frac{a_{text{ц1}}}{a_{text{ц2}}} = frac{dfrac{upsilon^2}{R_1}}{dfrac{upsilon^2}{R_2}} = frac{R_2}{R_1} = frac{3R_1}{R_1} = 3]
Ответ: 3
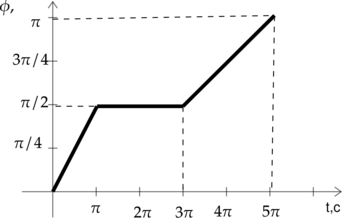
Точечное тело равномерно движется по окужности радиусом (R=2) м. На рисунке изображён график зависимости угла поворота (varphi) от времени (t). Найдите значение линейной скорости тела в интервале времени (3pi<t<5pi).
Линейная скорость тела, движущегося по окружности: [upsilon = omega cdot R qquad (1)] где (omega) – угловая скорость.
Угловая скорость: [omega = frac{Deltavarphi}{Delta t} qquad (2)] Подставив (2) в (1), получим: [upsilon = frac{Deltavarphi}{Delta t} cdot R] [upsilon = frac{dfrac{pi}{2}}{2pi} cdot 2 =0,5 text{ м/с}]
Ответ: 0,5

Как готовиться к сочинению за 2 дня до ЕГЭ? Четко и без воды
Как готовиться к сочинению за 2 дня до ЕГЭ? Четко и без воды
Криволинейное движение — движение, траекторией которого является кривая линия. Вектор скорости тела, движущегося по кривой линии, направлен по касательной к траектории. Любой участок криволинейного движения можно представить в виде движения по дуге окружности или по участку ломаной.
Движение по окружности с постоянной по модулю скоростью — частный и самый простой случай криволинейного движения. Это движение с переменным ускорением, которое называется центростремительным.
Особенности движения по окружности с постоянной по модулю скоростью:
- Траектория движения тела есть окружность.
- Вектор скорости всегда направлен по касательной к окружности.
- Направление скорости постоянно меняется под действием центростремительного ускорения.
- Центростремительное ускорение направлено к центру окружности и не вызывает изменения модуля скорости.
Период, частота и количество оборотов
Пусть тело двигается по окружности беспрерывно. Когда оно сделает один оборот, пройдет некоторое время. Когда тело сделает еще один оборот, пройдет еще столько же времени. Это время не будет меняться, потому что тело движется с постоянной по модулю скоростью. Такое время называют периодом.
Период — время одного полного оборота. Обозначается буквой T. Единица измерения — секунды (с).
t — время, в течение которого тело совершило N оборотов
За один и тот же промежуток времени тело может проходить лишь часть окружности или совершать несколько единиц, десятков, сотен или более оборотов. Все зависит от длины окружности и модуля скорости.
Частота — количество оборотов, совершенных в единицу времени. Обозначается буквой ν («ню»). Единица измерения — Гц.
N — количество оборотов, совершенных телом за время t.
Период и частота — это обратные величины, определяемые формулами:
Количество оборотов выражается следующей формулой:
Пример №1. Шарик на нити вращается по окружности. За 10 секунд он совершил 20 оборотов. Найти период и частоту вращения шарика.
Линейная и угловая скорости
Линейная скорость
Определение и формулы
Линейная скорость — это отношение пройденного пути ко времени, в течение которого этот путь был пройден. Обозначается буквой v. Единица измерения — м/с.
l — длина траектории, вдоль которой двигалось тело за время t
Линейную скорость можно выразить через период. За один период тело делает один оборот, то есть проходить путь, равный длине окружности. Поэтому его скорость равна:
R — радиус окружности, по которой движется тело
Если линейную скорость можно выразить через период, то ее можно выразить и через частоту — величину, обратную периоду. Тогда формула примет вид:
Выразив частоту через количество оборотов и время, в течение которого тело совершало эти обороты, получим:
Угловая скорость
Определение и формулы
Угловая скорость — это отношение угла поворота тела ко времени, в течение которого тело совершало этот поворот. Обозначается буквой ω. Единица измерения — радиан в секунду (рад./с).
ϕ — угол поворота тела. t — время, в течение которого тело повернулось на угол ϕ
Полезные факты
Радиан — угол, соответствующий дуге, длина которой равна ее радиусу. Полный угол равен 2π радиан.
За один полный оборот тело поворачивается на 2π радиан. Поэтому угловую скорость можно выразить через период:
Выражая угловую скорость через частоту, получим:
Выразив частоту через количество оборотов, формула угловой скорости примет вид:
Сравним две формулы:
Преобразуем формулу линейной скорости и получим:
Отсюда получаем взаимосвязь между линейной и угловой скоростями:
Полезные факты
- У вращающихся прижатых друг к другу цилиндров линейные скорости точек их поверхности равны: v1 = v2.
- У вращающихся шестерен линейные скорости точек их поверхности также равны: v1 = v2.
- Все точки вращающегося твердого тела имеют одинаковые периоды, частоты и угловые скорости, но разные линейные скорости. T1 = T2, ν1 = ν2, ω1 = ω2. Но v1 ≠ v2.
Пример №2. Период обращения Земли вокруг Солнца равен одному году. Радиус орбиты Земли равен 150 млн. км. Чему примерно равна скорость движения Земли по орбите? Ответ округлить до целых.
В году 365 суток, в одних сутках 24 часа, в 1 часе 60 минут, в одной минуте 60 секунд. Перемножив все эти числа между собой, получим период в секундах.
За каждую секунду Земля проходит расстояние, равное примерно 30 км.
Центростремительное ускорение
Определение и формула
Центростремительное ускорение — ускорение с постоянным модулем, но меняющимся направлением. Поэтому оно вызывает изменение направления вектора скорости, но не изменяет его модуль. Центростремительное ускорение обозначается как aц.с.. Единица измерения — метры на секунду в квадрате (м/с2). Центростремительное ускорение можно выразить через линейную и угловую скорости, период, частоту и количество оборотов/время:
Пример №3. Рассчитать центростремительное ускорение льва, спящего на экваторе, в системе отсчета, две оси которой лежат в плоскости экватора и направлены на неподвижные звезды, а начало координат совпадает с центром Земли.
Спящий лев сделает один полный оборот тогда, когда Земля сделает один оборот вокруг своей оси. Земля делает это за время, равное 1 сутки. Поэтому период обращения равен 1 суткам. Количество секунд в сутках: 1 сутки = 24•60•60 секунд = 86400 секунд = 86,4∙103 секунд.
Радиус Земли равен 6400 км. В метрах это будет 6,4∙106. Теперь у нас есть все, что нужно для вычисления центростремительного ускорения. Подставляем данные в формулу:
Задание EF18273
Верхнюю точку моста радиусом 100 м автомобиль проходит со скоростью 20 м/с. Центростремительное ускорение автомобиля равно…
Алгоритм решения
- Записать исходные данные.
- Записать формулу для определения искомой величины.
- Подставить известные данные в формулу и произвести вычисления.
Решение
Записываем исходные данные:
- Радиус окружности, по которой движется автомобиль: R = 100 м.
- Скорость автомобиля во время движения по окружности: v = 20 м/с.
Формула, определяющая зависимость центростремительного ускорения от скорости движения тела:
Подставляем известные данные в формулу и вычисляем:
Ответ: 4
pазбирался: Алиса Никитина | обсудить разбор
Задание EF17763
Точка движется по окружности радиусом R с частотой обращения ν. Как нужно изменить частоту обращения, чтобы при увеличении радиуса окружности в 4 раза центростремительное ускорение точки осталось прежним?
а) увеличить в 2 раза
б) уменьшить в 2 раза
в) увеличить в 4 раза
г) уменьшить в 4 раза
Алгоритм решения
- Записать исходные данные.
- Определить, что нужно найти.
- Записать формулу зависимости центростремительного ускорения от частоты.
- Преобразовать формулу зависимости центростремительного ускорения от частоты для каждого из случаев.
- Приравнять правые части формул и найти искомую величину.
Решение
Запишем исходные данные:
- Радиус окружности R1 = R.
- Радиус окружности R2 = 4R.
- Центростремительное ускорение: aц.с. = a1 = a2.
Найти нужно ν2.
Центростремительное ускорение определяется формулой:
Запишем формулы центростремительного ускорения для 1 и 2 случаев соответственно:
Так как центростремительное ускорение в 1 и 2 случае одинаково, приравняем правые части уравнений:
Произведем сокращения и получим:
Или:
Отсюда:
Это значит, чтобы центростремительное ускорение осталось неизменным после увеличения радиуса окружности в 4 раза, частота должна уменьшиться вдвое. Верный ответ: «б».
Ответ: б
pазбирался: Алиса Никитина | обсудить разбор
Алиса Никитина | Просмотров: 22.1k



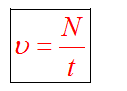

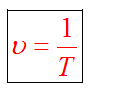























![{displaystyle z=x+iy=Rleft(cos[theta (t)]+isin[theta (t)]right)=Re^{itheta (t)},,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5f70473a1d45582449264bfb173bcd862be3dae7)

![{displaystyle v={dot {z}}={frac {d}{dt}}left(Re^{itheta [t]}right)=R{frac {d}{dt}}left(e^{itheta [t]}right)=Re^{itheta (t)}{frac {d}{dt}}left(itheta [t]right)=iR{dot {theta }}(t)e^{itheta (t)}=iomega Re^{itheta (t)}=iomega z}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bfe1c9630c24006fe24bf7de9194566b0aff4ca9)